Light passes through a closed cylindrical tube containing a gas. If the gas is gradually pumped out, the speed of light inside the tube will
1.increase
2.decrease
3.remain constant
4.first increases then decreases
The correct Answer is:A
To solve the question regarding the speed of light in a closed cylindrical tube containing gas and the effect of gradually pumping out the gas, we can follow these steps:
1. Understanding the Initial Condition:
- Initially, the cylindrical tube contains a gas (e.g., air). The speed of light in any medium is affected by the medium's refractive index. In this case, the gas has a certain refractive index which causes the speed of light to be less than its maximum value.
2. Refractive Index of the Gas:
- The refractive index (n) of a medium is defined as the ratio of the speed of light in vacuum (c) to the speed of light in the medium (v):
n=cv
- For air, the refractive index is slightly greater than 1, which means light travels slower in air than in vacuum.
3. Pumping Out the Gas:
- As the gas is gradually pumped out of the tube, the pressure decreases, and the density of the gas also decreases. Eventually, if enough gas is removed, a vacuum will be created inside the tube.
4. Speed of Light in Vacuum:
- In a vacuum, the refractive index is 1 (n = 1), which means the speed of light reaches its maximum value:
v=c
- Therefore, as the gas is removed and a vacuum is formed, the speed of light will increase to its maximum value.
5. Conclusion:
- Thus, as the gas is gradually pumped out and a vacuum is created, the speed of light inside the tube will increase and ultimately reach the speed of light in vacuum.
Final Answer:
The speed of light inside the tube will increase and reach its maximum value when the gas is completely pumped out, resulting in a vacuum.
---
- Initially, the cylindrical tube contains a gas (e.g., air). The speed of light in any medium is affected by the medium's refractive index. In this case, the gas has a certain refractive index which causes the speed of light to be less than its maximum value.
- The refractive index (n) of a medium is defined as the ratio of the speed of light in vacuum (c) to the speed of light in the medium (v):
n=cv
- For air, the refractive index is slightly greater than 1, which means light travels slower in air than in vacuum.
- As the gas is gradually pumped out of the tube, the pressure decreases, and the density of the gas also decreases. Eventually, if enough gas is removed, a vacuum will be created inside the tube.
- In a vacuum, the refractive index is 1 (n = 1), which means the speed of light reaches its maximum value:
v=c
- Therefore, as the gas is removed and a vacuum is formed, the speed of light will increase to its maximum value.
- Thus, as the gas is gradually pumped out and a vacuum is created, the speed of light inside the tube will increase and ultimately reach the speed of light in vacuum.
The speed of light inside the tube will increase and reach its maximum value when the gas is completely pumped out, resulting in a vacuum.
Topper's Solved these Questions
- SPEED OF LIGHTBOOK - HC VERMA ENGLISHCHAPTER - SPEED OF LIGHTEXERCISE - Objective -2
3 Videos
- SPEED OF LIGHTBOOK - HC VERMA ENGLISHCHAPTER - SPEED OF LIGHTEXERCISE - Exercises
3 Videos
- SEMICONDUCTOR AND SEMICONDUCTOR DEVICESBOOK - HC VERMA ENGLISHCHAPTER - SEMICONDUCTOR AND SEMICONDUCTOR DEVICESEXERCISE - Question for short answer
11 Videos
- THE NUCLEOUSBOOK - HC VERMA ENGLISHCHAPTER - THE NUCLEOUSEXERCISE - Short answer
12 Videos
Similar Questions
Name the gas used in :
light bulbs
View SolutionA beaker containing a liquid is kept inside a big closed jar. If the air inside the jar is continuously pumped out, the pressure in the liquid near the bottom of the liquid will
View SolutionA closed box contains an ideal gas if temperature of gas increased which
View SolutionWhite light is passed through sodium vapours contained in a thin walled glass flask and the transmitted light is examined with the help of a spectrometer. The spectrum so obtained is :
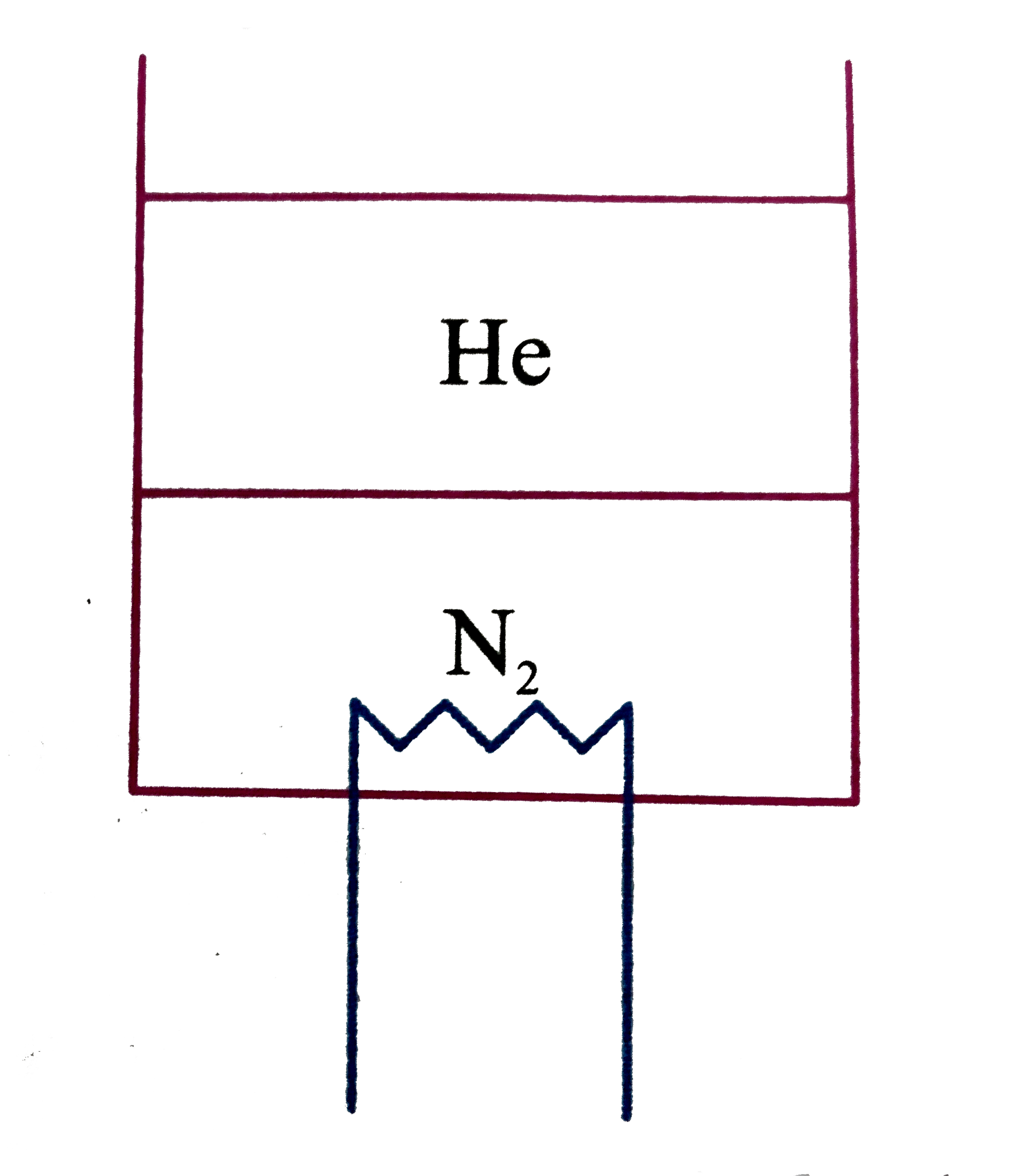
View Solution5 moles of nitrogen gas are enclosed in an adiabatic cylindrical vessel. The piston itself is a rigid light cylindrical container containing 3 moles of helium gas. There is a heater which given out a power of 100 cal of which 30 cal is transferred to helium through the bottom surface of the piston. The rate of increment of temperature of the nitrogen gas assuming that the piston moves slowly is
View SolutionPropyne when passed through a hot iron tube at 400∘C produces
View SolutionA piston is fitted in a cylindrical tube of small cross section with the other end of the tube open. The tube resonates with a tuning fork of frequency 512 Hz. The piston is gradually pulled out of the tube and it is found that a second resonance occurs when the piston is pulled out through a distance of 32.0 cm. Calculate the speed of sound in the air of the tube.
View SolutionWater is flowing through a tube of radius r with a speed v. If this tube is joined to another tube of radius r/2, what is the speed of water in the second tube?
View SolutionA gas at the temperature 250 K is contained in a closed vessel. If the gas is heated through 1K, then the percentage increase in its pressure will be
View SolutionA gas at the temperature 250 K is contained in a closed vessel. If the gas is heated through 1K, then the percentage increase in its pressure will be
View Solution
