Question

Make a diagram to show how hypermetropia is corrected. The near point of a hypermetropic eye is 1 m. What is the power of the lens required to correct this defect? Assume that the near point of the normal eye is 25 cm.

Explanation

The defect of an eye called hypermetropia (long-sightedness or far-sightedness) is corrected by using spectacles containing convex lenses of appropriate focal length.

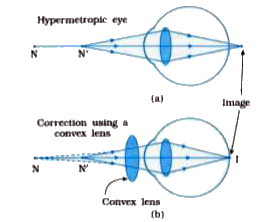
Here the near point of the hypermetropic eye is 1 m = 100 cm
(while near point of normal eye is 25 cm)
This means that this person can see the nearby object (kept at 25 cm) clearly if the image of this nearby object is formed at his own near point (which is lm = 100 cm here).
So, in this case :
Object distance `u=-25 cm` (Normal near point)
Image distance `v = -1 m = - 100 cm` (Near point of this defective eye in front of lens)
Focal length f = ?
Now,
By the lens formula, ` (1)/(f)=(1)/(v) – (1)/(u) `
`therefore (1)/(f)= (1)/(-100) –(1)/(-25) `
`therefore (1)/(f) = - (1)/(100) + (1)/(25) `
` therefore (1)/(f) = (-1+4)/(100) `
` therefore (1)/(f) = (3)/(100) `
` therefore f= (100)/(3) cm`
` = (1)/(3)m `
= 0.3333 m
= 33.33 cm (Convex lens)
Now,
Power of the lens,
` P= (1)/(f) `
`= (1)/(((1)/(3)m)) `
= 3D