Match the Column I with Column II
For a satellite in circular orbit
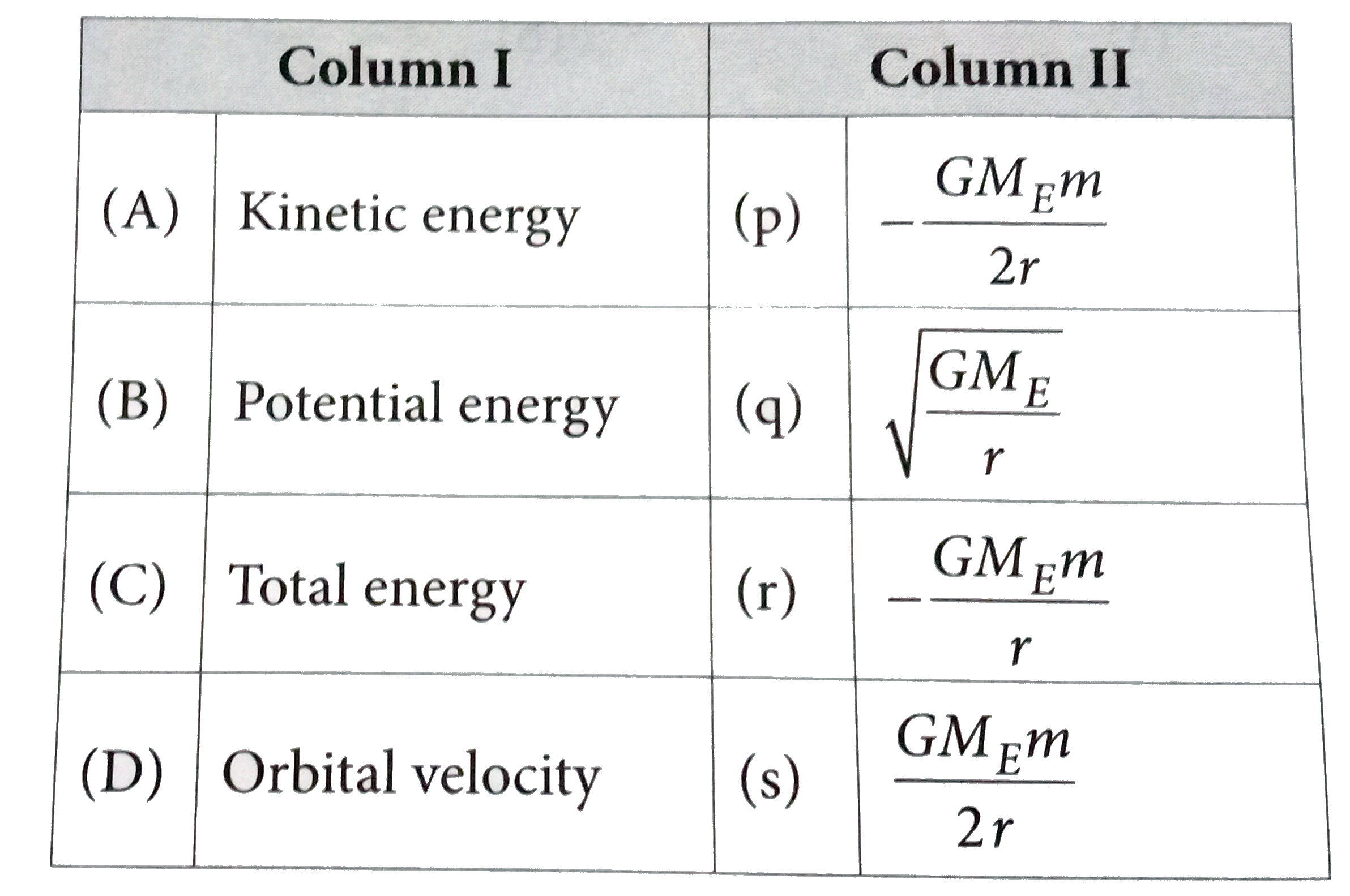
(where ME is the mass of the earth , m is the mass of the satellite and r is the radius of the orbit )
A-r, B-s,C-q,D-p
A-q,B-p,C-r,D-s
A-p,B-q,C-s,D-r
A-s,B-r,C-p,D-q
The correct Answer is:D
(d) Kinetic energy =GMEm2r,A−s
Potential energy =−GMEmr,B−r
Total energy =−GMEm2r,C−p
Orbital velocity =√GMEr,D−q
Topper's Solved these Questions
- GRAVITATIONBOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - Geostationary And Polar Satellites
7 Videos
- GRAVITATIONBOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - Weightlessness
2 Videos
- GRAVITATIONBOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - Earth Satellite
4 Videos
- KINETIC THEORYBOOK - NCERT FINGERTIPSCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason
10 Videos
Similar Questions
The time period of a satellite in a circular orbit of radius R is T. The period of another satellite in a circular orbit of radius 9R is :
View SolutionTwo satellites of masses 50kg and 150kg revolve around the earth in circular orbits of radii 4R and 9Rrespectively where R is the radius of the earth. The speed of the two satellites will be in the ratio ?
View Solution
Knowledge Check
A satellite is revolving in circular orbit of radius r around the earth of mass M. Time of revolution of satellite is
AT∝r5GMBT∝√r3GMCT∝√rGM23DT∝ ⎷r3GM4A satellite moves around the earth in a circular orbit with speed v. If m is the mass of the satellite, its total energy is
A−12mv2B12mv2C32mv2D14mv2The period of a satellite in a circular orbit of radius R is T. The period of another satellite in circular orbit of radius 4 R is :
A4 TBT/4CT/8D8 T
A satellite of mass m0 is revolving around the earth in circular orbit at a height of 3R from surface of the earth the areal velocity of satellite is (where R is radius of earth and M0 is the mass of earth)
View SolutionThe period of a satellite in a circular orbit of radius R is T, the period of another satellite in a circular orbit of radius 4R is
View SolutionAn earth satellite of mass m revolves in a circular orbit at a height h from the surface of the earth. R is the radius of the earth and g is acceleration due to gravity at the surface of the earth. The velocity of the satellite in the orbit is given by
View SolutionThe orbital velocity of an satellite in a circular orbit just above the earth's surface is v. For a satellite orbiting at an altitude of half of the earth's radius, the orbital velocity is
View SolutionThe orbit of geostationary satellite is circular, the time period of satellite depeds on (i) mass of the satellite, (ii) mass of earth, (iii) readius of the orbit and (iv) height of the satellite from the surface of the earth
View Solution
