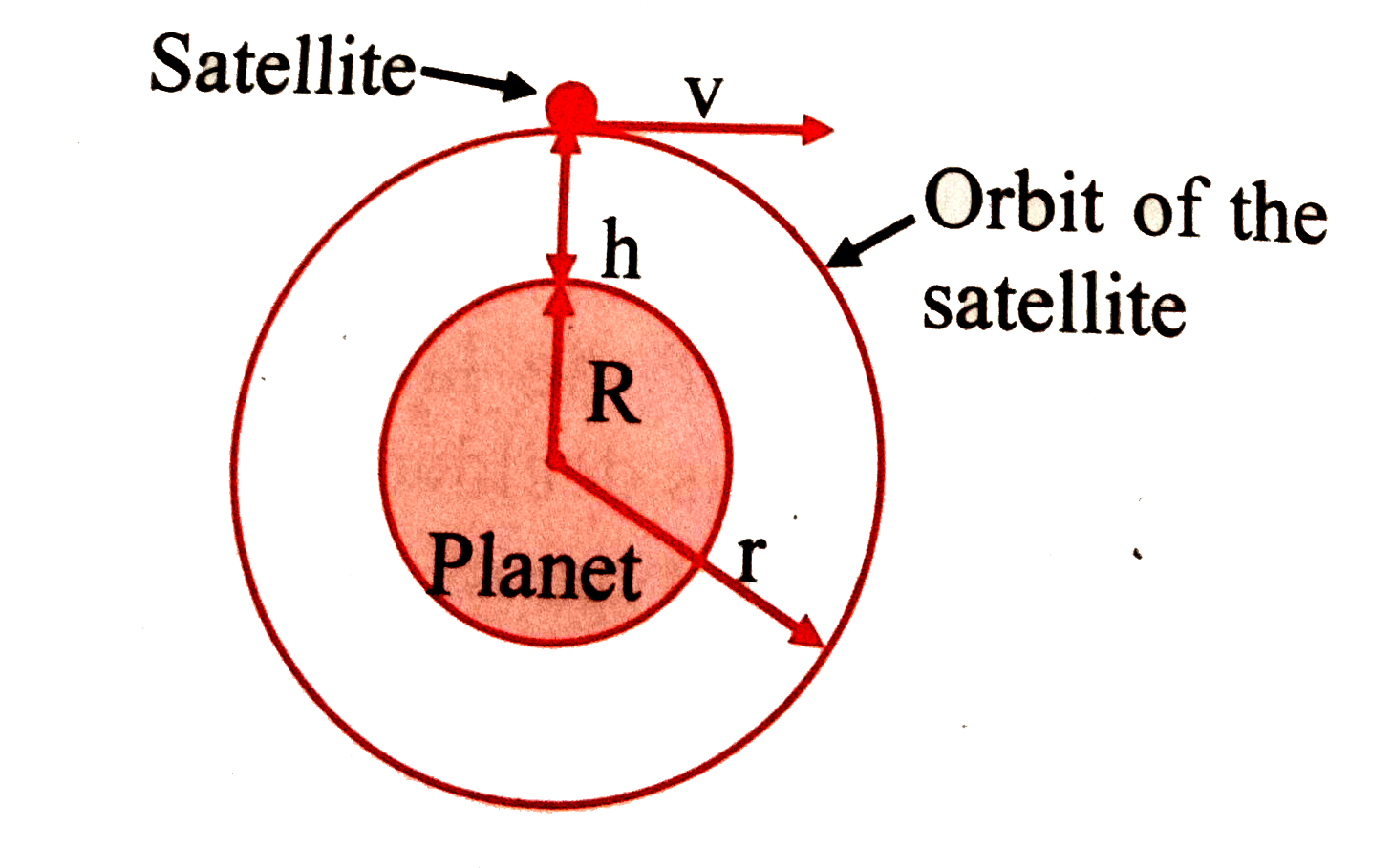
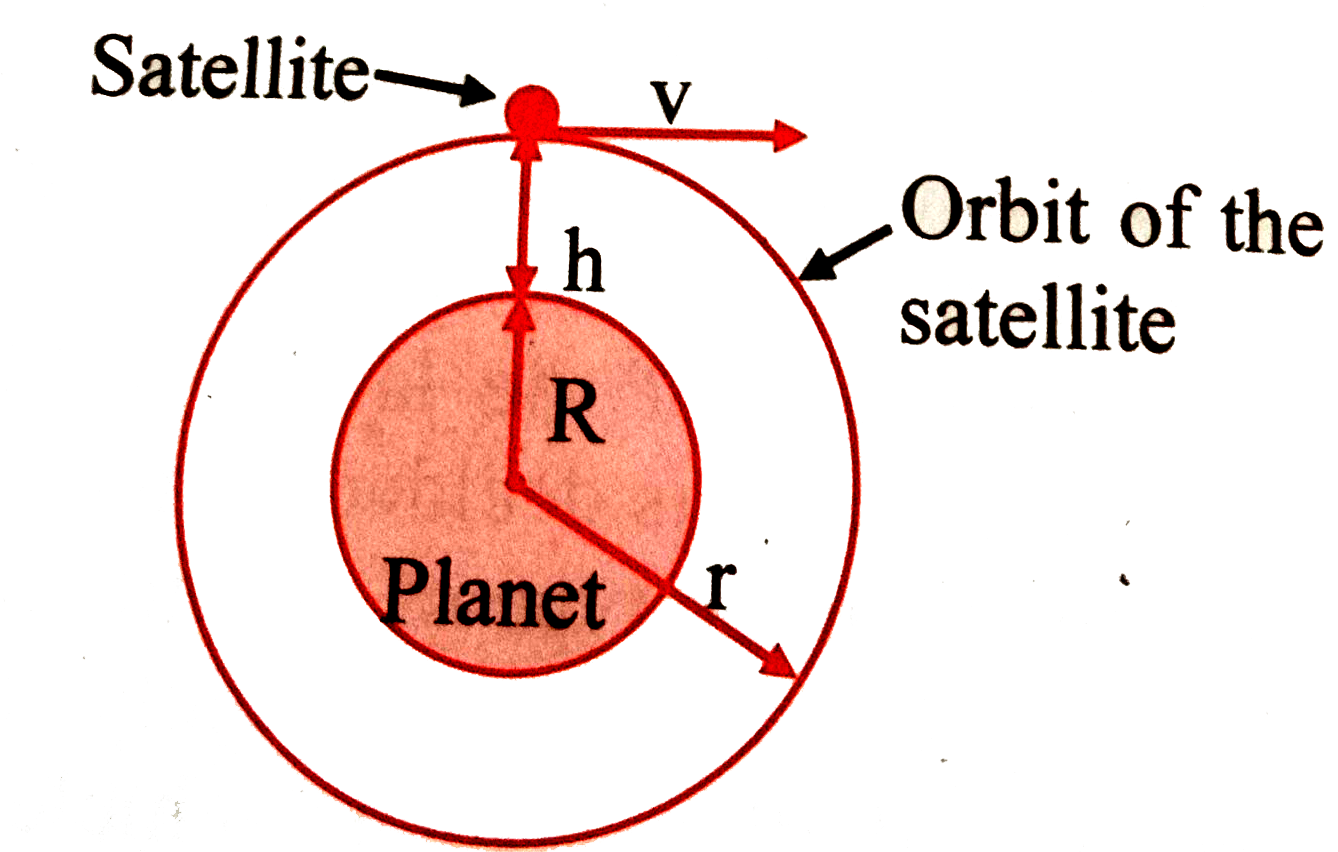
Observe the following diagram and answer the questions:
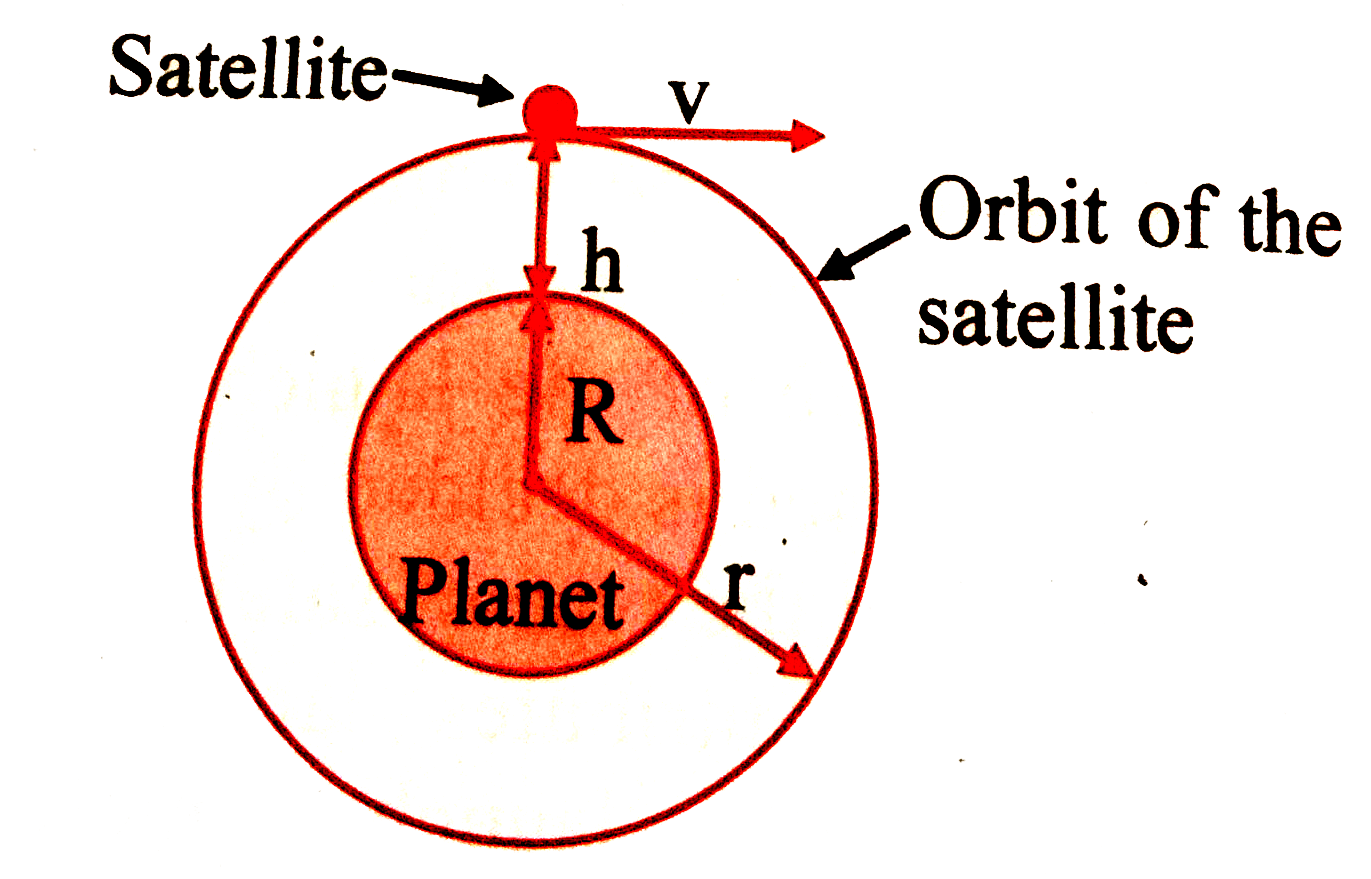
Derive the formula for that velocity of satellite assuming the mass of the planet M and mass of satellite as m .
Critical velocity of a satellite revolving around the planet in circular orbit can be derived as follows: Consider the satellite of mass m revolving at height around the planet of mass M and radius above . As the satellite is moving in circular orbit, centripetal force acting on it is provided by the gravitational force of the planet,
i e . , mv2r=GMmr2
But r =R+h
∴ v2=GM(R+h)
∴ v=√GMR+h
Hence , critical velocity is given by formula
√GMR+h.
Topper's Solved these Questions
- BOOK - TARGET PUBLICATIONCHAPTER - SPACE MISSIONSEXERCISE - Practice Problems4videos
SPACE MISSIONS
- BOOK - TARGET PUBLICATIONCHAPTER - SPACE MISSIONSEXERCISE - Apply your Knowledge2videos
SPACE MISSIONS
- BOOK - TARGET PUBLICATIONCHAPTER - SPACE MISSIONSEXERCISE - Complete the given Chart/table1videos
SPACE MISSIONS
- BOOK - TARGET PUBLICATIONCHAPTER - SOCIAL HEALTH EXERCISE - CHAPTER ASSESSMENT3videos
SOCIAL HEALTH
- BOOK - TARGET PUBLICATIONCHAPTER - TOWARDS GREEN ENERGY EXERCISE - Chapter Assessment2videos
TOWARDS GREEN ENERGY
Similar Questions
Knowledge Check
- Question 1 - Select One
If r represents the radius of the orbit of a satellite of mass m moving around a planet of mass M , the velocity of the satellite is given by
Av2=gMRBv2=GMmRCv=GMrDv2=GMr
Observe the following diagram and answer the questions
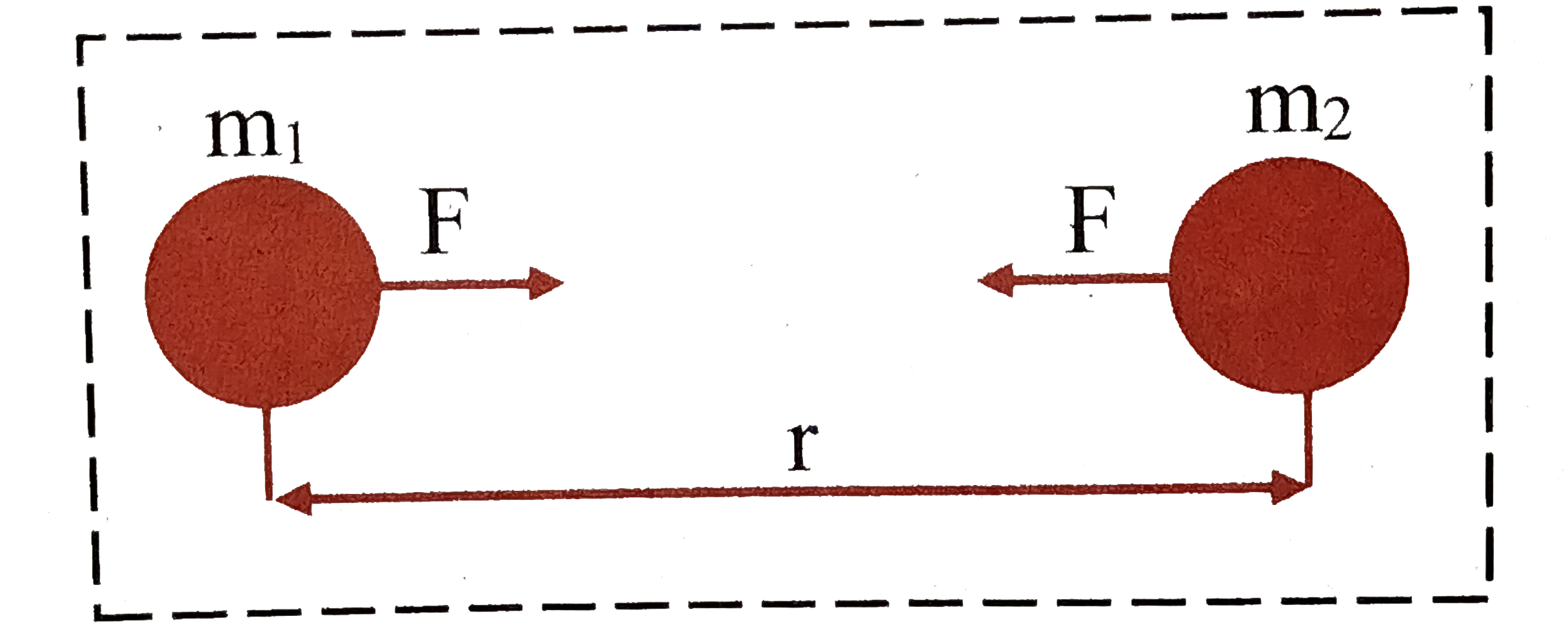
How will the value of F change if the mass m2 is incresed to 4m2 ?View SolutionHow does the orbital velocity of a satellite depend on the mass of the satellite ?
View SolutionOrbital velocity of a satellite depends on the ………..the planet around which the satellite revolves.
View SolutionThe escape velocity of a satellite from the surface of a planet is √2 times the orbital velocity of the satellite. If the ratio of the masses of two given planets is 1 : 4 and that of their radii is 1 : 2, respectively, then find the ratio of escape velocities of a satellite from the surfaces of two planets.
View SolutionDefine orbital velocity. Derive an expression for the orbital velocity of a satellite revolving around a planet
View SolutionWhy the motion of a satellite around a planet cannot be taken as S.H.M ?
View SolutionWhy the motion of a satellite around a planet cannot be taken as S.H.M ?
View Solution