Obtain anexpression for the average power of an a.c circuit .
To derive the expression for the average power in an AC circuit, we will follow these steps:
Step 1: Define the equations for current and voltage
Assume the alternating current (AC) is given by:
I(t)=I0sin(ωt)
And the voltage (or EMF) is given by:
E(t)=E0sin(ωt+ϕ)
where I0 is the peak current, E0 is the peak voltage, ω is the angular frequency, and ϕ is the phase difference between the current and voltage.
Step 2: Calculate the instantaneous power
The instantaneous power P(t) in the circuit is given by the product of voltage and current:
P(t)=I(t)⋅E(t)=I0sin(ωt)⋅E0sin(ωt+ϕ)
Step 3: Use the trigonometric identity
To simplify the expression for P(t), we can use the trigonometric identity:
sinAsinB=12(cos(A−B)−cos(A+B))
Applying this identity:
P(t)=I0E0⋅12(cos(ϕ)−cos(2ωt+ϕ))
Step 4: Find the average power
The average power Pavg over one complete cycle (from 0 to T) can be calculated by integrating the instantaneous power over one period and dividing by the period:
Pavg=1T∫T0P(t)dt
Substituting the expression for P(t):
Pavg=1T∫T0(I0E02(cos(ϕ)−cos(2ωt+ϕ)))dt
Step 5: Evaluate the integrals
The integral of cos(2ωt+ϕ) over one complete cycle is zero, since it oscillates equally above and below the axis. Thus, we can simplify:
Pavg=I0E02cos(ϕ)⋅1T∫T0dt
The integral ∫T0dt=T, so:
Pavg=I0E02cos(ϕ)
Step 6: Express in terms of RMS values
The root mean square (RMS) values of current and voltage are defined as:
Irms=I0√2
Erms=E0√2
Substituting these into the average power expression:
Pavg=IrmsErmscos(ϕ)
Final Expression
Thus, the average power in an AC circuit is given by:
Pavg=IrmsErmscos(ϕ)
Assume the alternating current (AC) is given by:
I(t)=I0sin(ωt)
And the voltage (or EMF) is given by:
E(t)=E0sin(ωt+ϕ)
where I0 is the peak current, E0 is the peak voltage, ω is the angular frequency, and ϕ is the phase difference between the current and voltage.
The instantaneous power P(t) in the circuit is given by the product of voltage and current:
P(t)=I(t)⋅E(t)=I0sin(ωt)⋅E0sin(ωt+ϕ)
To simplify the expression for P(t), we can use the trigonometric identity:
sinAsinB=12(cos(A−B)−cos(A+B))
Applying this identity:
P(t)=I0E0⋅12(cos(ϕ)−cos(2ωt+ϕ))
The average power Pavg over one complete cycle (from 0 to T) can be calculated by integrating the instantaneous power over one period and dividing by the period:
Pavg=1T∫T0P(t)dt
Substituting the expression for P(t):
Pavg=1T∫T0(I0E02(cos(ϕ)−cos(2ωt+ϕ)))dt
The integral of cos(2ωt+ϕ) over one complete cycle is zero, since it oscillates equally above and below the axis. Thus, we can simplify:
Pavg=I0E02cos(ϕ)⋅1T∫T0dt
The integral ∫T0dt=T, so:
Pavg=I0E02cos(ϕ)
The root mean square (RMS) values of current and voltage are defined as:
Irms=I0√2
Erms=E0√2
Substituting these into the average power expression:
Pavg=IrmsErmscos(ϕ)
Thus, the average power in an AC circuit is given by:
Pavg=IrmsErmscos(ϕ)
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - Revision Exercises (Long Answer Questions )
10 Videos
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - Revision Exercises (Nuumerical problems )
18 Videos
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - Revision Exercises (Fill in the Blanks )
10 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
Similar Questions
Knowledge Check
The average power in LCR series circuit is
AermsIrmscosϕBermsIrmssinϕCermsIrmsDermsIrmstanϕAn a.c. emf of e=220 sin 100πt is passed through the resistance of 1 kΩ. The average power of the a. c. circuit is
A48.4 WB34.2 WC24.2 WD12.1 WAn electric bulb consumes only 25% of the peak power in an a.c. circuit. What is the phase difference between the circuit and the applied a.c. voltage ?
Aπ6Bπ2Cπ3Dπ4
Power In An Ac Circuit
View SolutionFind the average power consumed in the circuit.
View SolutionObtain an expresion for average power dissipated in a purely resistive A.C. circuit. Define power factor of the circuit and state its value for purely resistive A.C. circuit.
A rectangular coil of a moving coil galvanometer contains 50 turns each having area 12cm2. It is suspended in radial magnetic field 0.025Wb/m2 by a fibre of twist constant 15×10−10N−m/degree. Calculate the sensitivity of the moving coil galvanometer.
View SolutionIn a series RC circuit with an AC source, R=300Ω,C=25μF,ε050V and v=50(Π)Hz. Find the peak current and the average power dissipated in the circuit.
View SolutionIn the given A.C. circuit the average power consumed in the circuit is
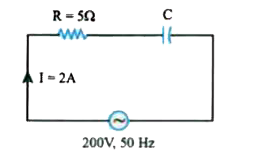
View Solution
