Obtain the expression of electric field by thir spherical shell with uniform charge distribution at a point outside it.Solution in Gujrati
Consider a uniformly charged sphere of radius R having the charge density ρ.
Gauss.s law is ∫→E.→da=qε0
Here we imagine one spherical Gaussian surface of radius r which is greater than R. (r>R)
Total flux passing through the surface = 4πr2E(r)
Total charge enclosed q=4π3R3ρε0
∴E(r)=R3ρ3ρ2ε0(r>R)
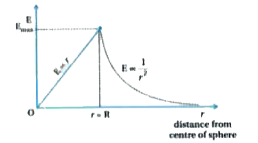
This equation gives the value of the electric field for the points lying in the region outside the sphere at a distance r from the centre of the sphere (r>R)
Total charge of the sphere Q=43πr3ρ
∴ρ=3Q4πR3
Using this in above equation:
∴E(r)=R33r2ε0.3Q4πR3
∴E(r)=Q4πε0.1r2
Thus, for a point outside the sphere the entire charge of the sphere can be treated as concentrated at its centre.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION A QUESTIONS- ANSWERS (TRY YOURSELF )
73 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION B NUMERICALS (NUMERICAL FROM TEXTUAL ILLUSTRATIONS)
24 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION A QUESTIONS- ANSWERS (FIELD DUE TO AN UNIFORMLY CHARGED INFINITE PLANE SHEET)
1 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - KUMAR PRAKASHANCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section-D (MCQs asked in GUJCET/Board Exam)
1 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section D MCQs (MCQs asked in Competitive Exams )
38 Videos
Similar Questions
Why spark is seen while removal of synthetic clothes in dark in winter?
View SolutionHow can you charge a metal sphere positively without touching it ?
View SolutionObtain the expression of electric field by a plane of infinite size and with uniform charge distribution.
View SolutionMass of photon in motion is ………
View SolutionThree pure inductance each of 3 H are connected as shown in figure. The equivalent inductance of this connection between points A and B is ......

View SolutionWrite the expression for electric intensity due to a charged conducting spherical shell at points outside and inside the shell.
View SolutionUsing Gauss' law deduce the expression for the electric field due to a uniformly charge spherical conducting shell of radius R at a point (i) outside the shell.
View SolutionWrite the expression for electric field intensity at any point outside and inside due to a charged spherical shell.
View SolutionState Gauss's theorem. Obtain an expression for elactric field at any point outside a charged spherical hollow conductor (shell).
View Solutionस्थिर वैधुतिकी में गाउस का प्रमेय लिखिए। इसका उपयोग करते हुए एकसमान आवेशित किसी पतले गोलीय खोल के बहार स्थित किसी बिंदु पर विधुत क्षेत्र हेतु व्यंजक विउत्पन्न व्युत्पन्न कीजिए।
View SolutionDerive an expression for electric intensity E due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionObtain the expression for electric field due to an uniformly charge spherical shell.
View SolutionObtain the expression for electric field due to an uniformly charge spherical shell.
View SolutionFind expression for electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionObtain the expression of electric field by a plane of infinite size and with uniform charge distribution.
View Solution
