Question

On a $120km$ track , a train travels the first $30km$ at a uniform speed of $30km/h$. How fast must the train travel the next $90km$ so as to average $60km/h$ for the entire trip?

Explanation

In this numrical problem we have been given the total distance travelled by the train (which is 120 km), and the average speed of the train for the whole journey (which is 60 km/h). From these two values we can calculate the total time taken by the train for the entire journey. This can be done as follows :
We know that, `" "` Average speed = `("Total distance travelled" )/("Total time taken")`
So, `" "` 60= `(120)/("Total time taken" )`
And, `" "` Total time taken = `(120)/(60)` hours
`" "` = 2 hours `" "`...(1)
We will now calculate the time taken by the train for the first 30 km journey, and the next 90 km journey, separaterly (see Figure 17).
(i) For the first part of the train journey, we have :
`" "` Speed = 30 km/h
`" "` Distance = 30 km
And, `" "` Time = ? `" "` (To be calculated )
Now, `" "` Speed = `("Distance travelled")/("Time taken")`
So, `" "30 =(30 )/("Time taken")`
And, `" "` Time taken = `(30)/(30)` hours
`" "`= 1 hour `" "` ...(2)

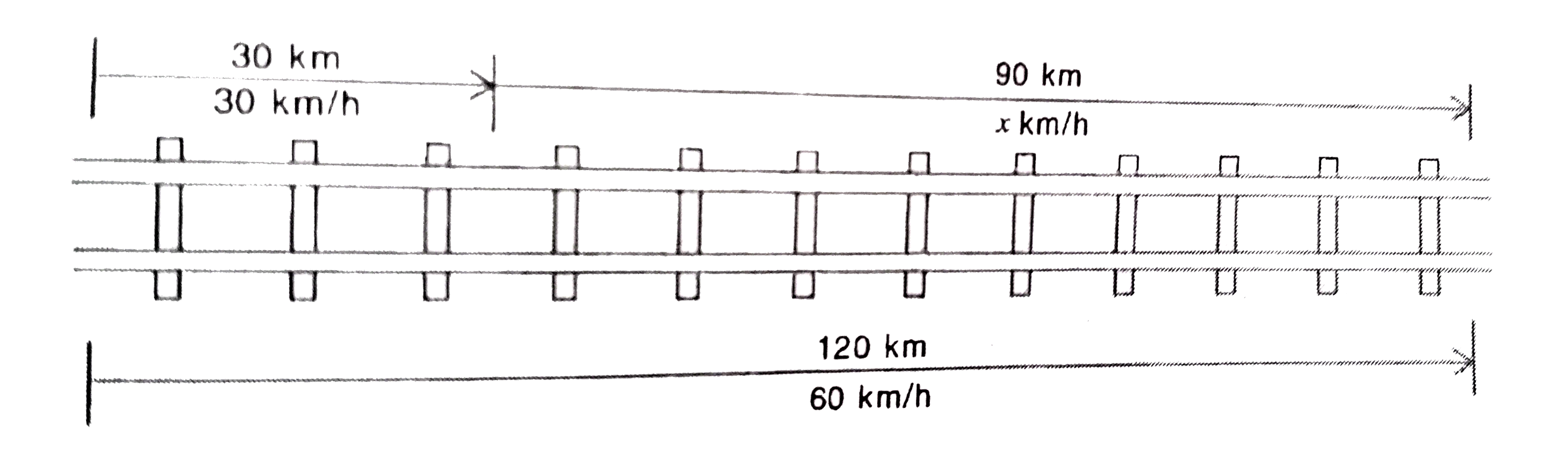
(ii) For the second part of the train journey, let us suppose that the speed of the train is x km/h. So, for the second part of the train journey, we have :
`" "` Speed = x km/h (Supposed)
And, `" "` Time = ? `" "` (To be calculated)
So, `" " x= (90)/("Time taken")`
And, `" "` Time taken = `(90)/(x)` hours `" "` ....(3)
Now, adding equations (2) and (3), we get the total time taken for the entire trip:
`" "` Total time taken = 1+ `(90)/(x)` hours `" "`...(4)
We already know from equation (1) that the total time taken for the entire trip is 2 hours, So, comparing equations (4) and (1), we get :
`" "1+(90)/(x)=2`
`" "(90)/(x)=2-1`
`" "(90)/(x)=1`
And `" "` x= 90 km/h
Thus, the train should travel the next 90 km distance at a speed of 90 km/h.