One end of a long iron chain of linear mass density λ is fixed to a sphere of mass m and specific density 1/3 while the other end is free. The sphere along with the chain is immersed in a deep lake. If specific density of iron is 7 , the height h above the bed of the lake at which the sphere will float in equilibrium is (assume that the part of the chain lying on the bottom of the lake exerts negligible force on the upper part of the the chain)

167mλ
7m3λ
5m2λ
8m3λ
The correct Answer is:B
Step by step video, text & image solution for One end of a long iron chain of linear mass density lambda is fixed to a sphere of mass m and specific density 1/3 while the other end is free. The sphere along with the chain is immersed in a deep lake. If specific density of iron is 7 , the height h above the bed of the lake at which the sphere will float in equilibrium is (assume that the part of the chain lying on the bottom of the lake exerts negligible force on the upper part of the the chain) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FLUID MECHANICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - FLUID MECHANICSEXERCISE - Advanced MCQ with one or more options correct
18 Videos
- FLUID MECHANICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - FLUID MECHANICSEXERCISE - Unsolved Numerical Problems
86 Videos
- FLUID MECHANICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - FLUID MECHANICSEXERCISE - MCQ
17 Videos
- GRAVITATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Unsolved Numerical
94 Videos
Similar Questions
One end of a long iron chain of linear mass density λ is fixed to a sphere of mass m and specific density 1/3 while the other end is free. The sphere slong with the chain is immersed in a deep lake. If specific density or iron is 7, the height h above the bed of the lake at which the lake at which the sphere will float in equillibrium is (Assume that the part of the chain lying on the bottom of the lake exerts negligible force on the upper part of the chain.).

View SolutionOne end of an iron chain is fixed to a sphere of mass M = 10 kg and of diameter D = 0.3 m (the volume of such a sphere is V=0.0141m3), while the other end is free. The length 1 of the chain is 3 m and its mass m is 9 kg. The sphere with the chain is in a reservoir whose depth H=3m.
Determine the depth at which the sphere will float, assuming that iron is 7.85 times heavier than water.
View Solution
Knowledge Check
If the density of iron is 7900kg m−3 , then its relative density is
A790B79C7.9D0.79If the density of a sphere is reduced to 1/8 of its original value, then the radius of the sphere is _________ times its original radius (assume that there is no change in mass). S
A2B4C1/2D1/4If the density of a sphere is reduced to 1/8 of its original value, then the radius of the sphere is _________ times its original radius (assume that there is no change in mass). S
A2B4C1/2D1/4
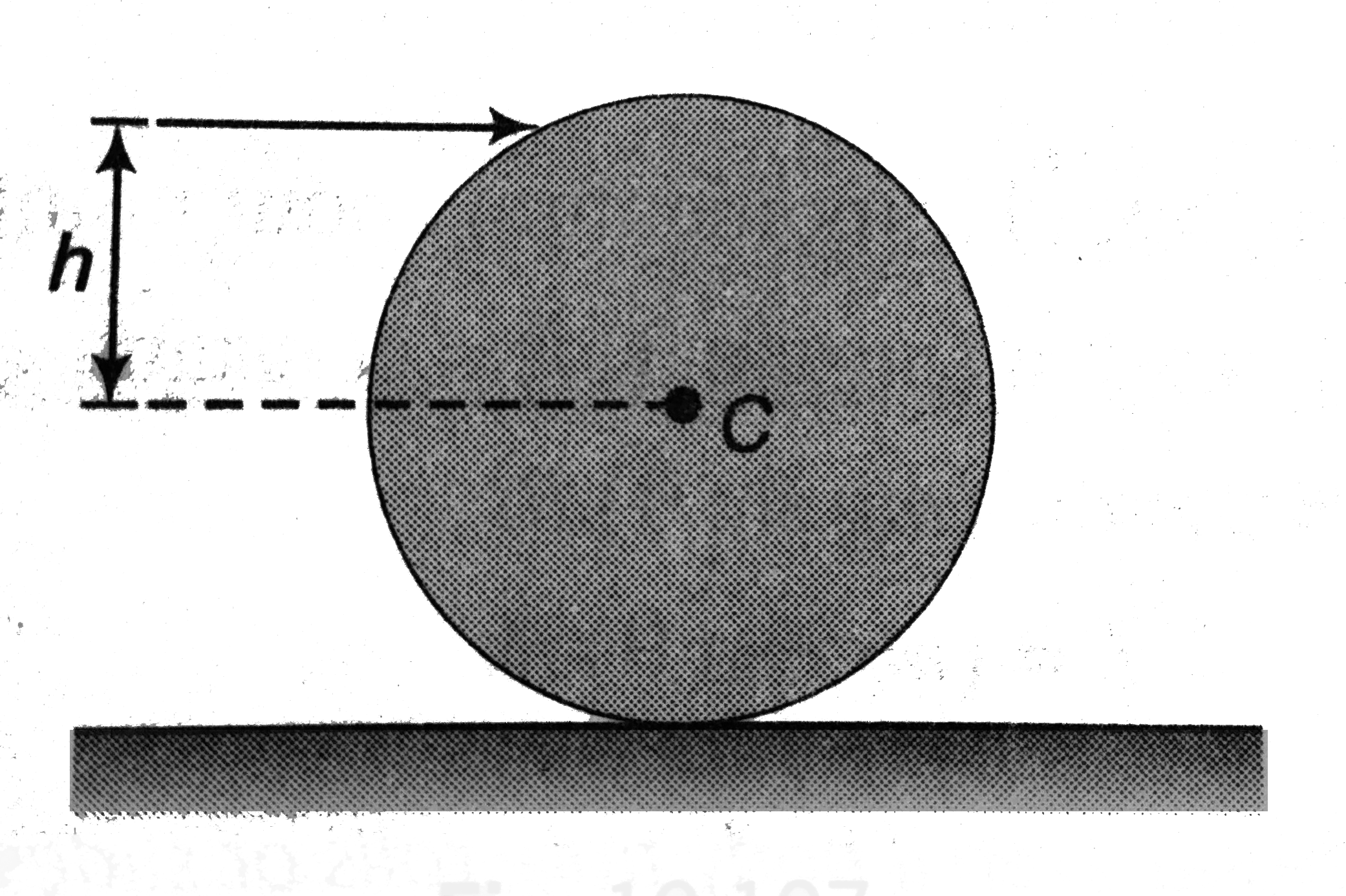
A solid sphere of mass M and radius R is hit by a cue at a height h above the centre C. for what value of h the sphere will rool without slipping ?
View SolutionCalculate work done in raising a stone of mass 5kg and specific gravity 3 lying at the bed of a lake through a height of 4 metre.
View SolutionA 10 m long iron chain of linear mass density 0.8 kg m−1 is hanging freely from a rigid support .If g=10 ms−2 ,then the power required to left the chain upto the point of support in 10 second
View SolutionStatement-1: Upthurst on a solid block of iron when immersed in a lake will be less on the surface than on the bed of the lake.
Statement-2: On the surface of the lake density of water will be less than that at the bed and upthrust depends on the density of liquid.
View SolutionIf the density of a sphere is reduced to 1/8 of its original value, then the radius of the sphere is _________ times its original radius (assume that there is no change in mass). S
View Solution
