One end of an iron chain is fixed to a sphere of mass M = 10 kg and of diameter D = 0.3 m (the volume of such a sphere is V=0.0141m3), while the other end is free. The length 1 of the chain is 3 m and its mass m is 9 kg. The sphere with the chain is in a reservoir whose depth H=3m.
Determine the depth at which the sphere will float, assuming that iron is 7.85 times heavier than water.
Step by step video & image solution for One end of an iron chain is fixed to a sphere of mass M = 10 kg and of diameter D = 0.3 m (the volume of such a sphere is V = 0.0141 m^(3)), while the other end is free. The length 1 of the chain is 3 m and its mass m is 9 kg. The sphere with the chain is in a reservoir whose depth H = 3m. Determine the depth at which the sphere will float, assuming that iron is 7.85 times heavier than water. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A sphere of radius 1.4 m weighs 500 kg. The sphere
View SolutionOne end of a long iron chain of linear mass density λ is fixed to a sphere of mass m and specific density 1/3 while the other end is free. The sphere slong with the chain is immersed in a deep lake. If specific density or iron is 7, the height h above the bed of the lake at which the lake at which the sphere will float in equillibrium is (Assume that the part of the chain lying on the bottom of the lake exerts negligible force on the upper part of the chain.).

View Solution
Knowledge Check
One end of a long iron chain of linear mass density λ is fixed to a sphere of mass m and specific density 1/3 while the other end is free. The sphere along with the chain is immersed in a deep lake. If specific density of iron is 7 , the height h above the bed of the lake at which the sphere will float in equilibrium is (assume that the part of the chain lying on the bottom of the lake exerts negligible force on the upper part of the the chain)
 A167mλB7m3λC5m2λD8m3λ
A167mλB7m3λC5m2λD8m3λA sphere of mass 10 kg and radius 0.5 m rotates about a tangent. The moment of inertia of the sphere is
A5kgm2B2.7kgm2C3.5kgm2D4.5kgm2A sphere of mass 10 kg and radius 0.5 m rotates about a tangent. The moment of inertia of the sphere is
A5 kg m2B2.7 kg m2C3.5 kg m2D4.5 kg m2
A sphere of mass 5kg and diameter 2m rotates about a tangent. What is its moment of inertia in kgm2 ?
View Solution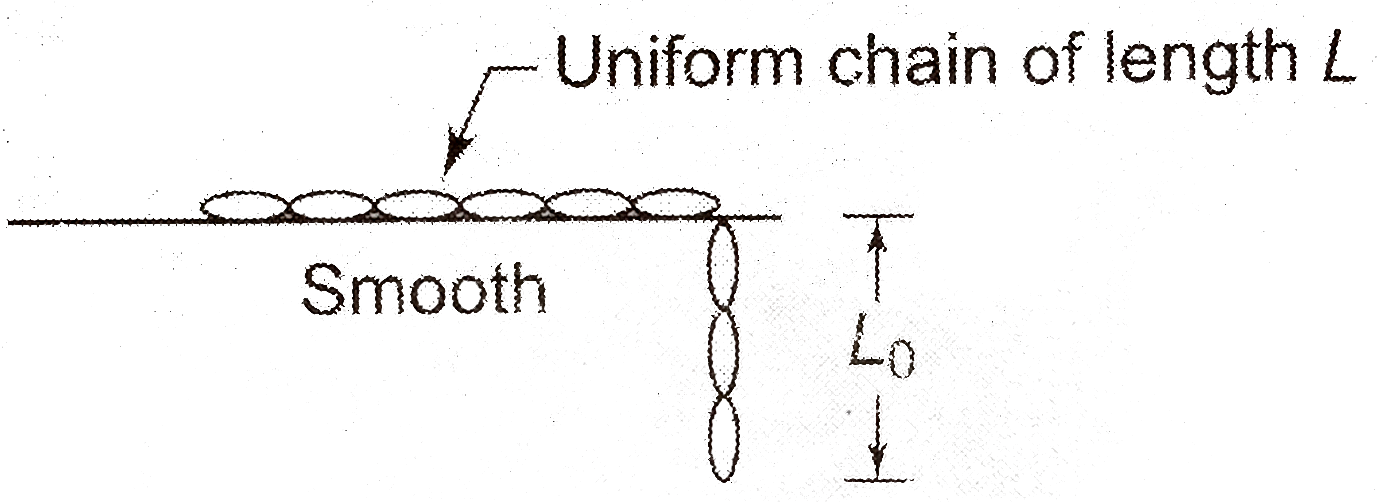
Consider a uniform chain of mass m and length L, the chain is released when hanging length is L0. Find the speed with which the chain leaves the table.
View SolutionFind the surface area of a sphere whose volume is 60.375m3
View SolutionA chain of length l is placed on a smooth spherical surface of radius R with one of its ends fixed at the top of the sphere. What will be the acceleration w of each element of the chain when its upper end is released? It is assumed that the length of the chain l<12πR.
View SolutionIf moment of inertia of a solid sphere of mass 5kg about its diameter is 50kgm2 . Its moment of inertia about its tangent in kg m2 is
View Solution
