Pipe A is open at both ends and has length $L_{A}$ = 0.343 m. We want to place it near three other pipes in which standing waves have been set up, so that the sound can set up a standing wave in pipe A. Those other three pipes are each closed at one end and have lengths $L_{B} = 0.500 L_{A}, L_{C} = 0.250 L_{A}$ and $L_{D}$ = 2.00L. For each of these three pipes, which of their harmonics can excite a harmonic in pipe A?

Text Solution

Verified by Experts

(1) The sound from one pipe can set up a standing wave it another pipe only if the harmonic frequencies match. (2) Equation gives the harmonic frequencies in a pipe with two open ends (a symmetric pipe) as f = nv/2L, for n 1,2,3,..., that is, for any positive integer. (3) Equation 17-40 gives the harmonic frequencies in a pipe with only one open end (an asymmetric pipe) as f= nv/4L, for n = 1, 3, 5, ..., that is, for only odd positive integers.
Pipe A: Let.s first find the resonant frequencies of symmetric pipe A (with two open ends) by evaluating Eq.
$f_{A} = \frac{n_{A} v}{2 L_{A}} = \frac{n_{A} (343 m / s)}{2 (0.434 m)}$
$= n_{A} (500 H z) = n_{A} (0.50 k H z), for o n_{A} = 1, 2, 3, ... ... ... .$
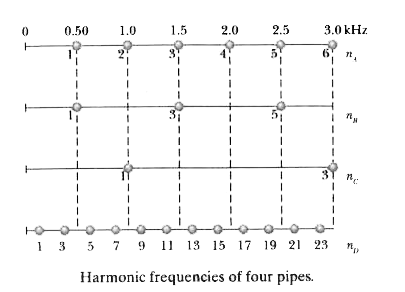
The first six harmonic frequencies are shown in the top plot in Fig.
Pipe B: Next let.s find the resonant frequencies of asymmetric pipe B (with only one open end) by evaluating Eq., being careful to use only odd integers for the harmonic numbers:
$f_{B} = \frac{n_{B} v}{4 L_{B}} = \frac{n_{B} v}{4 (0.500 L_{A})} = \frac{n_{B} (343 m / s)}{2 (0.343 m)}$
$n_{B} (500 H z) = n_{B} (0.500 k H z), for n_{B} = 1, 3, 5, ... . .$
Comparing our two results, we see that we get a match for each choice of $n_{B}$ :
$f_{A} = f_{B} for n_{A} = n_{B} with n_{B} = 1, 3, 5 ... ... ... ...$
For example, as shown in Fig. , if we set up the fifth harmonic in pipe B and bring the pipe close to pipe A, the fifth harmonic will then be set up in pipe A. However, no harmonic in B can set up an even harmonic in A.
Pipe C: Let.s continue with pipe C (with only one end) by writing Eq. as
$f_{C} = \frac{n_{C} v}{4 L_{C}} = \frac{n_{C} v}{4 (0.250 L_{A})} = \frac{n_{C} (343 m / s)}{0.343 m / s}$
$n_{C} (1000 H z) = n_{C} (1.00 k H Z), for n_{C} = 1, 3, 5, ... ...$
From this we see that C can excite some of the harmonics of A but only those with harmonic numbers n, that are twice an odd integer:
$f_{A} = f_{C} for n_{A} = 2 n_{C}, with n_{C} = 1, 3, 5, ... ... .$
Pipe D: Finally, let.s check D with our same procedure:
$f_{v} = \frac{n_{D} v}{4 L_{D}} = \frac{n_{D} v}{4 (2 L_{A})} = \frac{n_{D} (343 m / s)}{8 (0.343 m / s)}$
$= n_{D} (125 H z) = n_{D} (0.125 k H z), for n_{D} = 1, 3, 5, ... . .$
As shown in Fig., none of these frequencies match a harmonic frequency of A. (Can you see that we would get a match if $n_{D} = 4 n_{A}$ ? But that is impossible because $4 n_{A}$ cannot yield an odd integer, as required of $n_{D}$ .) Thus D cannot set up a standing wave in A.

Updated on:21/07/2023