Pressure exerted by a liquid at a given point is:
directly proportional to the depth of the point in liquid
directly proportional to the density of liquid
both (a) and (b)
none of these
To solve the question regarding the pressure exerted by a liquid at a given point, we can analyze the concepts of pressure in fluids step by step.
1. Understanding Pressure in Fluids:
- Pressure in a fluid is defined as the force exerted per unit area. In the case of liquids, this pressure can vary with depth and density.
2. Pressure and Depth:
- The pressure exerted by a liquid increases with depth. This is because the weight of the liquid above a certain point contributes to the pressure at that point.
- Mathematically, this can be expressed as:
P=h⋅ρ⋅g
where P is the pressure, h is the depth, ρ is the density of the liquid, and g is the acceleration due to gravity.
3. Pressure and Density:
- The pressure also depends on the density of the liquid. A denser liquid will exert more pressure at the same depth compared to a less dense liquid.
- For example, if you have two liquids at the same depth, the one with higher density will exert more pressure.
4. Combining the Effects:
- Therefore, the pressure at a given point in a liquid is directly proportional to both the depth of the liquid and the density of the liquid. This means that:
- If the depth increases, pressure increases.
- If the density increases, pressure also increases.
5. Conclusion:
- Based on the above analysis, the correct answer to the question "Pressure exerted by a liquid at a given point is:" is C) both A and B.
- Pressure in a fluid is defined as the force exerted per unit area. In the case of liquids, this pressure can vary with depth and density.
- The pressure exerted by a liquid increases with depth. This is because the weight of the liquid above a certain point contributes to the pressure at that point.
- Mathematically, this can be expressed as:
P=h⋅ρ⋅g
where P is the pressure, h is the depth, ρ is the density of the liquid, and g is the acceleration due to gravity.
- The pressure also depends on the density of the liquid. A denser liquid will exert more pressure at the same depth compared to a less dense liquid.
- For example, if you have two liquids at the same depth, the one with higher density will exert more pressure.
- Therefore, the pressure at a given point in a liquid is directly proportional to both the depth of the liquid and the density of the liquid. This means that:
- If the depth increases, pressure increases.
- If the density increases, pressure also increases.
- Based on the above analysis, the correct answer to the question "Pressure exerted by a liquid at a given point is:" is C) both A and B.
Topper's Solved these Questions
- FORCE AND PRESSURE BOOK - ICSECHAPTER - FORCE AND PRESSURE EXERCISE - EXERCISE 3.2 (STUDY QUESTIONS)
6 Videos
- FORCE AND PRESSURE BOOK - ICSECHAPTER - FORCE AND PRESSURE EXERCISE - EXERCISE 3.3 (FILL IN THE BLANK)
5 Videos
- FORCE AND PRESSURE BOOK - ICSECHAPTER - FORCE AND PRESSURE EXERCISE - EXERCISE 3.2 (WRITE TRUE OR FALSE)
4 Videos
- ENERGYBOOK - ICSECHAPTER - ENERGYEXERCISE - THEME ASSIGNMENT
4 Videos
- HEAT TRANSFER BOOK - ICSECHAPTER - HEAT TRANSFER EXERCISE - Picture Study
2 Videos
Similar Questions
Complete the following sentences:
(a) Pressure at a depth h in a liquid of density ρ is …......
(b) Pressure is …... In all direction about a point in a liquid.
(c ) Pressure at all points at the same depth is ….
(d) Pressure at a point inside a liquid is ....... to its depth.
(e ) Pressure of a liquid at a given depth is ....... to the density of liquid.
View SolutionPressure exerted by a liquid ________ with the increase in depth.
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.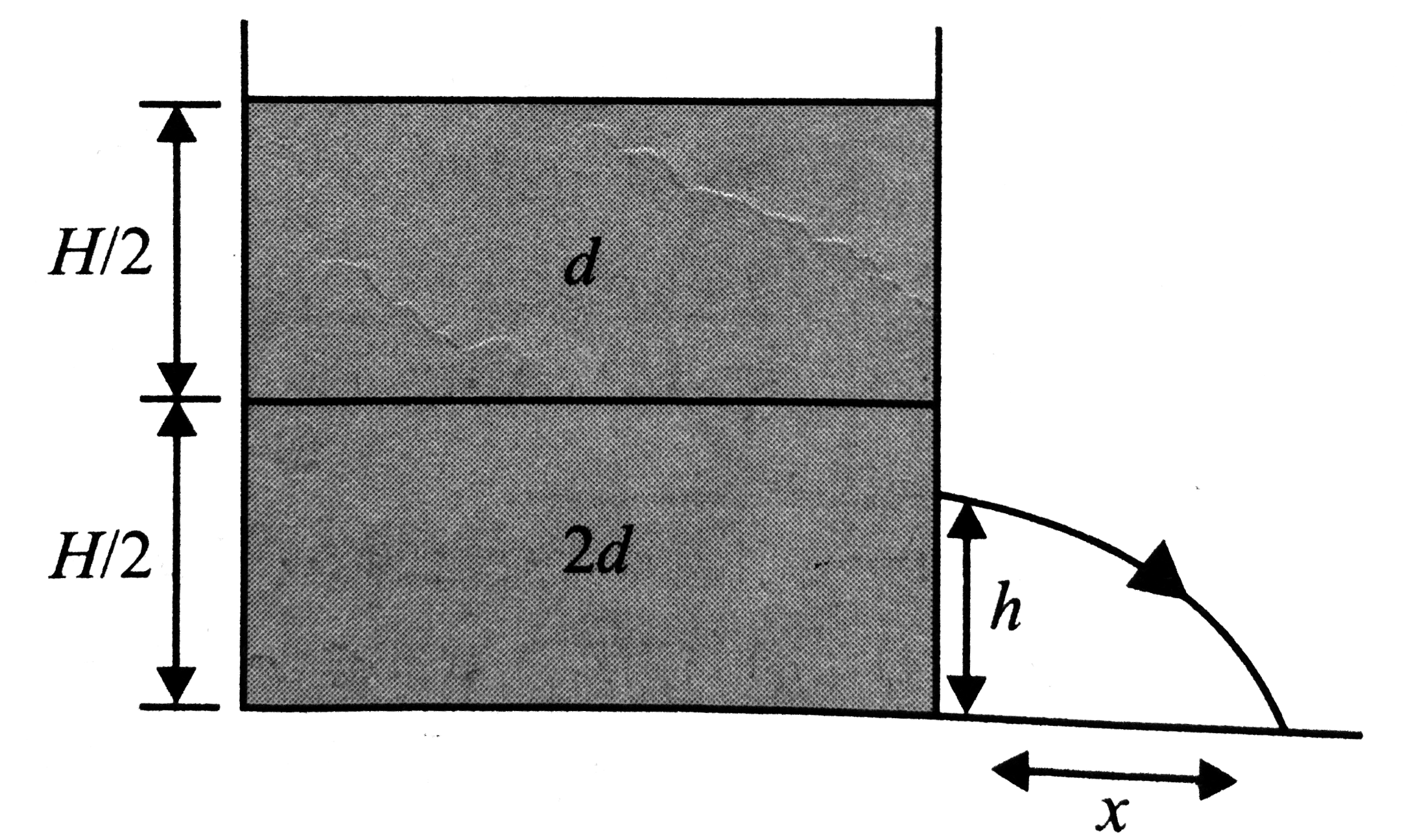
Situation I:
A homogeneous solid cylinder of length L(L<H/2). cross sectional area A/5 is immersed such that it floats with its axis vertical at liquid -liquid interface with lenght L/4 in the denser liquid.
The total pressure at the bottom of the container is
View SolutionPressure exerted by a perfect gas is equal to
View SolutionHow does the pressure exerted by a solid and a fluid differ ?
View SolutionPressure exerted by a liquid __________ with the decrease in the density of liquid .
View SolutionThe total pressure exerted in ideal binary solution is given by P=P∘AXA+P∘BXB where P∘A&P∘B are the respective vapour pressure of pure components and XA&XB are their mole fraction in liquid phase. And composition of the vapour phase is determined with the help of Datton's law partial pressure: YA=P∘AXAP
If total pressure exerted in an ideal binary solution is given by P=540060+30YAmm of Hg.
The more volatile liquid is:
View SolutionThe pressure exerted by real gas is :-
View SolutionThe total pressure exerted in ideal binary solution is given by P=P∘AXA+P∘BXB where P∘A&P∘B are the respective vapour pressure of pure components and XA&XB are their mole fraction in liquid phase. And composition of the vapour phase is determined with the help of Datton's law partial pressure: YA=P∘AXAP
If total pressure exerted in an ideal binary solution is given by P=540060+30YAmm of Hg.
The value of P∘A is:
View SolutionThe total pressure exerted in ideal binary solution is given by P=P∘AXA+P∘BXB where P∘A&P∘B are the respective vapour pressure of pure components and XA&XB are their mole fraction in liquid phase. And composition of the vapour phase is determined with the help of Datton's law partial pressure: YA=P∘AXAP
If total pressure exerted in an ideal binary solution is given by P=540060+30YAmm of Hg.
If the value of YA=0.4 then the value of XB is:
View Solution
