Protons having a kinetic energy of 50 eV are moving in the positive x-direction and enter a magnetic field B=0.5mˆkT directed out of the plane of the page and extending from x=0→x=1m as shown in Fig. 1.34.
a. Calculate the y-component of the protons' momentum as they leave the magnetic field.
b. Find the angle ϕ between the initial velocity vector of the proton beam and the velocity vector after the beam emerges from the field. Ignore relativistic effects and note that 1eV=1.60×10−19J.
(a) E=5MeV=5×1.6×10−13J
12mv2=5×1.6×10−13
v=√16×10−13m
r=mveB
1=rsinϕ
⇒sinϕ=1r=eBmv
y-component of the velocity is vsinϕ.
Hence y-component of momentum is mvsinϕ=eB
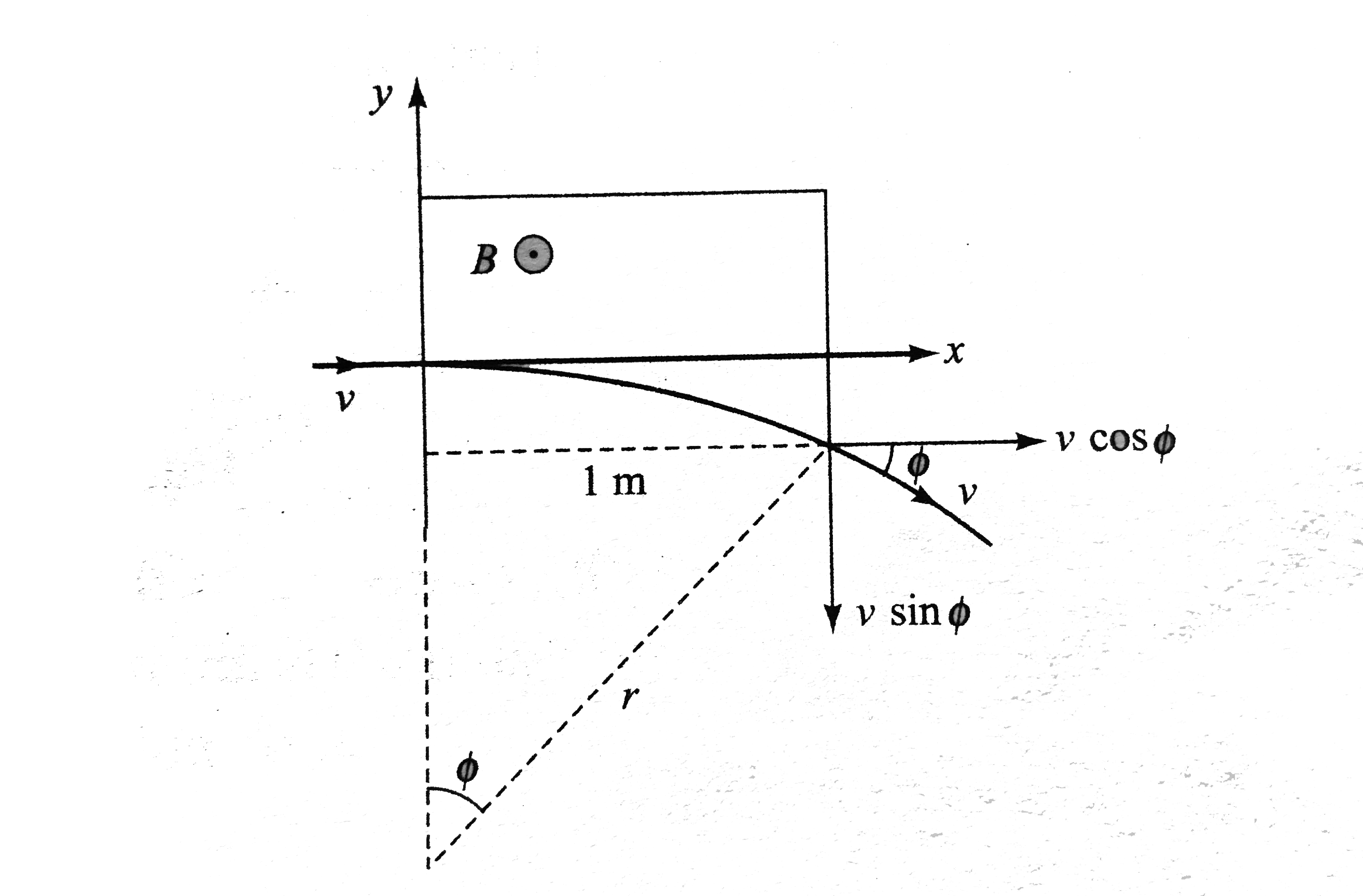
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.2
13 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise1.3
14 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Solved Example
25 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False
3 Videos
Similar Questions
A proton is moving along the negative direction of X-axis in a magnetic field directed along the positive direction of Y-axis. The proton will be deflected along the negative direction of
View SolutionA uniform magnetic field exists in the plane of paper pointing from left to right as shown in figure. In the field, an electron and a proton move as shown. The electron and the proton experience:
View Solution
Knowledge Check
An electron and a proton enter a magnetic field at right angles to the field with the same kinetic energy
Athe electron trajectory will be less curved than the proton trajectoryBthe proton trajectory will be less curved than the electron trajectoryCboth trajectories will be equally curvedDboth particles move in straight linesAn electron having momentum 2.4×10−23 kg m s−1 enters a region of uniform magnetic field of 0.15 T. The field vector makes an angle of 30∘ with the initial velocity vector of the electron. The radius of the helical path of the electron in the field shall be
A2mmB1mmC√32 mmD0.5mm
A magnet of magnetic moment 50ˆiA−m2 is placed along the x-axis in a magnetic field →B=(0.5ˆi+3.0ˆj)T. The torque acting on the magnet is
View SolutionA proton and an electron are projected into a region of uniform magnetic field in a direction perpendicular to the field. If they have the same initial velocities then
View SolutionA proton of charge e and mass m enters a uniform magnetic field B=Bi with an initial velocity v=vxˆi+vyˆj. Find an expression in unit vector notation for its velocity at time t.
View SolutionUniform electric and magnetic fields with strength E and induction B, respectively, are along y-axis as shown in Fig. A particle with specific charge q/m leaves the origin O in the direcction of x-axis with an initial non-relativistic velocity v0

The angle α between the particle's velocity vector and y-axis at and moment is
View SolutionA proton and an electron both moving with the same velocity v enter into a region of magnetic field directed perpendicular to the velocity of the particles. They will now move in cirular orbits such that
View SolutionA proton and a deuteron having equal momenta enter in a a region of a uniform magnetic field at right angle to the direction of a the field. What is the ratio of their radius of trajectory?
View Solution
