Radius of orbit of satellite of earth is R. Its kinetic energy is proportional to
1R
1√R
R
1R3/2
The correct Answer is:A
To determine how the kinetic energy of a satellite in orbit is related to the radius of its orbit (R), we can follow these steps:
1. Understand the orbital velocity of the satellite:
The orbital velocity (v) of a satellite in a circular orbit around a planet (like Earth) is given by the formula:
v=√GMR
where G is the gravitational constant and M is the mass of the Earth.
2. Write the expression for kinetic energy:
The kinetic energy (KE) of the satellite can be expressed as:
KE=12mv2
where m is the mass of the satellite.
3. Substitute the expression for orbital velocity into the kinetic energy formula:
By substituting v from step 1 into the kinetic energy formula, we get:
KE=12m(√GMR)2
4. Simplify the expression:
Squaring the velocity gives:
KE=12mGMR
This can be rewritten as:
KE=GMm2R
5. Identify the proportionality:
From the expression KE=GMm2R, we can see that the kinetic energy is inversely proportional to the radius R. Therefore, if we consider the relationship in terms of proportionality, we can say:
KE∝1R
Conclusion:
The kinetic energy of the satellite is inversely proportional to the radius of its orbit R.
The orbital velocity (v) of a satellite in a circular orbit around a planet (like Earth) is given by the formula:
v=√GMR
where G is the gravitational constant and M is the mass of the Earth.
The kinetic energy (KE) of the satellite can be expressed as:
KE=12mv2
where m is the mass of the satellite.
By substituting v from step 1 into the kinetic energy formula, we get:
KE=12m(√GMR)2
Squaring the velocity gives:
KE=12mGMR
This can be rewritten as:
KE=GMm2R
From the expression KE=GMm2R, we can see that the kinetic energy is inversely proportional to the radius R. Therefore, if we consider the relationship in terms of proportionality, we can say:
KE∝1R
The kinetic energy of the satellite is inversely proportional to the radius of its orbit R.
Topper's Solved these Questions
- GRAVITATIONBOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - AIIMS Questions
21 Videos
- GRAVITATIONBOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - Chapter Test
29 Videos
- GRAVITATIONBOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - Assertion Reasoning
16 Videos
- GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONBOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Chapter Test
30 Videos
- KINETIC THEORY OF GASES AND THERMODYNAMICSBOOK - A2ZCHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Chapter Test
29 Videos
Similar Questions
At what height above Earth's surface is the energy required to lift a satellite to that height equal to the kinetic energy required for the satellite to be in orbit at that height ?(b) For greater heights, which is greater, the energy for lifting or the kinetic energy for orbiting ?
View SolutionA satellite is orbiting earth at a distance r. Variations of its kinetic energy. potential energy and total energy, is sohwn in the figure. Of the three curves shown in figure, identify the type of mechanical energy they represent.
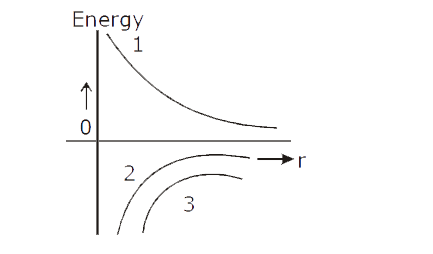
View Solution
Knowledge Check
The radius of the orbit of a satellite is r and its kinetic energy is k if the radius of the orbit is doubled then the new kinetic energy k is
A2kBk2C4 kDdata insufficientIn a satellite if the time of revolution is T, then kinetic energy is proportional to
A1TB1T2C1T3DT2/3The time period of a satellite in a circular orbit around the earth is T . The kinetic energy of the satellite is proportional to T−n . Then, n is equal to :
A12B23C43D32
If radius of an orbitating satellite is decreased , then its kinetic energy
View SolutionSuppose the force of gravitation is inversely proportional to the cube of the radius of circular orbit in which satellite is revolving then its time period is proportional to
View SolutionLet kinetic energy of a satellite is x, then its time of revolution T is proportional to
View SolutionAssertion : It we double the circular radius of a satellite, then its potential energy, kinetic energy and total mechanical energy will become half.
Reason : Orbital speed of a satellite.
υ∝1√r
where, r is its radius of orbit.
View SolutionThe mean radius of the orbit of a satellite is 4 times as great as that of the parking orbit of the earth. Then its period of revolution around the earth is
View Solution
