S1 and S2 are two equipotential surfaces on which the potentials are not equal
S1 and S2 cannot intersect.
S1 and S2 cannot both be plane surfaces.
In the region between S1 and S2 the field is maximum where they are closest to each other.
A line of force from S1 and S2 must be perpendicular to both.
The correct Answer is:A, C
Step by step video, text & image solution for S_(1) and S_(2) are two equipotential surfaces on which the potentials are not equal by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
In an equipotential surface, the value of potential at all points on the
AIs differentBis constantCmay be differentDmay be constant
On the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
If a charge of 2 nC is taken from equipotential surface number 2 to 3, what is the amount of work done ?
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Four equipotential surfaces are shown in the adjoining figure. Find the magnitude and direaction of the electric field.
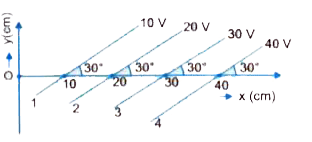
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Draw equipotential surfaces around an isolated positive point charge + q. Mark direction of electric field →E also.
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
If electric potential at origin point O be 100 V, then for electric field specified in part (b), draw equipotential surfaces corresponding to potentials 80 V, 60 V and 40 V respectively.
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
A uniform electric field →E=1000ˆiVm−1 exists in space at a given place. What is the shape of equipotential surfaces there ?
View SolutionCan two equipotential surfaces intersect each other? Give reasons.
View SolutionJustify why two equipotential surfaces cannot intersect.
View Solution