Show how internal energy U varies with T in isochoric, isobaric and adiabatic process?
The correct Answer is:A, B, C, D
For all process,
ΔU=nCVΔT
=nRΔTγ−1 (as CV=Rγ−1)
Topper's Solved these Questions
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Single Correct
27 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 More Than One Correct
6 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 1 Objective Questions
1 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - DC PANDEYCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Integer type Questions
10 Videos
Similar Questions
Knowledge Check
The relation between internal energy U, pressure p and volume V of a gas in an adiabatic process is U=2a+bpV
where a and b are constants. What is the effective value of adiabatic constant γ?A2b+12bBb+1bCb2+1b2D(b+1b)2The relation between internal energy U, pressure P and volume V of a gas in an adiabatic process is
U=a+bPV where a and b are constant . What is the effective value of adiabatic constant γ.AabBb+1bCa+1aDbaThe relation between internal energy U, pressure P and volume V of a gas in an adiabatic process is
U=a+bPV
where a and b are positive constants. What is the value of γ ?AabBb+1bCa+1aDba
The relation between internal energy U, pressure P and volume V of a gas in an adiabatic processes is : U=a+bPV where a = b = 3 . Calculate the greatest integer of the ratio of specific heats [γ] .
View SolutionSolve the previous problem for the cases of an isochoric, an isobaric and an isothermic process.
View SolutionOne mole of an ideal gas is carried through a thermodynamics cycle as shown in the figure. The cycle consists of an isochoric, an isothermal and an adiabatic process. Find the adiabatic exponent of the gas.
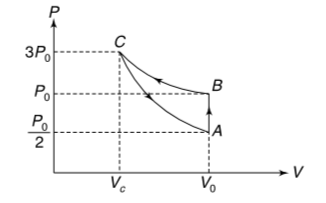
View SolutionAn ideal diatomic gas, without vibrations loses heat to a system in a process. Is the resulting decrease in the internal energy of the gas greater if the loss occurs in isochoric process or in isobaric process.
View SolutionTemperature of a monoatomic gas is increased from T0 to 2T0 in three different processes:
isochoric, isobaric and adiabatic. Heat given to the gas in these three processes are Q1,Q2andQ3 respectively. Then, choose the correct option.
View Solution
