Show that in open organ pipe, all harmonics are present.
To show that in an open organ pipe, all harmonics are present, we can follow these steps:
Step 1: Understanding the Open Organ Pipe
An open organ pipe is open at both ends. This means that at both ends of the pipe, there are antinodes (points of maximum amplitude) of the wave.
Step 2: Visualizing the First Harmonic
The first harmonic (fundamental frequency) can be represented by the simplest wave that fits in the pipe. For an open pipe, the length L of the pipe corresponds to half the wavelength λ of the wave:
L=λ2
From this, we can express the wavelength:
λ=2L
Step 3: Calculating the Frequency of the First Harmonic
The frequency f of the wave is given by the formula:
f=Vλ
Substituting the value of λ:
f1=V2L
This is the fundamental frequency f0.
Step 4: Visualizing Higher Harmonics
To find higher harmonics, we need to fit more wavelengths into the same length L. The second harmonic will have one full wavelength fitting in the pipe:
L=λ
Thus, the wavelength for the second harmonic is:
λ=L
Calculating the frequency:
f2=Vλ=VL
Step 5: Continuing to Higher Harmonics
For the third harmonic, we add another half wavelength:
L=3λ2
This gives us:
λ=2L3
Calculating the frequency:
f3=Vλ=V2L3=3V2L
Step 6: Generalizing the Harmonics
From the pattern observed, we can generalize the frequency for the nth harmonic:
fn=n⋅V2L
Where n can take any positive integer value (1, 2, 3, ...).
Conclusion
Since n can be any positive integer, this means that all harmonics (both odd and even) are present in an open organ pipe. Therefore, we conclude that in an open organ pipe, all harmonics are indeed present.
---
An open organ pipe is open at both ends. This means that at both ends of the pipe, there are antinodes (points of maximum amplitude) of the wave.
The first harmonic (fundamental frequency) can be represented by the simplest wave that fits in the pipe. For an open pipe, the length L of the pipe corresponds to half the wavelength λ of the wave:
L=λ2
From this, we can express the wavelength:
λ=2L
The frequency f of the wave is given by the formula:
f=Vλ
Substituting the value of λ:
f1=V2L
This is the fundamental frequency f0.
To find higher harmonics, we need to fit more wavelengths into the same length L. The second harmonic will have one full wavelength fitting in the pipe:
L=λ
Thus, the wavelength for the second harmonic is:
λ=L
Calculating the frequency:
f2=Vλ=VL
For the third harmonic, we add another half wavelength:
L=3λ2
This gives us:
λ=2L3
Calculating the frequency:
f3=Vλ=V2L3=3V2L
From the pattern observed, we can generalize the frequency for the nth harmonic:
fn=n⋅V2L
Where n can take any positive integer value (1, 2, 3, ...).
Since n can be any positive integer, this means that all harmonics (both odd and even) are present in an open organ pipe. Therefore, we conclude that in an open organ pipe, all harmonics are indeed present.
Similar Questions
Knowledge Check
- Question 1 - Select One
A closed organ pipe in 3rd harmonic and an open organ pipe in 5th harmonic mode are in resonance with a tuning fork P. The ratio of lengths of closed to open organ pipe will be
A3:10B10:3C5:4D10:5 - Question 2 - Select One
Modes of vibration in an open organ pipe are represented by –
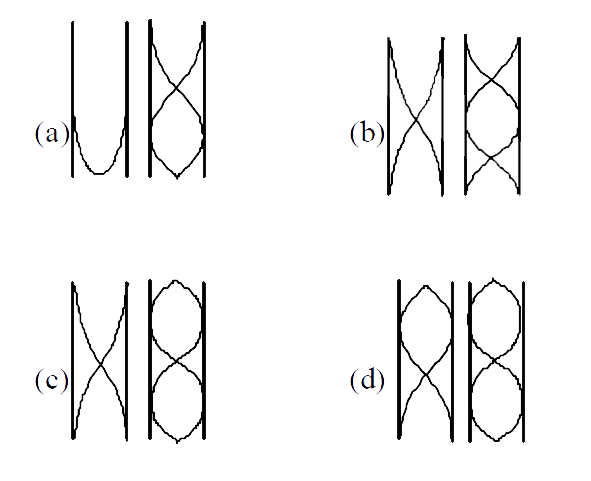 AaBbCcDd
AaBbCcDd - Question 3 - Select One
Which of the following statements related to organ pipe is/are correct?
I. In a closed organ pipe longitudinal standing waves can be formed,
II. In a closed organ pipe (closed at one end) only odd harmonics are present.
III. The harmonics which are present in a pipe, open at both ends are odd harmonics only Choose the correct option those given below.AOnly IBII and IIICI and IIDI.II and III
In an open organ pipe, third harmonic has a frequency 450Hz. What is the frequency of fifth harmonic ?
View SolutionShow that in a closed end organ pipe the first three harmonics are in the ratio of 1 : 3 : 5.
View SolutionIn an……………………Organ pipe, both odd and even harmonics are present.
View SolutionIn an open organ pipe, second harmonic is 400 Hz. Find the frequency of sixth harmonic.
View SolutionIn an open organ pipe, second harmonic is 200 Hz. Find the frequency of 5th harmonic.
View Solution

