Show that the vectors a=3ˆi−2ˆj+ˆk,b=ˆi−3ˆj+5ˆk and c=2ˆj+ˆj−4ˆk form a right angled triangle.
Video Solution
Text Solution
Given , a=3ˆi−2ˆj+5ˆkandb=ˆi−3ˆj+5ˆk
c=2ˆi+ˆj−4ˆk
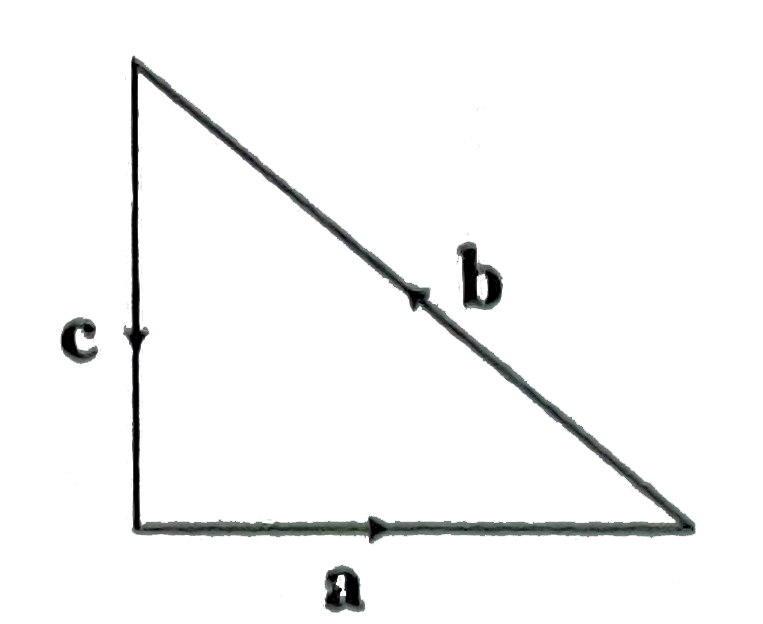
We have B+c=(ˆi−3ˆj+5ˆk)+(2ˆiˆj−4ˆk)
=3ˆi−2ˆj+ˆk=a
hence ,abandc are coplana.
Also, We observe that no two of these vectors are vectors are parallel, therefore, thegiven vectors form a triangle .Further,
a.c=(3ˆi−2ˆj+ˆk).(2ˆi+ˆj−4ˆk)=O
Dot product of two non-zero vectors is zero. hence ,they are perpendicular so they from a right angled triangle.
∴magnitude of a,|a|=√9+4+1=√14,
magnitude of b,|b|=√1+9+25=√35
and magnitude ofc,|c|=√4+1+16=√21
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The three vectors →A=3ˆi−2ˆj+ˆk,→B=ˆi−3ˆj+5ˆk and →C=2ˆi+ˆj−4ˆk form
AAn equilateral triangleBIsosceles triangleCA right angled triangleDNo triangle
Show that vectors 3ˆi−2ˆj+2ˆk,ˆi−3ˆj+4ˆkand2ˆi−ˆj−2ˆk form a right angled triangle
View SolutionThe three vectors →A=3ˆi≡2ˆj+kˆ,→B=ˆi−3ˆj+5ˆkand→C=2ˆi+ˆj−4ˆk form
View SolutionProve that the points 2ˆi−ˆj+ˆk,ˆi−3ˆj−5ˆkand3ˆi−4ˆj−4ˆk are the vertices of a righat angled triangle. Also find the remaining angles of the triangle.
View SolutionShow that the vectors ˆi−ˆj−ˆk,2ˆi+3ˆj+ˆkand7ˆi+3ˆj−4ˆk are coplanar.
View SolutionShow that the vectors ˆi−3ˆj+2ˆk,2ˆi−4ˆj−ˆkand3ˆi+2ˆj−ˆk are linearly independent
View SolutionShow that the vectors 4ˆi−ˆj+ˆk,3ˆi−2ˆj−ˆk and ˆi+ˆj+2ˆk are co-planar.
View SolutionThe three vectors →A=3ˆi−2ˆj−ˆk,¯¯¯B=ˆi−3ˆj+5ˆkand¯¯¯C=2ˆi−ˆj−4ˆk does not form
View Solution
