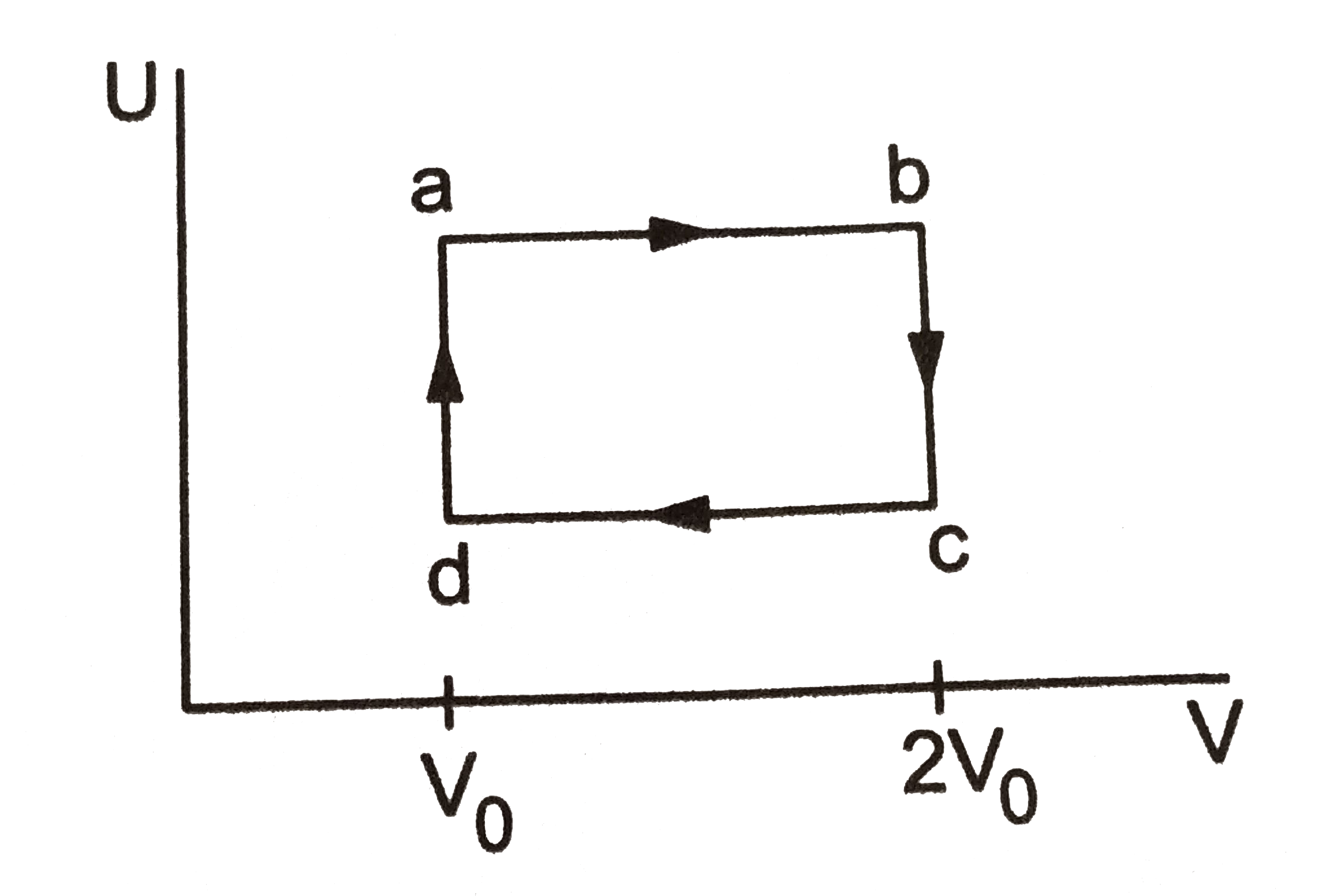
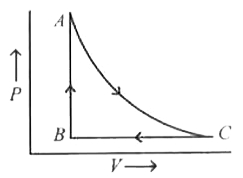
shows the variation in the internal energy U with the volume V of 2.0 mol of an ideal gas in a cyclic process abcda. The temperatures of the gas at b and c are 500 K and 300 K respectively. Calculate the heat absorbed by the gas during the process.
The correct Answer is:B, C
Given ,.DeltaV = 0In ab and bcHence DeltaW = DeltaQDeltaW =DeltaW_AB+DeltaW_CD = nRT_1 In((2V_0)/(V_0)+nRT_2 In((V_0)/(2V_0))nRxx2.303xxlog 2xx(500-300)= 2xx8.314xx2.303xx0.301xx200 = 2305.31J
Topper's Solved these Questions
- LAWS OF THERMODYNAMICSBOOK - HC VERMACHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Short Answer
15 Videos
- LAWS OF THERMODYNAMICSBOOK - HC VERMACHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Objective 2
5 Videos
- KINETIC THEORY OF GASESBOOK - HC VERMACHAPTER - KINETIC THEORY OF GASESEXERCISE - Exercises
62 Videos
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMACHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Exercises
42 Videos
Similar Questions
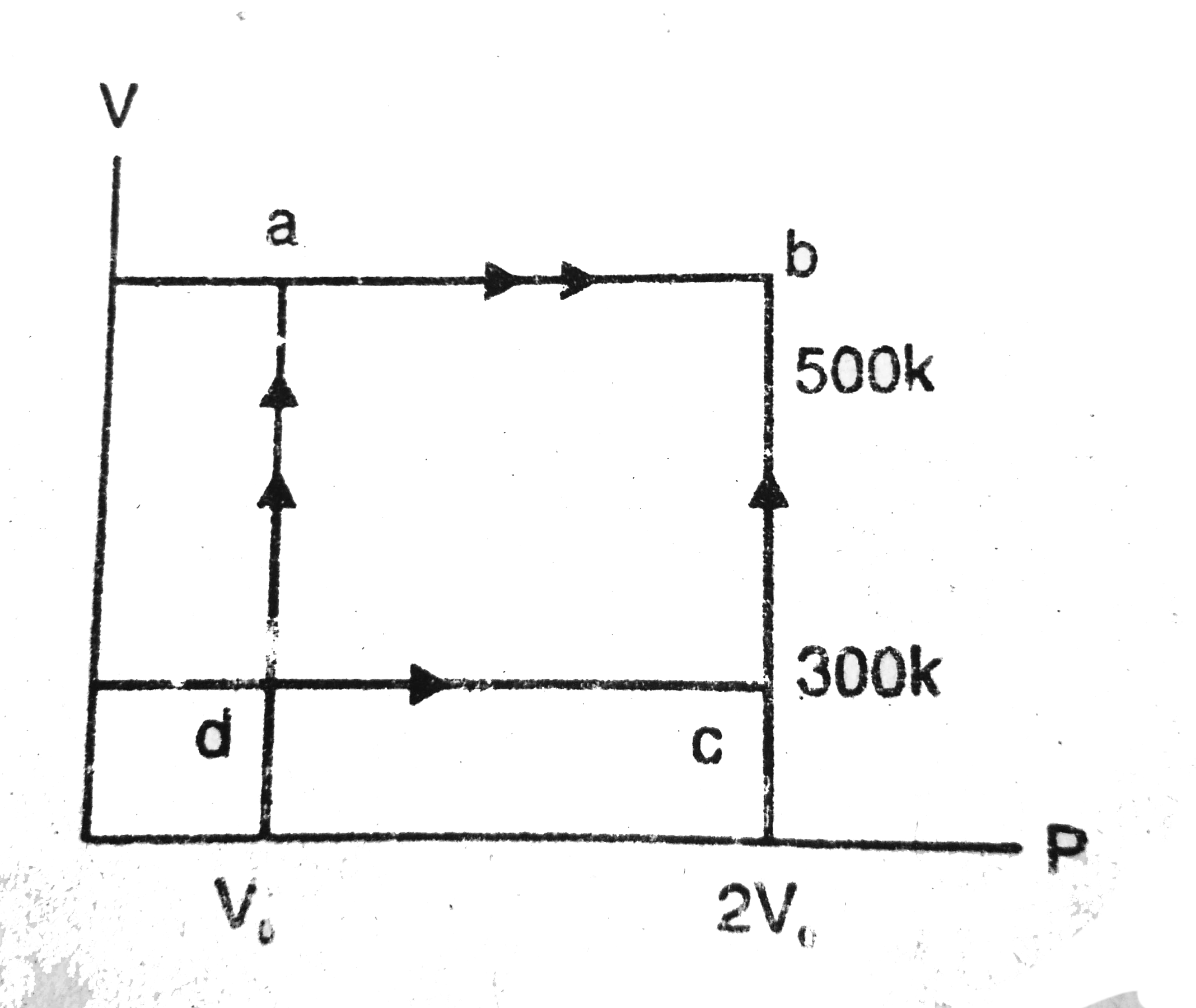
Fig. shows the variation of internal energy (U) with the pressure (P) of 2.0 mole gas in cycle process abcda. The temperatures of gas at c and d are 300 and 500K, respectively. Calculate the heat absorbed by the gas during the process.

View SolutionFigure shows the variation of internal energy (U) with the pressure (P) of 2.0 mole gas in cyclic process abcda.The temperature of gas at c and d are 300and500K respectively. The heat absorbed by the gas during the process is X×10Rln2 . Find the value of x.
View Solution
Knowledge Check
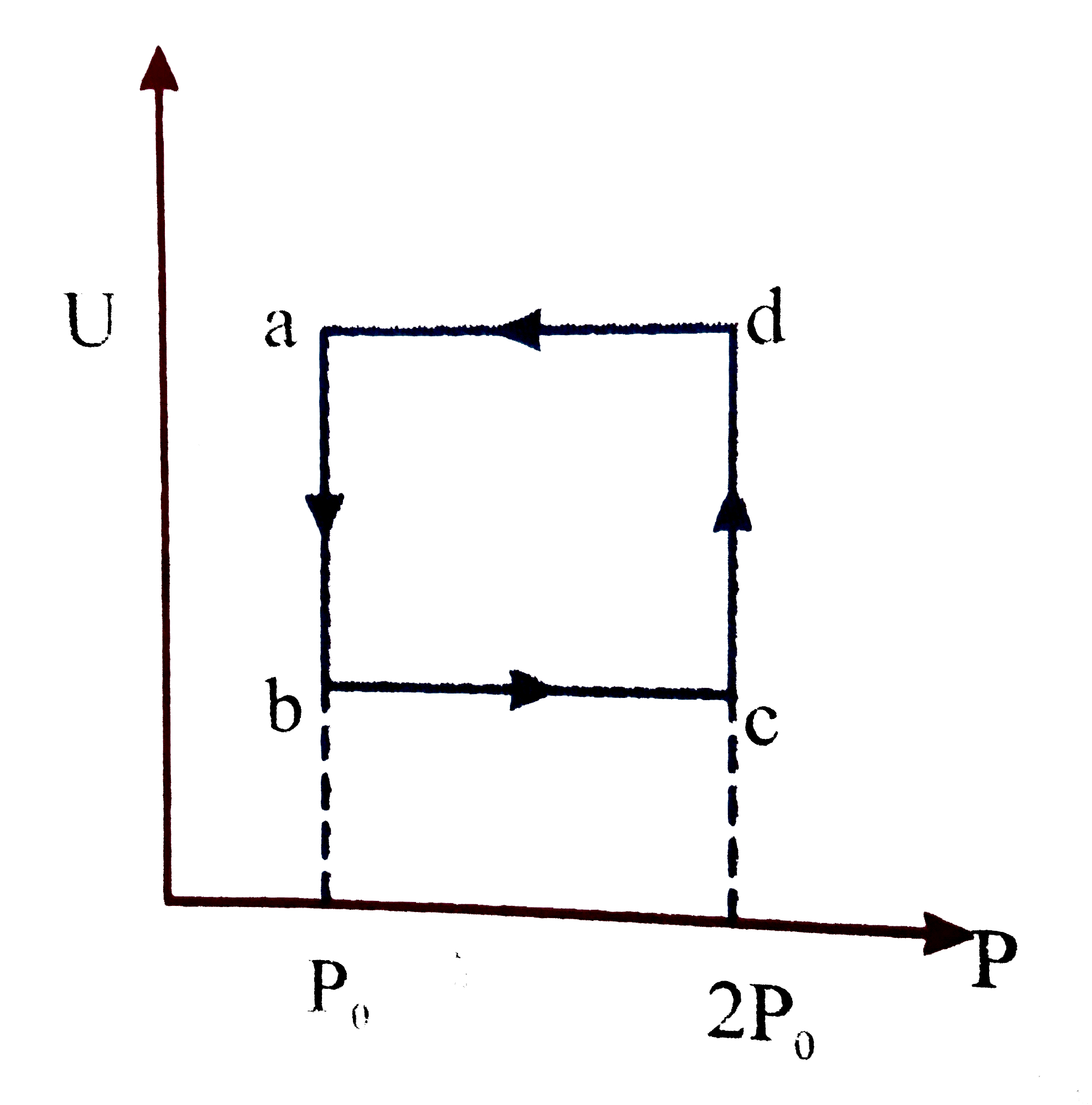
Figure shows the variation of internal energy (U) with the pressure (P) of 2.0mole gas in cyclic process abeda. The temperature of gas at c and d are 300 and 500 K. How much will be the heat absorbed by the gas during the process?
 A400R In 2B100 Rin 2C100 Rin2D50 Rin 2
A400R In 2B100 Rin 2C100 Rin2D50 Rin 2One mole of an ideal monoatomic gas undergoes a cyclic process, as shown in the figure. If the temperature of the gas at state 1 is 300 K and at state 4 is 500 K, then heat exchanged during process 2→3, is
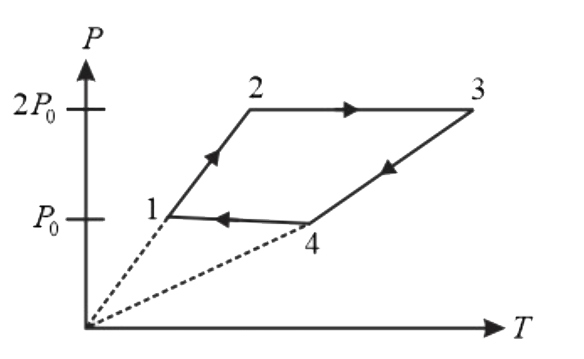 A1000 RB600 RC750 RD800 R
A1000 RB600 RC750 RD800 ROne mole of an ideal gas is taken through a cyclic process. The minimum temperature during the cycle is 300K. Then, net exchange of heat for complete cycle is
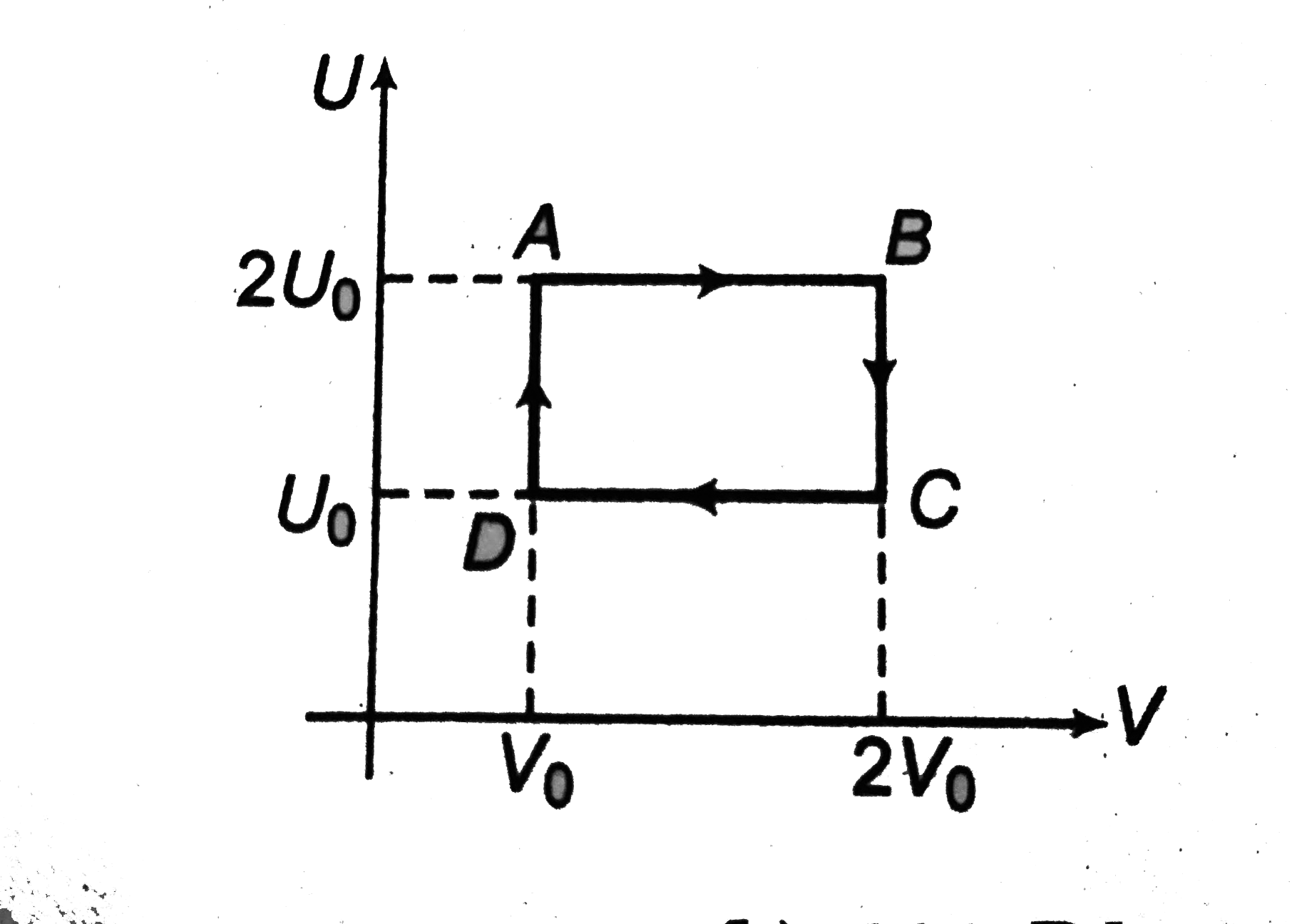 A600Rln2B300Rln2C−300Rln2D900Rln2
A600Rln2B300Rln2C−300Rln2D900Rln2
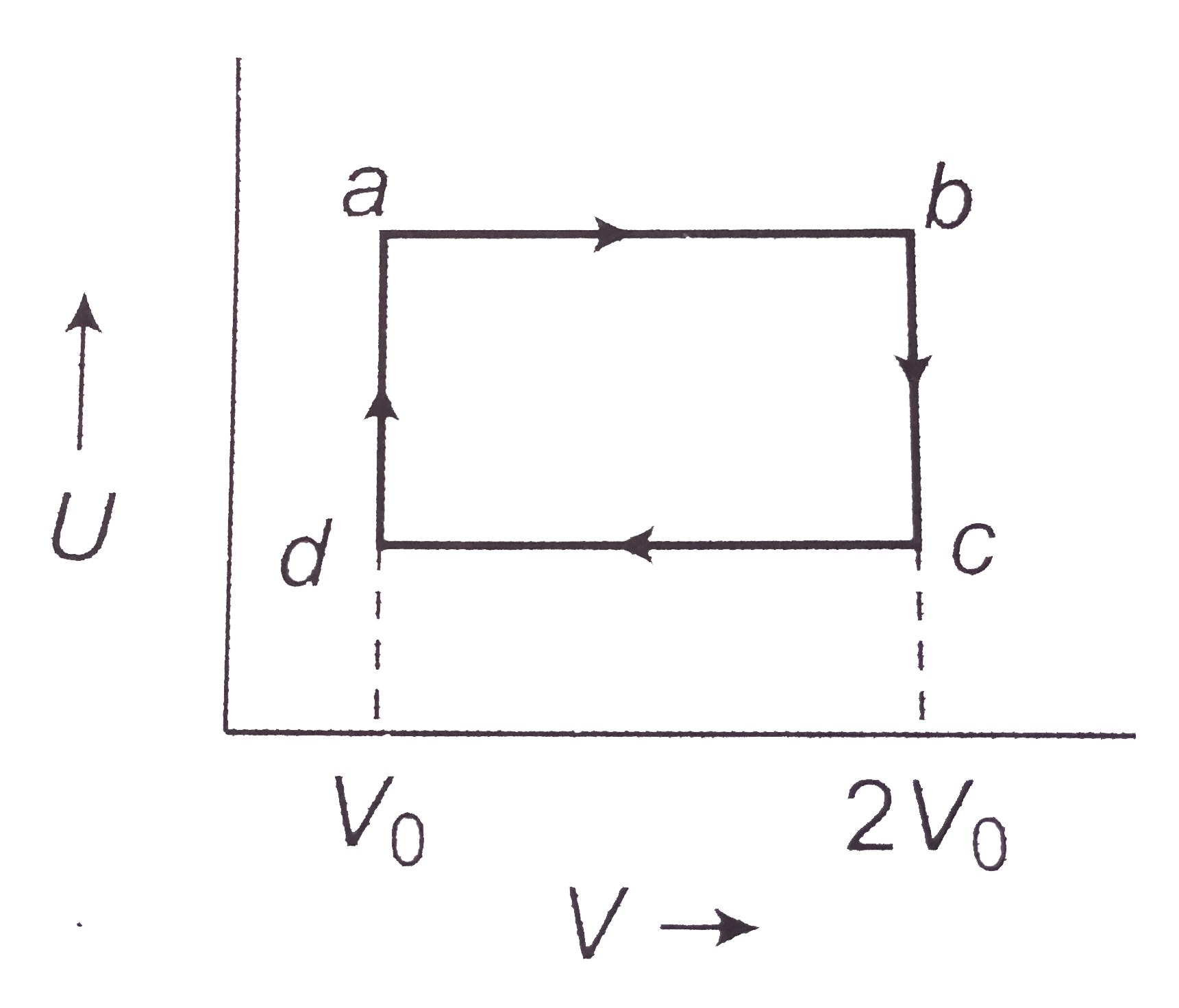 Consider the following cyclic abcda performed on 2 mole of an ideal gas. The temperature of the gas at b and c are 400 k and 300 k respectively. Calculated the heat absorbed by the gas during the process in calories.
Consider the following cyclic abcda performed on 2 mole of an ideal gas. The temperature of the gas at b and c are 400 k and 300 k respectively. Calculated the heat absorbed by the gas during the process in calories.
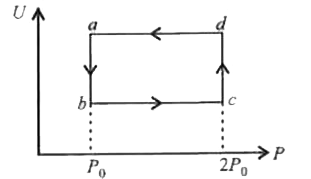
View SolutionThe internal energy (U) of an ideal gas is plotted against volume for a cyclic process ABCDA, as shown in the figure.
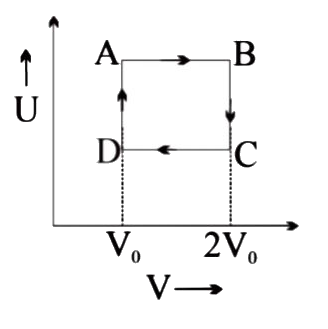
The temperature of the gas at B and C are 500 K and 300 K, respectively. The heat absorbed by the gas (in cal/mol) in this cyclic process, is :
View Solutionconsider the cyclic process ABCA on a sample of 2.0 mol of an ideal gas as shown in the temperature of the gas at A and B are 300 K and 500 k respectively. A total of 1200 J heat is withdrawn from the sample in the process. Find the work done by the gas in part BC. take R=8.3JK−1mol−1

View SolutionOne mole of an ideal monoatomic gas undergoes a cyclic process, as shown in the figure. If the temperature of the gas at state 1 is 300 K and at state 4 is 500 K, then heat exchanged during process 2→3, is

View SolutionOne mole of ideal gas undergoes a cyclic process ACBA as shown in figure. Process AC is adiabatic. The temperatures at 1, B and C are 300 K,600 K and 450 K respectively. Choose the correct statement.
View Solution
