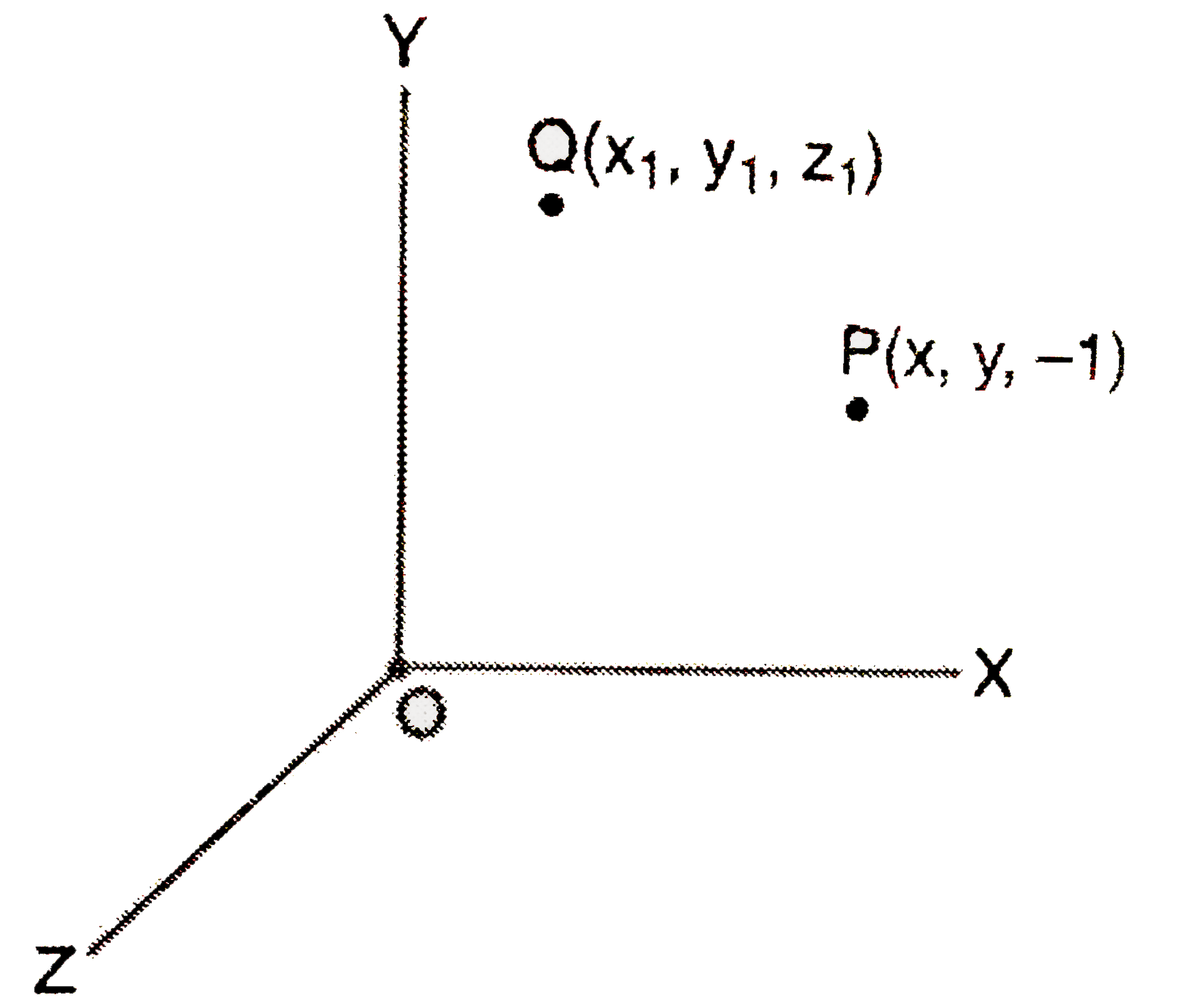
Sppose that a point mass 'm' is moving under a constant force →F=2ˆi−ˆj+ˆk netweon . At some instant , t=0, point P(xm, ym, -1m) [m- metre ] is the instantaneous position of the mass. We know that torque can be expressed as the cross- product of position vector and forces vector, i.e.,
τ=→r×→F . At P, torque can be expessed as τ=(−4ˆj−4ˆk)Nm At some other instant, t=3 sec, the point mass has another instantaneous position Q(x1,y1,z1) such that the displacement vectors between points P and Q and the given force are mutually perpendicular. Also, x-component of torqure at Q is zero and y z-components are equal in magnitude and direction along the negative direction of the respective axes. Using a definite scale, if we construct a parallelogram with the position vectors of Q and the gives force →F as its adjacent sides , area of this parallelogram is 2√2m2 . Area of the given parallelogram , in fact , represents a physical quantity whose magnitude in SI system can be expressed as 5 times the gives are
![Sppose that a point mass 'm' is moving under a constant force vecF = 2hati-hatj + hatk netweon . At some instant , t=0, point P(xm, ym, -1m) [m- metre ] is the instantaneous position of the mass. We know that torque can be expressed as the cross- product of position vector and forces vector, i.e., tau= vecr xx vecF . At P, torque can be expessed as tau= (-4hatj - 4 hatk)Nm At some other instant, t=3 sec, the point mass has another instantaneous position Q(x(1), y(1), z(1)) such that the displacement vectors between points P and Q and the given force are mutually perpendicular. Also, x-component of torqure at Q is zero and y z-components are equal in magnitude and direction along the negative direction of the respective axes. Using a definite scale, if we construct a parallelogram with the position vectors of Q and the gives force vecF as its adjacent sides , area of this parallelogram is 2sqrt(2)m^(2) . Area of the given parallelogram , in fact , represents a physical quantity whose magnitude in SI system can be expressed as 5 times the gives are Answer the following questions. Coordinates of P are :](https://d10lpgp6xz60nq.cloudfront.net/physics_images/GRB_AM_PHY_C03_E01_174_Q01.png)
Answer the following questions.
Coordinates of P are :
The correct Answer is:C
τp=→rpo×→F
=(xi+yj−ˆk)×(2i−j+ˆk)
→τp=(y−1)i−(x+2)j−(x+2y)ˆk
Given →τp=−4ˆj−4ˆk
∴ x=2andy=1
Also, →rPQ=(x1−x)i+(y1−y)j+(z1+1)ˆk
Given →rPQ⊥→F
∴ →rPQ.→F=0
∴[(x1−2)ˆi+(y1−1)ˆj+(z1+1)ˆk].[2i−ˆj+ˆk]=0......(i)
τQ=→rQO×→F=∣∣
∣
∣∣ijkx1y1z12−11∣∣
∣
∣∣
τQ=(y1+z1)ˆi−j(x1−2z1)+k(−x1−2y1)
Given, y1+z1=0 ...........(ii)
And, x1−2z1=x1+2y1 ......(iii)
Also, ∣∣→rQO×→F=10√2
∴(y1+z1)2+(x1−2z1)2+(x1+2y1)2=100×2....(iv)
∴ 2(x1−2z1)2=100×2
∴ x1−2z1=10
∴ →τQ=−10ˆj−10ˆk
W=0, as →rPQ is prependicular to →F
Topper's Solved these Questions
- VECTORSBOOK - GRB PUBLICATIONCHAPTER - VECTORSEXERCISE - PASSAGE -2
3 Videos
- VECTORSBOOK - GRB PUBLICATIONCHAPTER - VECTORSEXERCISE - PASSAGE -3
3 Videos
- VECTORSBOOK - GRB PUBLICATIONCHAPTER - VECTORSEXERCISE - INTERGER ANSWER
9 Videos
- UNITS AND DIMENSIONSBOOK - GRB PUBLICATIONCHAPTER - UNITS AND DIMENSIONSEXERCISE - Link Compression
6 Videos
Similar Questions
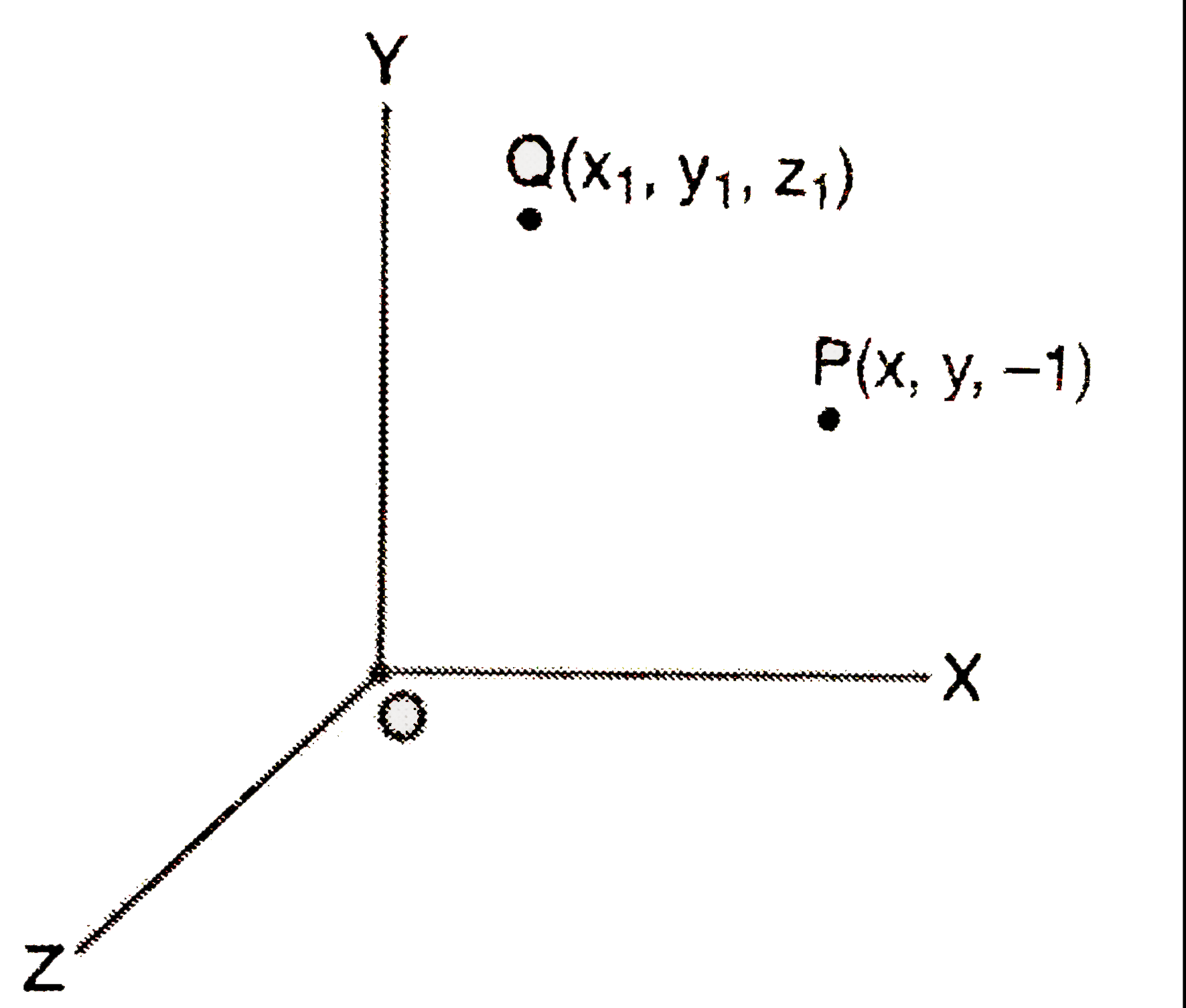
Sppose that a point mass 'm' is moving under a constant force →F=2ˆi−ˆj+ˆk netweon . At some instant , t=0, point P(xm, ym, -1m) [m- metre ] is the instantaneous position of the mass. We know that torque can be expressed as the cross- product of position vector and forces vector, i.e.,
τ=→r×→F . At P, torque can be expessed as τ=(−4ˆj−4ˆk)Nm At some other instant, t=3 sec, the point mass has another instantaneous position Q(x1,y1,z1) such that the displacement vectors between points P and Q and the given force are mutually perpendicular. Also, x-component of torqure at Q is zero and y z-components are equal in magnitude and direction along the negative direction of the respective axes. Using a definite scale, if we construct a parallelogram with the position vectors of Q and the gives force →F as its adjacent sides , area of this parallelogram is 2√2m2 . Area of the given parallelogram , in fact , represents a physical quantity whose magnitude in SI system can be expressed as 5 times the gives are
Answer the following questions.
At Q torque acting on the mass can be expressed as :
View SolutionSppose that a point mass 'm' is moving under a constant force →F=2ˆi−ˆj+ˆk netweon . At some instant , t=0, point P(xm, ym, -1m) [m- metre ] is the instantaneous position of the mass. We know that torque can be expressed as the cross- product of position vector and forces vector, i.e.,
τ=→r×→F . At P, torque can be expessed as τ=(−4ˆj−4ˆk)Nm At some other instant, t=3 sec, the point mass has another instantaneous position Q(x1,y1,z1) such that the displacement vectors between points P and Q and the given force are mutually perpendicular. Also, x-component of torqure at Q is zero and y z-components are equal in magnitude and direction along the negative direction of the respective axes. Using a definite scale, if we construct a parallelogram with the position vectors of Q and the gives force →F as its adjacent sides , area of this parallelogram is 2√2m2 . Area of the given parallelogram , in fact , represents a physical quantity whose magnitude in SI system can be expressed as 5 times the gives are
Answer the following questions.
Work done the for the motion of the points mass from P to Q is :
View SolutionA force F=(2ˆi+3ˆj+4ˆk)N is acting at point P(2m,−3m,6m) find torque of this force about a point O whose position vector is
(2ˆi−5ˆj+3ˆk) m.
View SolutionA force F=(2ˆi+3ˆj+4ˆk)N is acting at point P(2m,−3m,6m) find torque of this force about a point O whose position vector is
(2ˆi−5ˆj+3ˆk) m.
View SolutionA force →F=(ˆi+ˆj−ˆk)N acts at a point P(3 m, 6 m, 9 m). The torque exerted by this force about a point Q(2 m, 7 m, 8 m) is
View SolutionA force →F=(ˆi+ˆj−ˆk)N acts at a point P(3 m, 6 m, 9 m). The torque exerted by this force about a point Q(2 m, 7 m, 8 m) is
View SolutionThe work done in moving an object from origin to a point whose position vector is →r=3ˆi+2ˆj−5ˆk by a force →F=2ˆi−ˆj−ˆk is
View SolutionA force →F=(3ˆi+4ˆj)N is acting on a point mass m=(12)kg at a point A(2m,2m). Find the angular acceleration of the line OA at this instant.
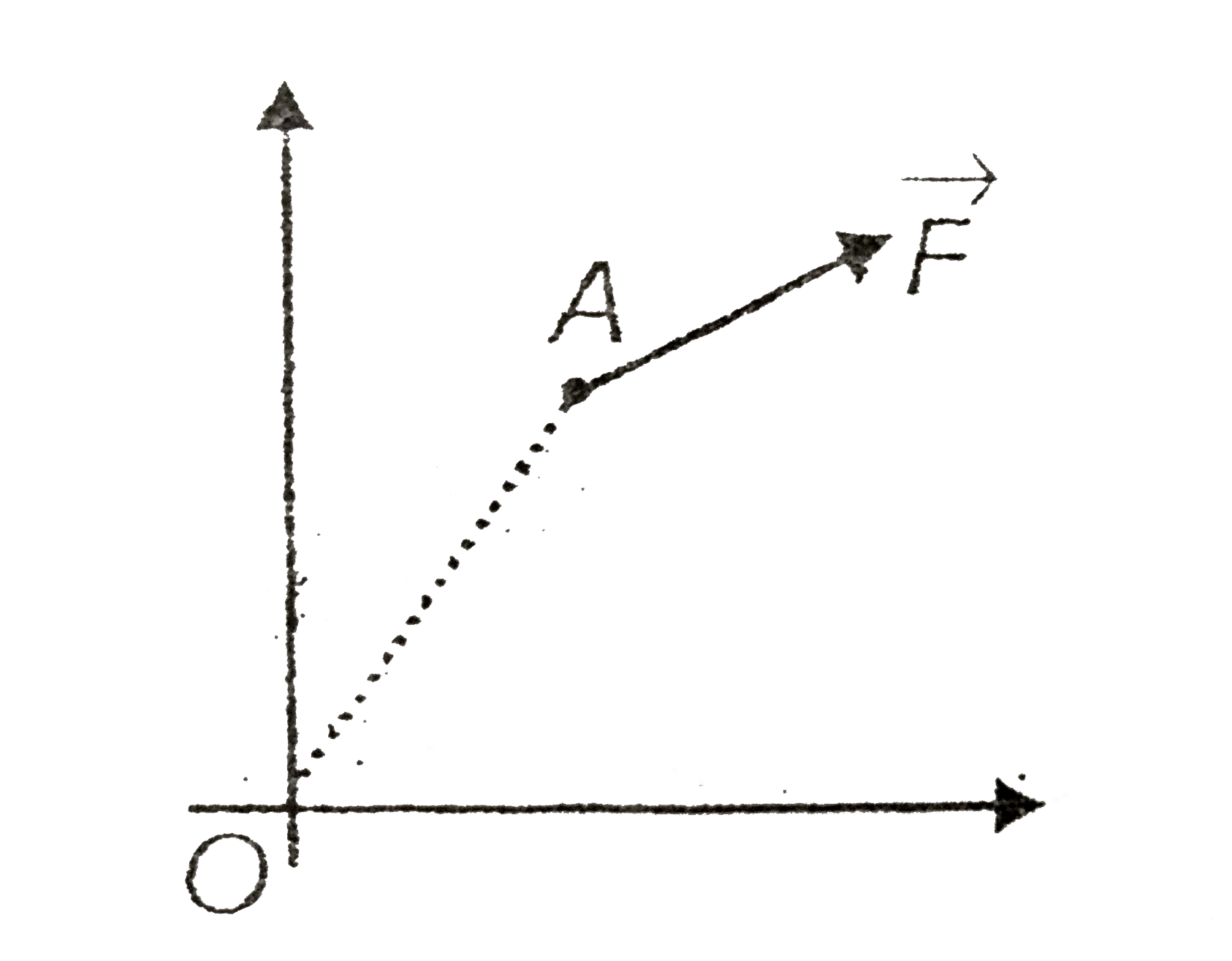
View SolutionA force →F=(3ˆi+4ˆj)N is acting on a point mass m=(12)kg at a point A(2m,2m). Find the angular acceleration of the line OA at this instant.

View SolutionA force →F=2ˆi+ˆj−ˆk acts at point A whose position vector is2ˆi−ˆj. Find the moment of force →F about the origin.
View Solution
