State the expression for linear magnification of a concave mirror in terms of object distance and image distance.
m=h2h1=−vu=−image distanceobject distance
Topper's Solved these Questions
- REFLECTION AND REFRACTIONBOOK - PRADEEPCHAPTER - REFLECTION AND REFRACTIONEXERCISE - Higher Order Thinking Skills Q/A
6 Videos
- REFLECTION AND REFRACTIONBOOK - PRADEEPCHAPTER - REFLECTION AND REFRACTIONEXERCISE - Value based Q/A
4 Videos
- REFLECTION AND REFRACTIONBOOK - PRADEEPCHAPTER - REFLECTION AND REFRACTIONEXERCISE - long Q/A
7 Videos
- MAGNETIC EFFECTS OF ELECTRIC CURRENTBOOK - PRADEEPCHAPTER - MAGNETIC EFFECTS OF ELECTRIC CURRENTEXERCISE - Mock Test
21 Videos
- SOURCES OF ENERGYBOOK - PRADEEPCHAPTER - SOURCES OF ENERGYEXERCISE - Mock Test
20 Videos
Similar Questions
Knowledge Check
The linear magnification of a concave mirror is.
Aalways positiveBalways negativeCsometimes positive and sometimes negativeDcannot predictThe linear magnification of a concave mirror is.
Aalways positiveBalways negativeCpositive or negative depending upon the position of the object.Dcannot sayImage of an object in a concave mirror is.
Aalways realBalways virtualCalways erectDreal or virtual depending on position of object.
Can you change linear magnification of a spherical mirror by changing the object distance from the mirror?
View SolutionThe linear magnification of a convex mirror of focal length 15 cm is 13. What is the distance of the object from the focus of the mirror ?
View SolutionFollowing figure illustrates the ray diagram for the formation of image by a concave mirror. The position of the object is beyond the centre of curvature of the concave mirror. On the basis of given diagram .
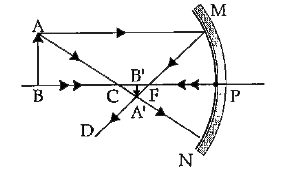
If the focal length of the concave mirror is 10 cm, the image formed will be at a distance ____
View SolutionMagnification produced by a convex mirror is 13, then distance of the object from mirror is
View SolutionAn object is placed at the center of curvature of a concave mirror. The distance between image and pole is:
View Solution