Suppose the bob of the previous problem has a speed of 1.4 m/s when the string makes an angle of 0.20 radian with the vertical. Find the tension at this instant. You can use cosθ=1−θ22andsinθ=θ for small theta.Solution in Tamil
The correct Answer is:A
Bob has a veloicyt 1.4msec, when the string makes and angle of 0.2 radian,
g0.1kg,m=100
r=1m,v=1.4msec
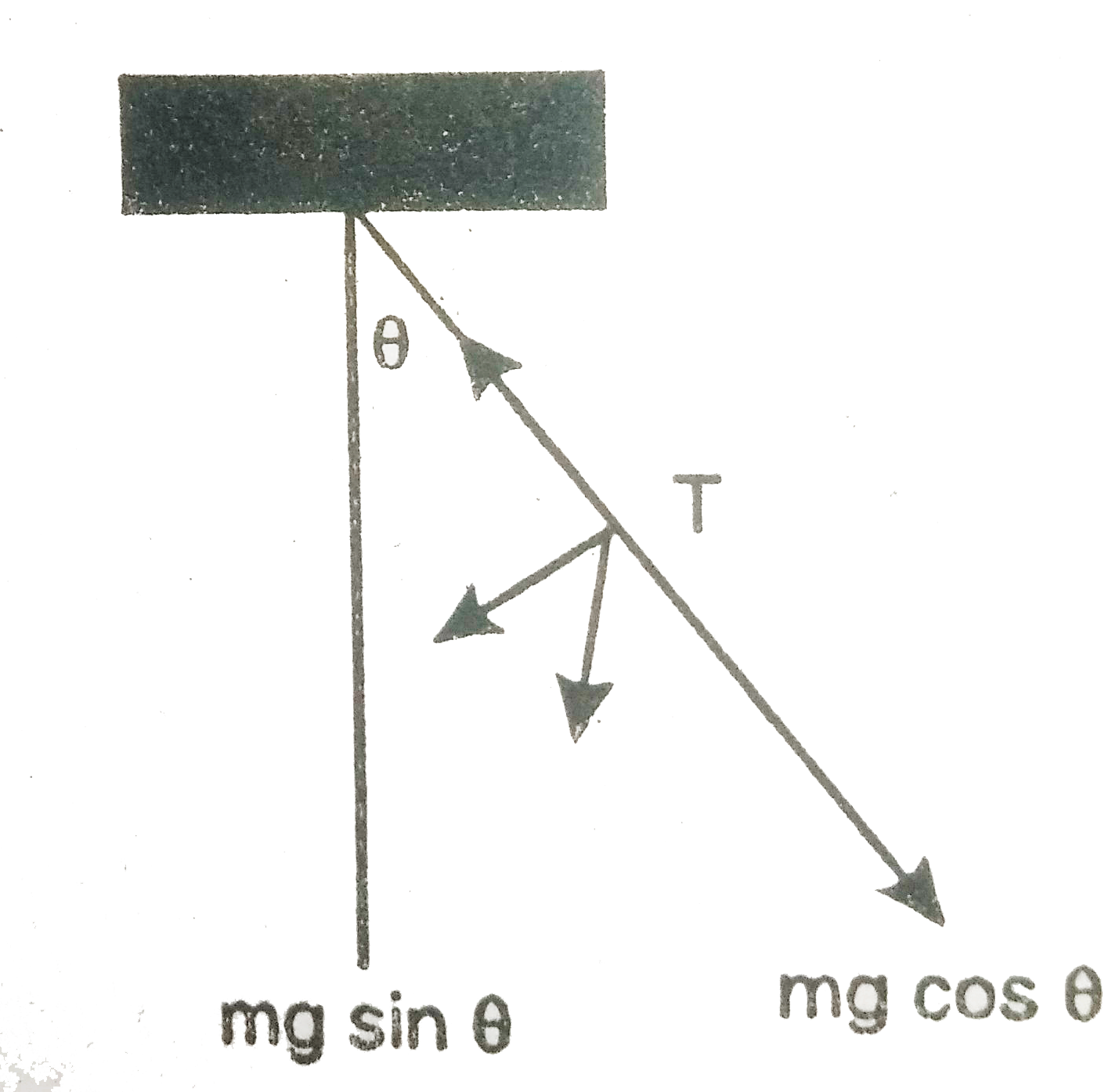
From the diagram,
T−mgcosθ=mv2R
→T=mv2r+mgcosθ
Given cosθ=1θ2/2 for small theta<br>:. T=(0.1xx(1.4)^2)/1+(0.1)xx9.8(1-theta^2/2)<br>=0.196+0.98xx(1-2^2/2)<br>=0.196+0.98xx(0.98)<br>0.196+0.9604<br>=1.156N~~1.16N
Topper's Solved these Questions
- CIRCULAR MOTIONBook:HC VERMAChapter:CIRCULAR MOTIONExercise:Objective -2
Explore 7 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBook:HC VERMAChapter:CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONExercise:Exercises
Explore 64 Videos
- FLUID MECHANICSBook:HC VERMAChapter:FLUID MECHANICSExercise:All Questions
Explore 96 Videos
Similar Questions
An object follows a curved path. The following quantities may remain constant during the motion
View SolutionThree particles of masses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
View SolutionWater is filled in a flask up to a height of 20 cm. The bottom of the flask is circular with radius 10 cm. If the atmospheric pressure is 1.01×105 Pa, find the force exerted by the water on the bottom. Take g=10ms−2 and density of water =1000 kgm^-3.
View SolutionShow that cosθ [cosθsinθ−sinθcosθ]+sinθ [sinθ−cosθcosθsinθ]=1
View SolutionA simple pendulum is constructed by attaching a bob of mas m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View SolutionA 8kg stone tied at the end of a string 1 metre long is whirled in a vertical circle. At the instant when the string makes an angle theta with the vertical, the speed of the stone is 4ms−1 and the tension in the thread is 104N. Then theta is.
View SolutionA pendulum bob of mass m and length L is released from angle theta with the vertical. Find (a) the speed of the bob at the bottom of the swing and (b) tension in the string at that time
View Solutionमान ले कि ऊपर के प्रश्न 14 में जब धागा ऊर्ध्वाधर से 0.20 रेडियन का कोण बनाता है तो गोली की चाल 1.4m/s होती है। इस समय धागे में तनाव बताएँ। आप cosθ के मान को 1−θ22 तथा sinθ के मान को θ ले सकते है।
View Solutionthe bob of simple pendulum is relasesd when string makes an angle theta with the vertical . If m be the mass of the bob then find the tension when the bob is at the bottiommost position .
View SolutionFind d2ydx2 at θ=π2 when :
x=a(θ+sinθ),y=a(1−cosθ)
View SolutionFind d2ydx2 at θ=π2 when :
x=a(θ−sinθ),y=a(1+cosθ)
View SolutionFind d2ydx2 at θ=π2 when :
x=a(1−cosθ),y=a(θ+sinθ)
View SolutionFind d2ydx2 at θ=π2 when :
x=a(θ−sinθ),y=a(1−cosθ)
View SolutionIf sinθ−cosθ=12,then find the value of sinθ+cosθ
View SolutionA simple pendulum is constructed by attaching a bob of mass m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View SolutionIf u =-f''(theta) sintheta + f'(theta) costheta and v = f''(theta) costheta + f'(theta) sintheta , then int[((du)/(d theta))^2+((dv)/(d theta))^2]^(1/2)d theta is equal to
View Solution
