The acceleration of an athlete running at 36 kmph when neotiating a turn of radius 25 m is
1m/s2
2m/s2
4m/s2
8m/s2
The correct Answer is:D
v=45×518=252
ω=vr=2520.5=25rad/s
Topper's Solved these Questions
Similar Questions
The acceleration of an athlete running at 36 kmph when negotiating a turn of radius 25 m is
View SolutionFigure shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5 m at a given instant of time. At this instant, Find: (i) the radial acceleration, (ii) the speed of the particle and (iii) its tangential acceleration.
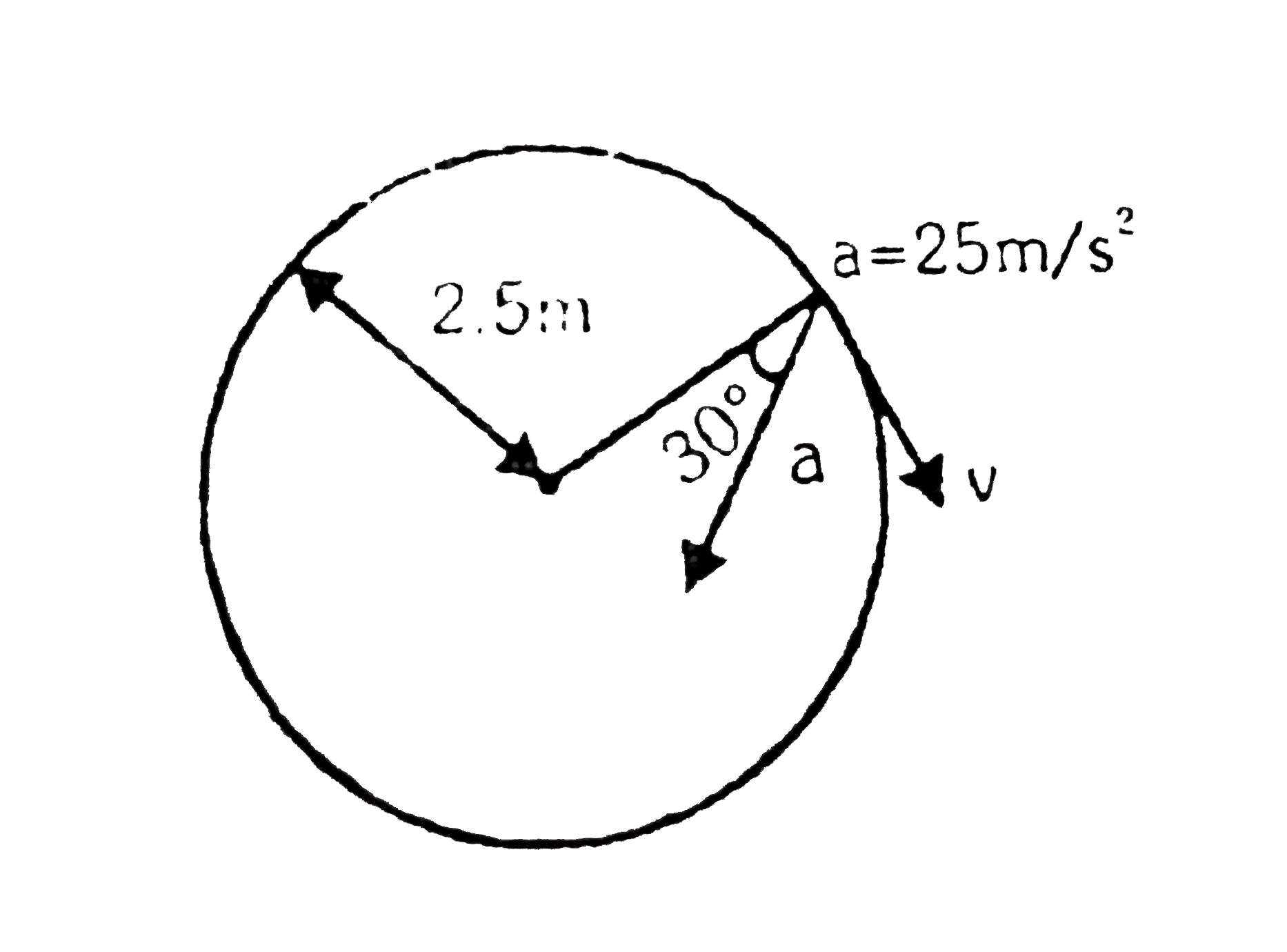
View Solution
Knowledge Check
In the given figure, a=15m/s2 represents the total acceleration of a particle moving in the clockwise dirction in a circle of radius R=2.5m at a given instant of time. The speed of the particle is
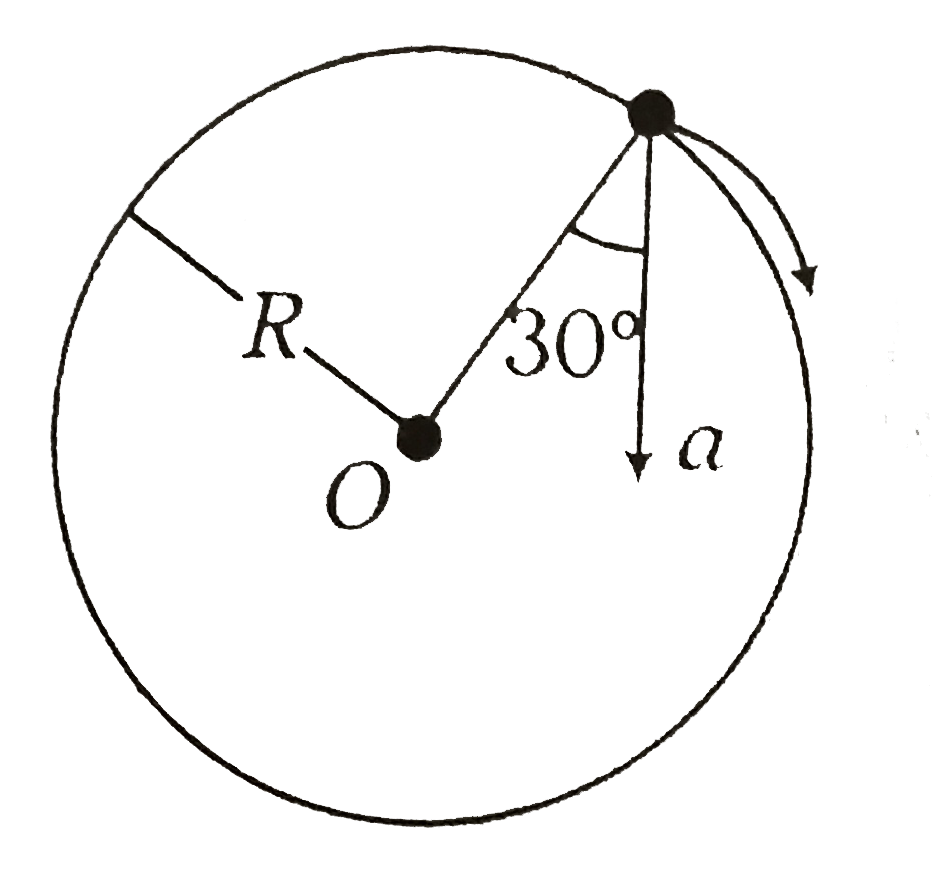 A5.7m/sB6.2m/sC4.5m/sD5.0m/s
A5.7m/sB6.2m/sC4.5m/sD5.0m/sA superfast Duronto express running at 90 kmph overtakes a bike running at 36 kmph in 25 seconds . What is the length of the train in meters ?
A375 mB225 mC275 mD325 mThe centripetal force required by a 1000 kg car that takes a turn of radius 50 m at a speed of 36 kmph is
A1000 NB3500NC1600 ND2000N
Calculate the magnitude of linear acceleration of a particle moving in a circle of radius 0.5 m at the instant when its angular velocity is 2.5 rad s–1 and its angular acceleration is 6rads−2.
View SolutionA 220-m long train is running with a speed of 59 kmph. In what time will it pass a man who is running at 7 kmph in the direction opposite to that in which the train is going?
View SolutionThe centripetal force required by a 1000 kg car that takes a turn of radius 50m at a speed of 36kmph is .
View SolutionA car is travelling at 36 kmph on a road . The maximum turning radius of the car is 20 m ans g=10m/s2 .Then the coefficient of friction between its tyres and the road is
View SolutionTwo trains of equal length are running on parallel lines in the same direction at 46 km/h and 36 km/h respectively. The faster train passes the slower train in 36 seconds. The length of each train is
View Solution
