The deBroglie wavelength of a particle of kinetic energy K is λ. What would be the wavelength of the particle, if its kinetic energy were K4?
λ
2λ
λ2
4λ
The correct Answer is:B
To solve the problem regarding the de Broglie wavelength of a particle with different kinetic energies, we can follow these steps:
1. Understand the de Broglie Wavelength Formula:
The de Broglie wavelength (λ) of a particle can be expressed in terms of its momentum (p):
λ=hp
where h is Planck's constant.
2. Relate Momentum to Kinetic Energy:
The momentum of a particle can also be expressed in terms of its kinetic energy (K):
p=√2mK
where m is the mass of the particle.
3. Substitute Momentum into the Wavelength Formula:
By substituting the expression for momentum into the de Broglie wavelength formula, we get:
λ=h√2mK
4. Determine the New Wavelength for Kinetic Energy K4:
If the kinetic energy is changed to K′=K4, we can find the new wavelength (λ′):
λ′=h√2mK′
Substituting K′:
λ′=h√2m⋅K4=h√2mK4=h√mK2
5. Relate the New Wavelength to the Old Wavelength:
Now, we can express λ′ in terms of λ:
λ′=h√mK2=h√2√mK=√2⋅h√mK=√2⋅λ
6. Final Calculation:
Since we have λ′=√2⋅λ and we need to find the relationship when K′=K4:
λ′=2⋅λ
Thus, the new de Broglie wavelength when the kinetic energy is K4 is:
λ′=2λ
Conclusion:
The wavelength of the particle when its kinetic energy is K4 is 2λ.
---
The de Broglie wavelength (λ) of a particle can be expressed in terms of its momentum (p):
λ=hp
where h is Planck's constant.
The momentum of a particle can also be expressed in terms of its kinetic energy (K):
p=√2mK
where m is the mass of the particle.
By substituting the expression for momentum into the de Broglie wavelength formula, we get:
λ=h√2mK
If the kinetic energy is changed to K′=K4, we can find the new wavelength (λ′):
λ′=h√2mK′
Substituting K′:
λ′=h√2m⋅K4=h√2mK4=h√mK2
Now, we can express λ′ in terms of λ:
λ′=h√mK2=h√2√mK=√2⋅h√mK=√2⋅λ
Since we have λ′=√2⋅λ and we need to find the relationship when K′=K4:
λ′=2⋅λ
λ′=2λ
The wavelength of the particle when its kinetic energy is K4 is 2λ.
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - DAVISSON AND GERMER EXPERIMENT3videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - HIGHER ORDER THINKING SKILLS8videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - PARTICLE NATURE OF LIGHT : THE PHOTON19videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Assertion And Reason15videos
CURRENT ELECTRICITY
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Assertion And Reason15videos
ELECTRIC CHARGES AND FIELDS
Similar Questions
Knowledge Check
- Question 1 - Select One
Which of these particles having the same kinetic energy has the largest de Broglie wavelength ?
AElectronBAlpha particleCProtonDNeutron
Choose the correct alternatives
A. Ratio of de-Broglie wavelengths of proton and α-particle of same kinetic energy is 2L1
B. if neutron, α-particle and β-particle all are moving with same kinetic energy , β-particle has maximum de-Broglie wavelength
C. de-Broglie hypothesis treats particles as waves
D. de-Broglie hypothesis treats waves as made of particlesView SolutionDe-Broglie wavelengths of the particle increases by 75% then kinetic energy of particle becomes.
View SolutionIf the kinetic energy of a particle is increased by 16 times, the percentage change in the de Broglie wavelength of the particle is
View SolutionMatch the following: (P = momentum of particle, K = kinetic energy of particle)
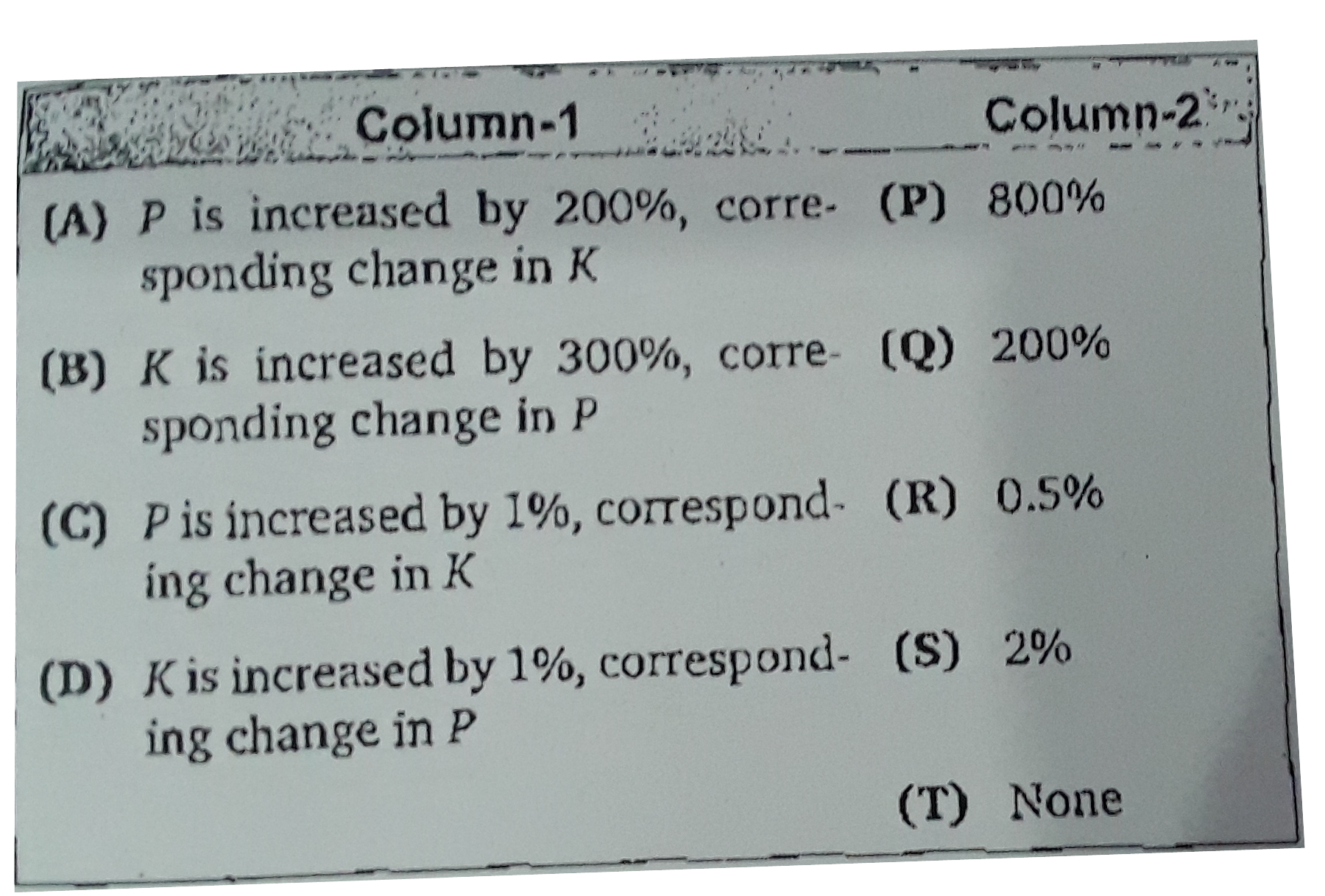 View Solution
View SolutionMatch the following: (P = momentum of particle, K = kinetic energy of particle)
 View Solution
View SolutionTwo particles A and B having mass ratio 4:3 are moving with kinetic energy 1:27 ratio. What would be the ratio of wavelength associated with particle A to that of particle B.
View SolutionTwo particles A and B having mass ratio 4:3 are moving with kinetic energy 1:27 ratio. What would be the ratio of wavelength associated with particle A to that of particle B.
View Solution

