The density of a rod AB increases linearly from A to B its midpoint is O and its centre of mass is at C. four axes pass through A, B, O and C, all perpendicular to the length of the rod. The moment of inertial of the rod about these axes are IA,IB,IO and IC respectively.
IA>IB
IA<IB
IO<IB
IA<IC
The correct Answer is:A
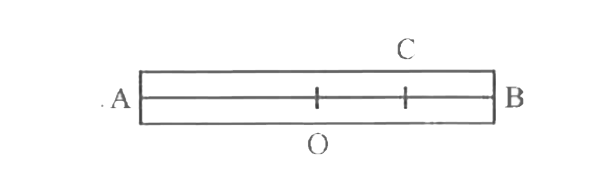
The density of the rod increases lineraly from A to C. As the mass of the section OB is more than that of AO, the centre of gravity (C) of the rod will not be at O. It will be between O and B.
Similarly larger the distances of the various masses from the axis of rotation, larger is the M.I. Hence IA will be maximum and IA>IO>IC>IBandIA>IB is the correct option.
Topper's Solved these Questions
Similar Questions
Find the radius of gyration of a rod of mass 100 g and length 100 cm about an axis passing through its centre and perpendicular to its length.
View SolutionThe moment of inertia of a thin uniform rod about an axis passing through its centre and perpendicular to its length is I0. What is the moment of inertia of the rod about an axis passing through one end and perpendicular to the rod ?
View Solution
Knowledge Check
Moment of inertia of a thin uniform rod about an axis passing through one end perpendicular to its length is I . Then moment of inertia the same rod about the central axis perpendicular to its plane is
AI/4B2IC4ID3 IA rod of length 2 m has a mass of 0.24 kg. Its moment of inerita about an axis passing through its centre and perpendicular to the length of the rod is
A0.04 kg - m2B0.08 kg - m2C0.12 kg - m2D0.02 kg - m2A rod of length 2 m, has a mass of 0.12 kg. Its moment of inertia about an axis passing through its one end and perpendicular to the length of the rod is
A0.16 kg - m2B0.12 kg - m2C0.32 kg - m2D0.08 kg - m2
The M.I. of thin uniform rod of mass 'M' and length 'l' about an axis passing through its centre and perpendicular to its length is
View SolutionThe moment of inertia of a thin uniform rod of mass M and length l about an axis perpendicular to the rod through its centre is I. The moment of inertia of the rod through its end point is
View SolutionA thin rod of length l and mass m is turned at mid-point O at angle of 60°. The moment of inertia of the rod about an axis passing through O and perpendicular to the plane of the rod will be

View SolutionMoment of inertia of a uniform rod of length L and mass M, about an axis passing through L/4 from one end and perpendicular to its length is
View SolutionMoment of inertia of a thin rod of mass m and length l about an axis passing through a point l/4 from one end and perpendicular to the rod is
View Solution
