The displacement covered by a body in time (5.0±0.6)sis(40.0±0.4)m. Calculate the speed of the body . Also determine the percentage error in the speed.
To solve the problem, we need to calculate the speed of the body and then determine the percentage error in that speed.
Step 1: Calculate the speed
The formula for speed v is given by:
v=dt
where:
- d is the displacement,
- t is the time.
Given:
- Displacement d=40.0m with an uncertainty of Δd=0.4m,
- Time t=5.0s with an uncertainty of Δt=0.6s.
Substituting the values into the speed formula:
v=40.0m5.0s=8.0m/s
Step 2: Calculate the percentage error in speed
The percentage error in speed can be calculated using the formula:
Percentage Error=(Δvv)×100
To find Δv, we use the formula for the propagation of uncertainty for division:
Δvv=Δdd+Δtt
Where:
- Δd=0.4m
- d=40.0m
- Δt=0.6s
- t=5.0s
Calculating each term:
1. Calculate Δdd:
Δdd=0.4m40.0m=0.01
2. Calculate Δtt:
Δtt=0.6s5.0s=0.12
3. Combine the uncertainties:
Δvv=0.01+0.12=0.13
4. Now, calculate the percentage error:
Percentage Error=0.13×100=13%
Final Answers
- Speed of the body: 8.0m/s
- Percentage error in speed: 13%
The formula for speed v is given by:
- d is the displacement,
- t is the time.
- Displacement d=40.0m with an uncertainty of Δd=0.4m,
- Time t=5.0s with an uncertainty of Δt=0.6s.
The percentage error in speed can be calculated using the formula:
- Δd=0.4m
- d=40.0m
- Δt=0.6s
- t=5.0s
- Speed of the body: 8.0m/s
- Percentage error in speed: 13%
Topper's Solved these Questions
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Exercise 1.1
16 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Exercise 1.2
6 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
The distance covered by a body in time (30.0±0.4)m is (6.0±0.6)s. Calculate the speed of the body. The percentage error in the speed is
View SolutionA body travels uniformly a distance of (13.8±0.2)m in a time (4.0±0.3)s. Find the velocity of the body within error limits and the percentage error.
View Solution
Knowledge Check
A body travels uniformly a distance of (13.8±0.2)m in a time (4.0±0.3)s. Find the velocity of the body within error limits and the percentage error.
A(3.5±0.6)ms−1B(3.5±0.3)ms−1C(6.1±0.6)ms−1D(6.1±0.3)ms−1
A body travels uniformly a distance of (13.8±0.2)m in a time (4.0±0.3)s. Find the velocity of the body within error limits and the percentage error.
View SolutionA moving body of 40 kg has 180 J of KE. Calculate the speed.
View SolutionA body travels uniformly a distance of 13.8±0.2m in time 4.0±0.3s. The velocity of the body within error limits is?
The percentage errors in the above problem is?
View SolutionA body travels uniformly a distance of (20.0±0.2)m in time (4.0±0.04)s. The velocity of the body is
View SolutionThe displacement of a body at any time t after starting is given by s=15t−0.4t2. Find the time when the velocity of the body will be 7ms−1.
View SolutionFig. 2.38 shows the displacement of a body at different times.
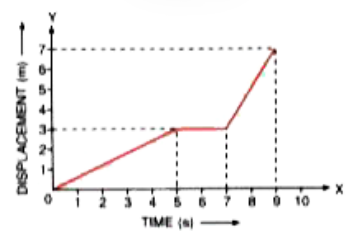
(a) Calculate the velocity of the body as it moves for time interval (i) 0 to 5 s,
(ii) 5 s to 7 S and
(iii) 7 s to 9 s.
(b) Calculate the average velocity during the time interval 5 s to 9 s. [Hint : From 5 s to 9 s, displacement = 7 m - 3 m = 4 m ]
View SolutionAb object covers (16.0±0.4) m distance in (4.0±0.2) s. Find out the second.
View Solution
