The elastic limit of an elavator cable is 2×109N/m2. The maximum upward acceleration that an elavator of mass 2×103kg can have when supported by a cable whose cross sectional area is 10−4m2, provided the stres in cable would not exceed half to the elastic limit would be
10ms−2
50ms−2
40ms−2
Not possible to move up
The correct Answer is:C
From the free body diagram of the elevator
T−mg=ma
T=m(g+a)
Stress is cable is α=TA=m(g+a)a 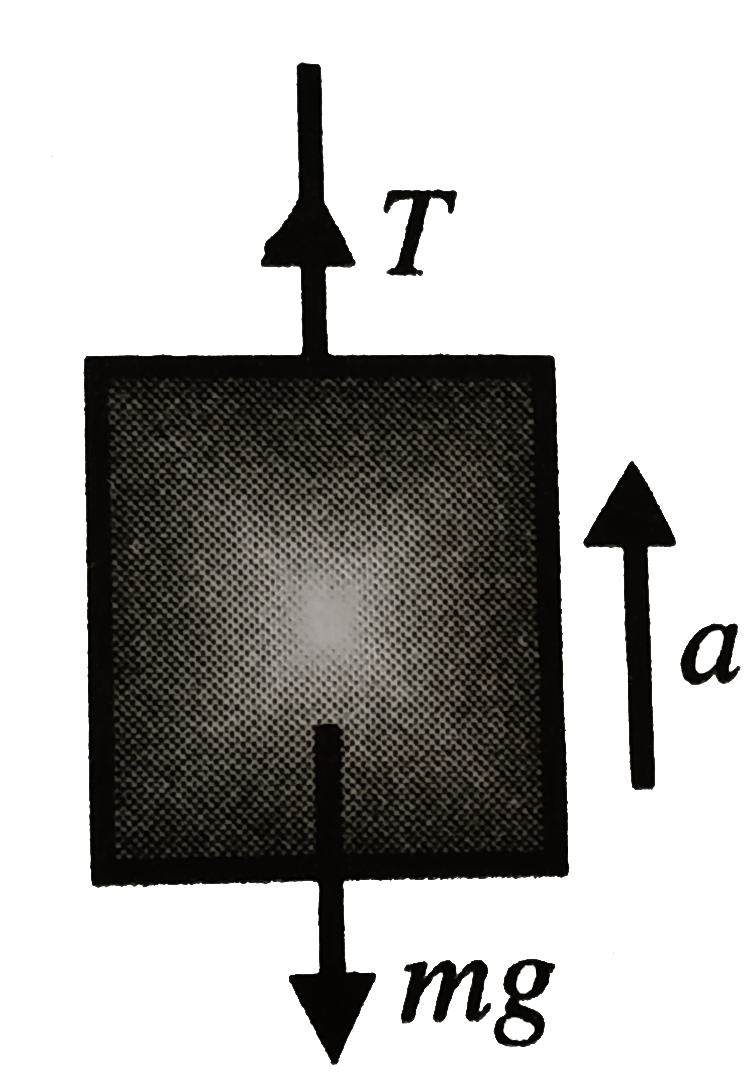
From the given condition α≤αmax2
m(g+a)A≤σmax2
g+a≤2×1092×10−42×103=50
g+a≤2×1092×10−42×10−3=50
Topper's Solved these Questions
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Multiple Correct
17 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Assertion- Reasoning
13 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Subjective
16 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
Similar Questions
The elastic limit of a steel cable is 3.0×108N/m2 and the cross-section area is 4cm2. Find the maximum upward acceleration that can be given to a 900kg elevator supported by the cable if the stress is not to exceed one - third of the elastic limit.
View SolutionA steel wire with cross-section 3 cm2 has elastic limit 2.4 × 108 Nm−2. The maximum upward acceleration that can be given to a 1200 kg elevator supported by this cable if the stress is not to exceed one-third of the elastic limit (take g =10m/ s2) is
View Solution
Knowledge Check
The elastic limit of an elevator cable is 2×109N/m2. The maximum upward acceleration that an elevator of amss 2×103kg can have been supported by a cable would not exceed half of the elastic limit would be
A10m/s2B50m/s2C40m/s2DNot possible to move upA steel wire of cross-sectional area 4 cm2 has elastic limit of 2.2×108N/m2. The maximum upward acceleration that can be given to a 1000 kg elevator supported by this steel wire if the stress is to exceed one-fourth of the elastic limit is [Take, g = 10 m/s2 ]
A10 m/s2B9m/s2C11m/s2D12m/s2An elevator weighing 6000 kg is pulled upward by a cable with an acceleration of 5ms−2 . Taking g to be 10ms−2 , then the tension in the cable is
A6000 NB9000 NC60000 ND90000 N
The stress for elastic limit of a material is 3.5×108Nm2 .The minimum diameter of a rod made of this material which can support 500N load without exceeding elastic limit will be
View SolutionIf the elastic limit of copper is 1.5×108N/m2,determine the minimum diameter a copper wire can have under a load of10.0kgfind , if its elastic limit is not to be exceeded.
View SolutionThe cross-sectional area of water pipe entering the basement is 4 x 10−4m2. The pressure at this point is 3 x 105Nm−2 and the speed of water is 2 ms−1. This pipe tapers to a cross-sectional area of 2 x 10−4m2 when it reaches the second floor 8 m above. Calculate the speed and pressure at the 2nd floor
View Solution[" The stress for elastic limit of a "],[" material is "3.5times10^(8)N/m^(2)" .The "],[" minimum diameter of a rod made of "],[" this material which can support "500N],[" load without exceeding elastic limit "],[" will be "]
View SolutionThe mass of an electron at rest is 9.1×10−31 kg. the mass of an electron, when it is moving with a speed of 2.4×108 m/s is (c=3×108m/s)
View Solution
