The energy of a silver atom with a vacancy in K shell is 25.31 keV, in L shell is 3.56 keV and in M shell is 0.530 keV higher than the energy of the atom with no vacancy. Find the frequency of Kα, Kβ and Lα X-rays of silver.
To find the frequencies of K_alpha, K_beta, and L_alpha X-rays of silver, we will use the energy differences between the shells and Planck's equation, which relates energy and frequency.
1. Identify the Energy Values:
- Energy of the silver atom with a vacancy in the K shell (EK) = 25.31 keV
- Energy of the silver atom with a vacancy in the L shell (EL) = 3.56 keV
- Energy of the silver atom with a vacancy in the M shell (EM) = 0.530 keV
2. Convert Energy from keV to Joules:
- 1 eV = 1.6 × 10^-19 Joules
- Therefore, we convert the energies:
- EK = 25.31 keV = 25.31 × 10^3 eV = 25.31 × 10^3 × 1.6 × 10^-19 J
- EL = 3.56 keV = 3.56 × 10^3 eV = 3.56 × 10^3 × 1.6 × 10^-19 J
- EM = 0.530 keV = 0.530 × 10^3 eV = 0.530 × 10^3 × 1.6 × 10^-19 J
3. Calculate K_alpha Frequency:
- K_alpha X-ray is emitted when an electron transitions from the L shell to the K shell.
- The energy difference for K_alpha is given by:
EKα=EK−EL
- Substitute the values:
EKα=(25.31−3.56)×103×1.6×10−19 J
- Calculate the energy difference:
EKα=21.75×103×1.6×10−19 J
- Now, use Planck's equation E=hν to find the frequency:
νKα=EKαh
- Where h=6.63×10−34 J s.
- Substitute and calculate:
νKα=21.75×103×1.6×10−196.63×10−34
4. Calculate K_beta Frequency:
- K_beta X-ray is emitted when an electron transitions from the M shell to the K shell.
- The energy difference for K_beta is given by:
EKβ=EK−EM
- Substitute the values:
EKβ=(25.31−0.530)×103×1.6×10−19 J
- Calculate the energy difference:
EKβ=24.78×103×1.6×10−19 J
- Use Planck's equation to find the frequency:
νKβ=EKβh
5. Calculate L_alpha Frequency:
- L_alpha X-ray is emitted when an electron transitions from the M shell to the L shell.
- The energy difference for L_alpha is given by:
ELα=EL−EM
- Substitute the values:
ELα=(3.56−0.530)×103×1.6×10−19 J
- Calculate the energy difference:
ELα=3.03×103×1.6×10−19 J
- Use Planck's equation to find the frequency:
νLα=ELαh
Final Frequencies:
- Calculate the frequencies for K_alpha, K_beta, and L_alpha using the above equations.
- Energy of the silver atom with a vacancy in the K shell (EK) = 25.31 keV
- Energy of the silver atom with a vacancy in the L shell (EL) = 3.56 keV
- Energy of the silver atom with a vacancy in the M shell (EM) = 0.530 keV
- 1 eV = 1.6 × 10^-19 Joules
- Therefore, we convert the energies:
- EK = 25.31 keV = 25.31 × 10^3 eV = 25.31 × 10^3 × 1.6 × 10^-19 J
- EL = 3.56 keV = 3.56 × 10^3 eV = 3.56 × 10^3 × 1.6 × 10^-19 J
- EM = 0.530 keV = 0.530 × 10^3 eV = 0.530 × 10^3 × 1.6 × 10^-19 J
- K_alpha X-ray is emitted when an electron transitions from the L shell to the K shell.
- The energy difference for K_alpha is given by:
EKα=EK−EL
- Substitute the values:
EKα=(25.31−3.56)×103×1.6×10−19 J
- Calculate the energy difference:
EKα=21.75×103×1.6×10−19 J
- Now, use Planck's equation E=hν to find the frequency:
νKα=EKαh
- Where h=6.63×10−34 J s.
- Substitute and calculate:
νKα=21.75×103×1.6×10−196.63×10−34
- K_beta X-ray is emitted when an electron transitions from the M shell to the K shell.
- The energy difference for K_beta is given by:
EKβ=EK−EM
- Substitute the values:
EKβ=(25.31−0.530)×103×1.6×10−19 J
- Calculate the energy difference:
EKβ=24.78×103×1.6×10−19 J
- Use Planck's equation to find the frequency:
νKβ=EKβh
- L_alpha X-ray is emitted when an electron transitions from the M shell to the L shell.
- The energy difference for L_alpha is given by:
ELα=EL−EM
- Substitute the values:
ELα=(3.56−0.530)×103×1.6×10−19 J
- Calculate the energy difference:
ELα=3.03×103×1.6×10−19 J
- Use Planck's equation to find the frequency:
νLα=ELαh
- Calculate the frequencies for K_alpha, K_beta, and L_alpha using the above equations.
Topper's Solved these Questions
- X-RaysBOOK - HC VERMA ENGLISHCHAPTER - X-RaysEXERCISE - Ques for Short Ans
10 Videos
- X-RaysBOOK - HC VERMA ENGLISHCHAPTER - X-RaysEXERCISE - Objective 2
8 Videos
- THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTBOOK - HC VERMA ENGLISHCHAPTER - THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTEXERCISE - Short Question
9 Videos
Similar Questions
The energy of an element with a vacancy in K-shell is 35.2 keV, in L-shell is 5.25 keV and in M-shell is 0.55 keV higher than the energy of the atom of with no vacancy. Find the frequency of Kα,KβandLα X-rays of that element.
View SolutionThe energy of a tungsten atom with a vacancy in L small is 11.3KaV. Wavelength of Kα photon for tungsten is 21.3pm. If a potential difference of 62kV is applied across the X-raystube following characterstic X-rays will be produced.
View SolutionThe energy of K-electron in tungsten is -20.0 keV and of an L-electron is -2.0 ke V. Find the wavelength of X-rays emitted when an electron jumps from L - shell to K-shell.
View SolutionWhy 3d sub-shell has higher energy than 4s sub-shell in a muti-electron atom?
View SolutionFigure shows Kα& Kβ X-rays along with continuous X-ray. Find the energy of Lα X-ray. (Use hc = 12420 evÅ)

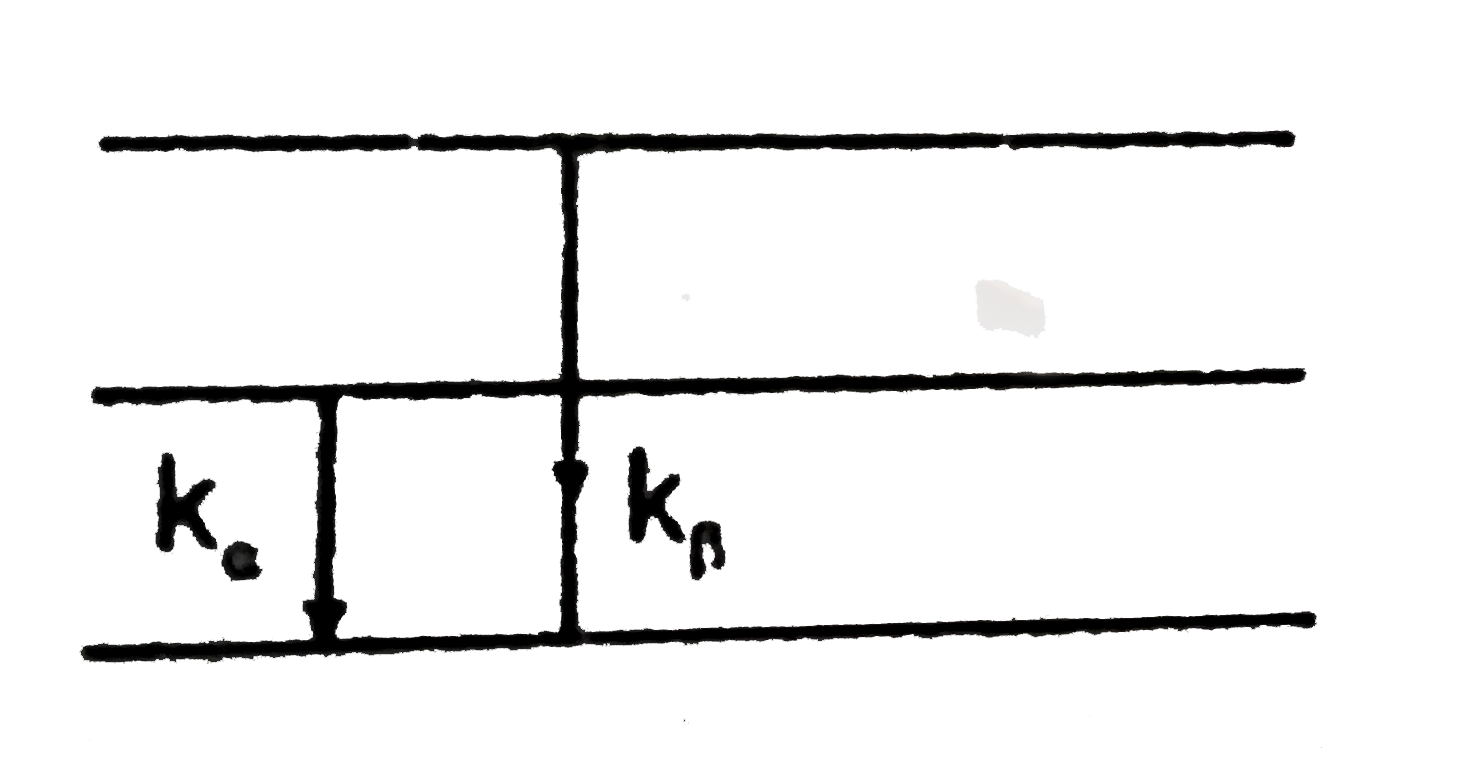
View SolutionIf K and L shells of an atom are full, then what would be the total number of electrons in the atom?
View SolutionThe Ka X-ray of molybdenum has wavelength 71 pm. If the energy of a molybdenum atom with a K electron knocked out is 23:32 keV, what will be the energy of this atom when an L electron is knocked out?
View SolutionThe Kα and Kβ X-rays of molybdenum have wavelengths 0⋅71A and0⋅63A respectively. Find the wavelength of Lα X-ray of molybdenum.
View SolutionCan Lα X-ray of one material have shorter wavelength than Kα X-ray of another?
View SolutionSimplified model of electron energy levels for a cartain atom is shown in figure. The atom is bombarded will fast moving electrons. The impact of one of these electrons can cause the removal of electron from K-level , thus, creating a vacancy in the K-level. This vacancy in K-level is filled by an electron from l-level and the energy reased in this transition can either appear as electromagnetic waves or may all be used knock out an electron from M-level of the atom.
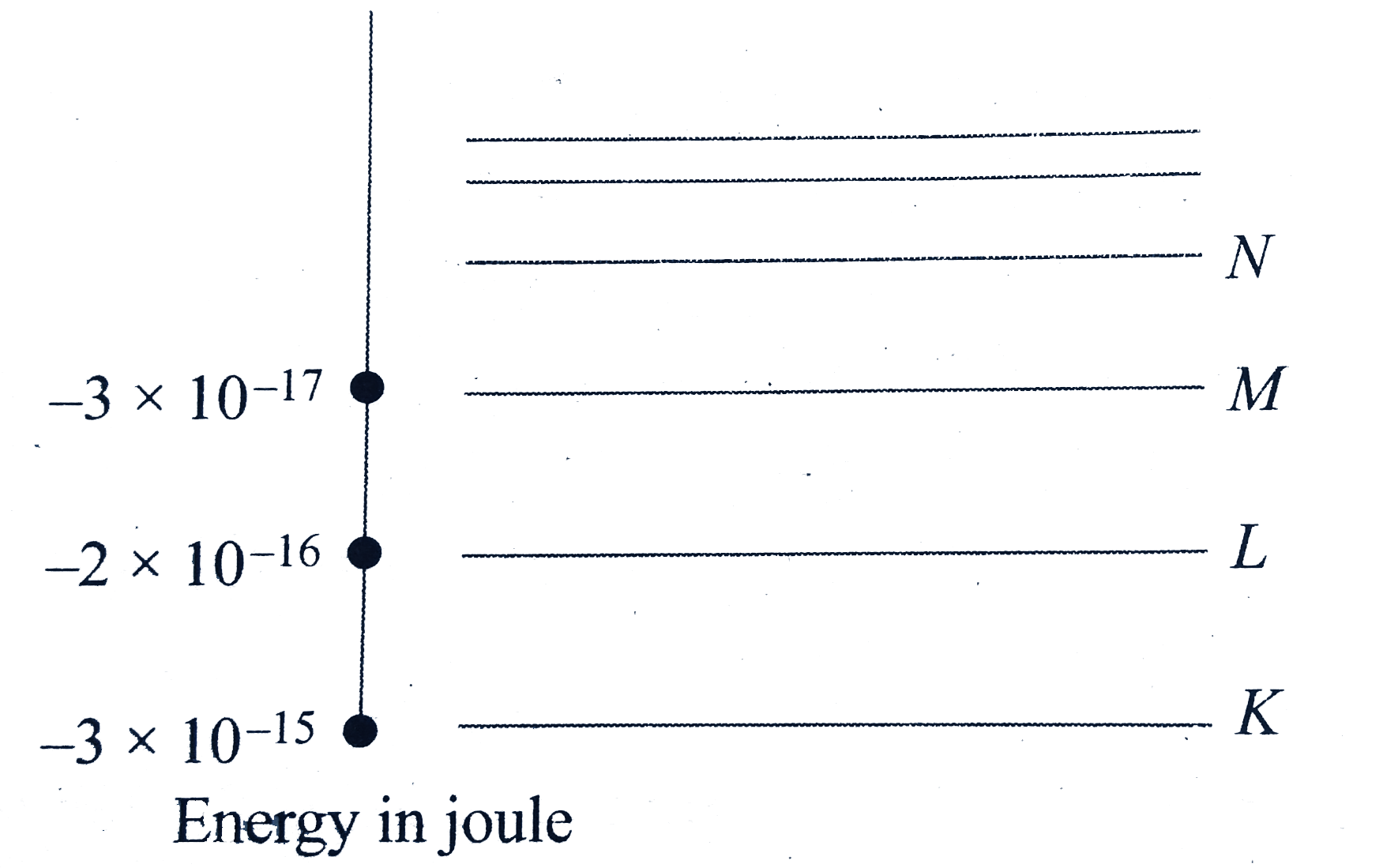
Based on above information, answer the following question:
Wavelength of the electromagnetic waves emitted due to transition from L to K-level is
View Solution
