The equation of an alternating current is I=20sin300πt. Calculate the frequency and r.m.s value of current.
To solve the problem, we will follow these steps:
Step 1: Identify the given equation
The equation of the alternating current is given as:
I=20sin(300πt)
Step 2: Compare with the standard form
The standard form of the equation for alternating current is:
I=I0sin(ωt)
where I0 is the peak current and ω is the angular frequency.
By comparing the two equations, we can identify:
- I0=20 A (peak current)
- ω=300π rad/s
Step 3: Calculate the r.m.s value of the current
The root mean square (r.m.s) value of the current is given by the formula:
Irms=I0√2
Substituting the value of I0:
Irms=20√2
To simplify:
Irms=20⋅√22=10√2
Now, we can calculate 10√2:
Using √2≈1.414:
Irms=10⋅1.414=14.14A
Step 4: Calculate the frequency
We know that the relationship between angular frequency ω and frequency f is given by:
ω=2πf
From our earlier step, we have:
ω=300π
Setting the two expressions for ω equal:
2πf=300π
Dividing both sides by 2π:
f=300π2π=150Hz
Final Answers
- The r.m.s value of the current is 14.14A.
- The frequency of the current is 150Hz.
---
The equation of the alternating current is given as:
I=20sin(300πt)
The standard form of the equation for alternating current is:
I=I0sin(ωt)
where I0 is the peak current and ω is the angular frequency.
- I0=20 A (peak current)
- ω=300π rad/s
The root mean square (r.m.s) value of the current is given by the formula:
Irms=I0√2
Irms=20√2
Irms=20⋅√22=10√2
Using √2≈1.414:
Irms=10⋅1.414=14.14A
We know that the relationship between angular frequency ω and frequency f is given by:
ω=2πf
ω=300π
2πf=300π
f=300π2π=150Hz
- The r.m.s value of the current is 14.14A.
- The frequency of the current is 150Hz.
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - AAKASH SERIESCHAPTER - ALTERNATING CURRENTEXERCISE - Exercises (Long Answer Questions)
2 Videos
- ALTERNATING CURRENTBOOK - AAKASH SERIESCHAPTER - ALTERNATING CURRENTEXERCISE - Exercies (Short Answer Questions)
4 Videos
- APPENDICES ( REVISION EXERCISE )BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (MAGNETISM AND MATTER )
52 Videos
Similar Questions
The rms value of an alternating current
View SolutionThe frequency of alternating current is .........
View SolutionThe peak value of an alternating current is 10. A. Find its rms value
View SolutionThe equation of an alternating voltage is E=220sin(ωt+π/6) and the equation of the current in the circuit is I=10sin(ωt−π/6). Then the impedance of the circuit is
View SolutionAn alternating current is given by the following equation: I=3√2sin(100πt+π/4). Give the frequency and rms value of the current.
View SolutionIf i=t2,0<t<T then r.m.s. value of current is
View SolutionThe peak value of an alternating current is 5A and its frequency is 60Hz. Find its rms value. How long will the current take to reach the peak value starting from zero?
View SolutionThe peak value of an alternating current is 5A and its frequency is 60Hz. Find its rms value. How long will the current take to reach the peak value starting from zero?
View SolutionVariation of alternating current .l. with time .t. is shown in the graph below.
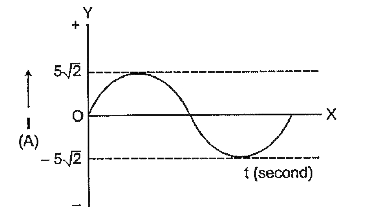
What is ther.m.s.value of this current ?
View SolutionThe peak value of an alternating current is 5A and its frequency is 60Hz. Find its rms value. How long will the cukrgrenQt current IS 5 A and its frequency is 60 Hz. Find its runs value.
View Solution
