The figure, drawn here, shows the geometry of path differences for diffraction by a single slit of width a.
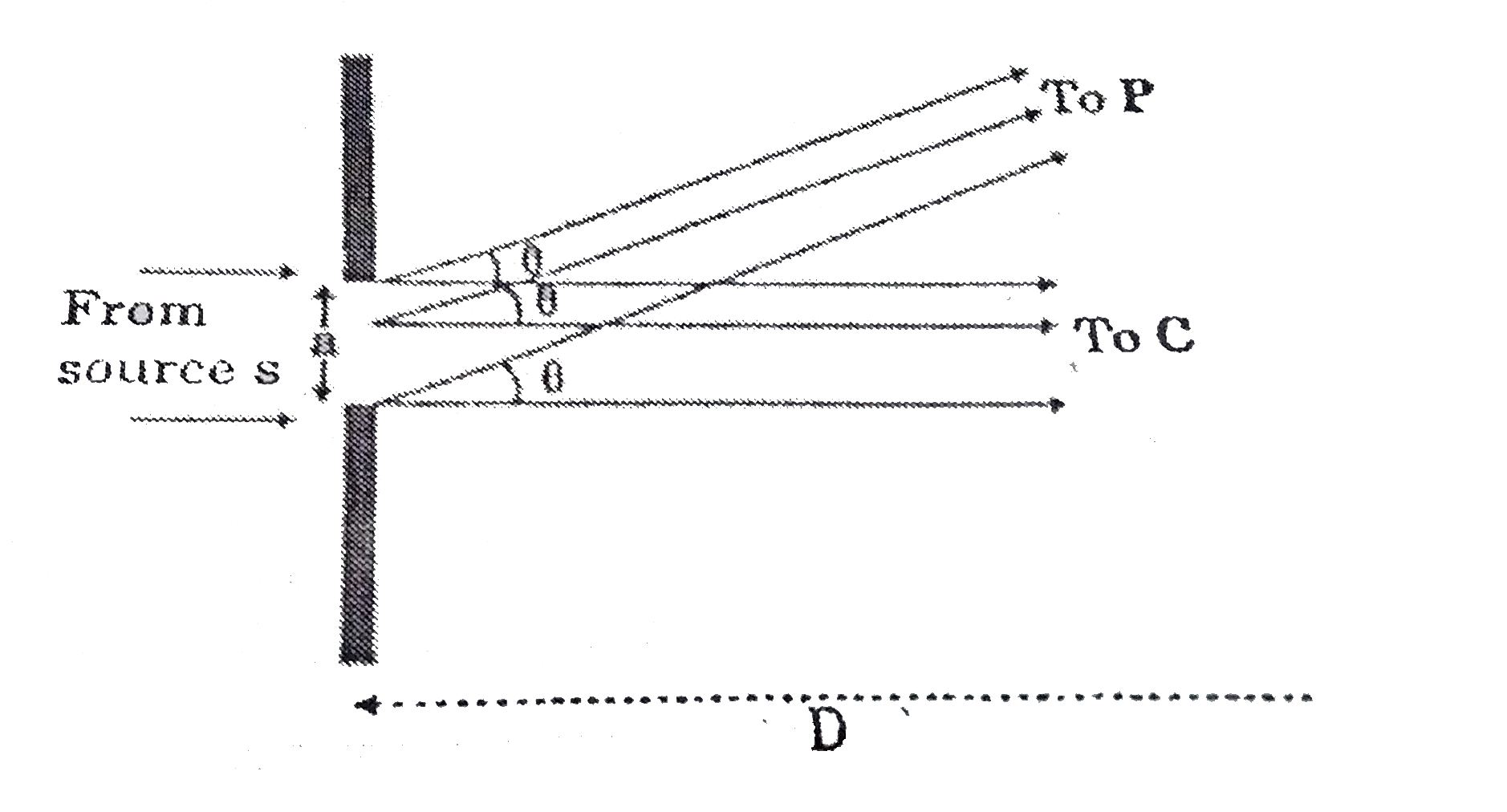
Given appropriate reasoning to explain why the intensity of light is
(i) maximum of the central point C on the screen.
(ii) (nearly) zero for point P on the screen when .
Hence, write an expression for the total linear width of the central maxima on a screen kept at a distance D from the plane of the slit.
Step by step video & image solution for The figure, drawn here, shows the geometry of path differences for diffraction by a single slit of width a. Given appropriate reasoning to explain why the intensity of light is (i) maximum of the central point C on the screen. (ii) (nearly) zero for point P on the screen when theta=lamda//a. Hence, write an expression for the total linear width of the central maxima on a screen kept at a distance D from the plane of the slit. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
In a single slit diffraction of light of wavelength λ by a slit of width e, the size of the central maximum on a screen at a distance b is
View SolutionThe angular width of the central maximum of the diffraction patternn in a single slit (of width a) experiment, with λ as the wavelenth of light, is
View SolutionIn the experiment of diffraction at a single slit, if the slit width is decreased, the width of the central maximum
View SolutionAngular width of central maximum in diffraction at a single slit is……………. .
View SolutionIn diffraction froma a single -slit, the angular width of the centre maxima does not depends on
View SolutionIn single sit diffraction experiment, the width of the central maximum inversely proportional to
View SolutionIn a Young’s double slit experiment, the ratio of the slit’s width is 4 : 1. The ratio of the intensity of maxima to minima, close to the central fringe on the screen, will be:
View SolutionLight of wavelength 5000A0 is incident on a slit. The first minimum of the diffraction pattern is observed to lie at a distance of 5 mm from the central maximum on a screen placed at a distance of 3 m from the slit. Then the width of the slit is
View SolutionA single slit of width d is placed in the path of beam of wavelength λ. The angular width of the principal maximum obtained is-
View SolutionFraunhofer diffraction from a single slit of width 1.24×10−6m is observed with the light of wavelength 6200 Å. Calculate the angular width of the central maximum.
View Solution
