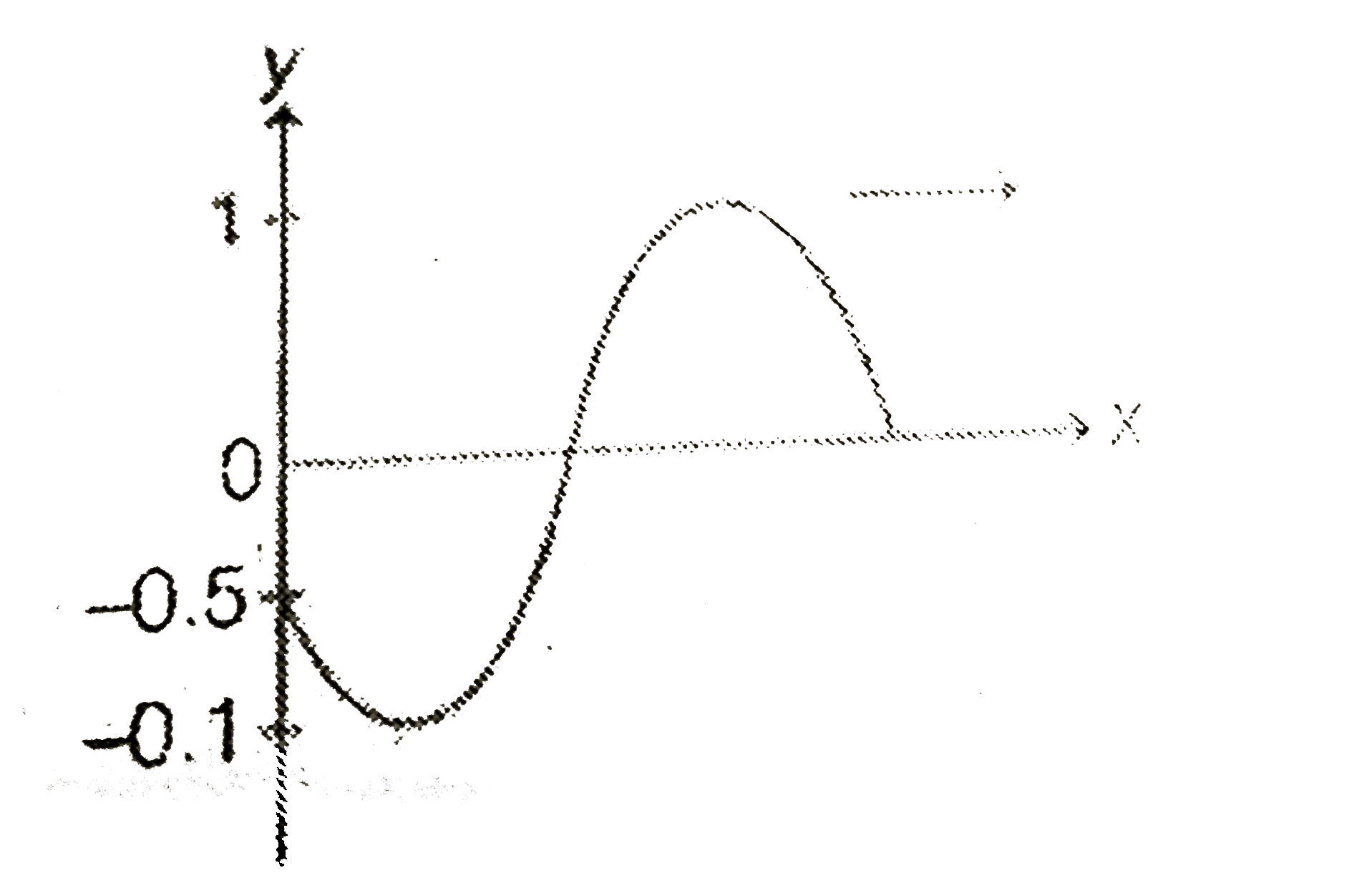
The given figure shows the position of a medium particle at t=0, supporting a simple harmonic wave travelling either along or opposite to the positive x-axis.

(a) write down the equation of the curve.
(b) find the angle θ made by the tangent at point P with the x-axis.
(c ) If the particle at P has a velocity vpm/s, in the negative y-direction, as shown in figure, then determine the speed and direction of the wave.
(d) find the frequency of the wave.
(e) find the displacement equation of the particle at the origin as a function of time.
(f) find the displacement equation of the wave.
(a). From given curve: amplitude of the wave A=10cm and wavelength λ=12cm
y=Asin(kx±ωt+ϕ)=Asin(2πλx±2πTt+ϕ)….(i)
At t=0,y=Asin(kx+ϕ)=10sin(π)6x+ϕ)
At x=0,y=−5cm=10sinϕ
sinϕ=−(1)(2)⇒ϕ=−π6
hence y=0.10(m)sin(π)6x−π6)
(b). tanθ=dydx=0.10×(π)(6)cos(π)6x−π6)
At x=6m
dydxtanθ=π600cos(π−π6)=π60(−cos(π)6)
=−π60.√32=−π√3120
θ=tan−1(−π√3120)
(c ). nParticle velocity
vp=v(dydx)
−vp=−v−π√3120)
v=−120√3πvp=−40√3πvp Along negative x−axis.
(d). wave velocity
v=fλ
⇒f=vλ0=40√3vpπ×12
=10√33πvps−1
(e). hence displacement equation of medium particle at origin at x=0, from eq... (ii)
y=10(cm)sin[20√33vpt−π6]
(f). As wave is travelling towards negative x-directin hence positive sign should be used between kx and ωt in eq. ..(i)
The equation of the wave,
y=0.1(m)sin[π6x(m)+ωt−π6]
here ω=2πf=2π10√33πvp=20√33vp
Hence displacement equation of the wave
y=0.1(m)sin[π6x(m)+20√33v−(p)t−π6]...(ii)
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct
62 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct Answer
1 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Exercise 5.2
23 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
Knowledge Check
The differential equation of a particle executing simple harmonic motion along y-axis is
Ad2ydt2+ω2y=0Bd2ydt2+ω2y2=0Cd2ydt2−ω2y=0Ddydt+ωy=0
in a sine wave ,postive of different particles at time t=0 is shown in figure. The equation for this wave if it is travelling along postive x -axis can beB
View SolutionIf the perpendicular distance of a point P from the X-axis is 5 units and the foot of the perpendicular lies on the negative direction of X-axis then the point P has
View SolutionThe position of a transverse wave travelling in medium along positive x-axis is shown in figure at time t=0. Speed of wave is v=200 m/s
Equation of the wave is (in SI unit)
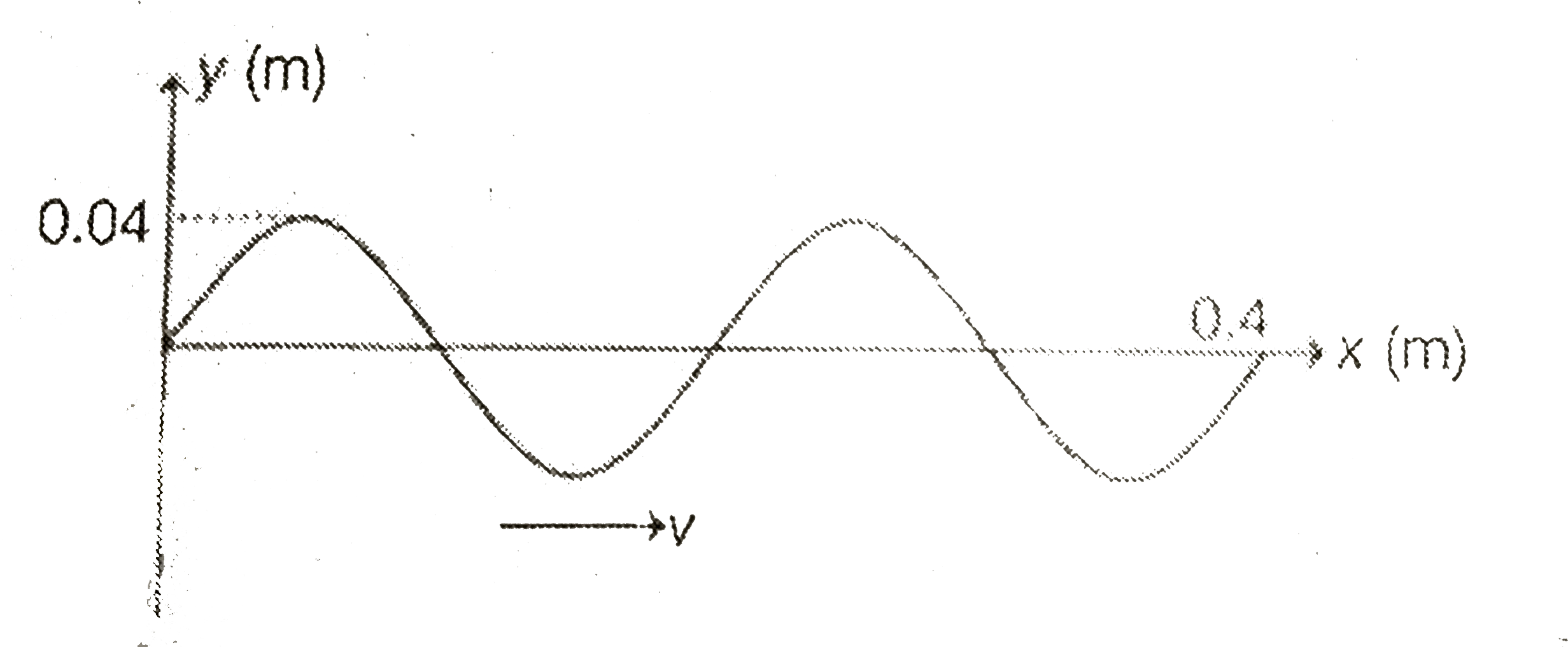
View SolutionThe position of a transverse wave travelling in medium along positive x-axis is shown in figure at time t=0. Speed of wave is v=200 m/s
Frequency of the wave is

View SolutionThe sketch in the figure shows displacement time curve of a sinusoidal wave at x=8m. Taking velocity of wave v=6m/s along positive x−axis, write the equation the wave.
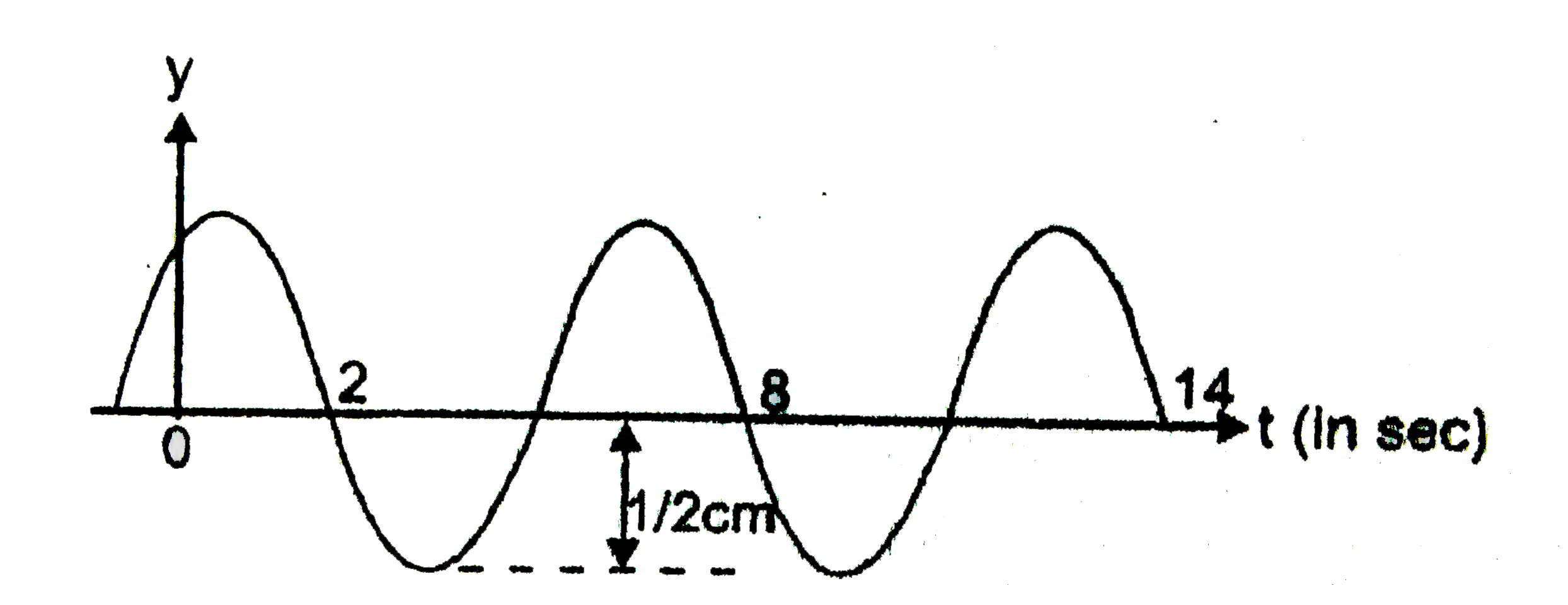
View SolutionA particle moves in the x-y plane with a constant acceleration of 1.5m/s2 in the direction making an angle of 37∘ with the x-axis.
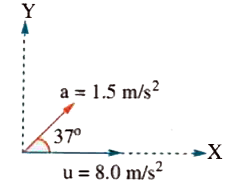
At t=0 the particle is at the origin and its velocity is 8.0m/s along the x- aixs . Find the velocity and the position of the particle at t = 4.0s.
View SolutionThe figure below is a representation of a simple harmonic progressive in the negative X-axis, at a given instant. The direction of the velocity of the particle at stage P in the figure is best represent by the arrow.
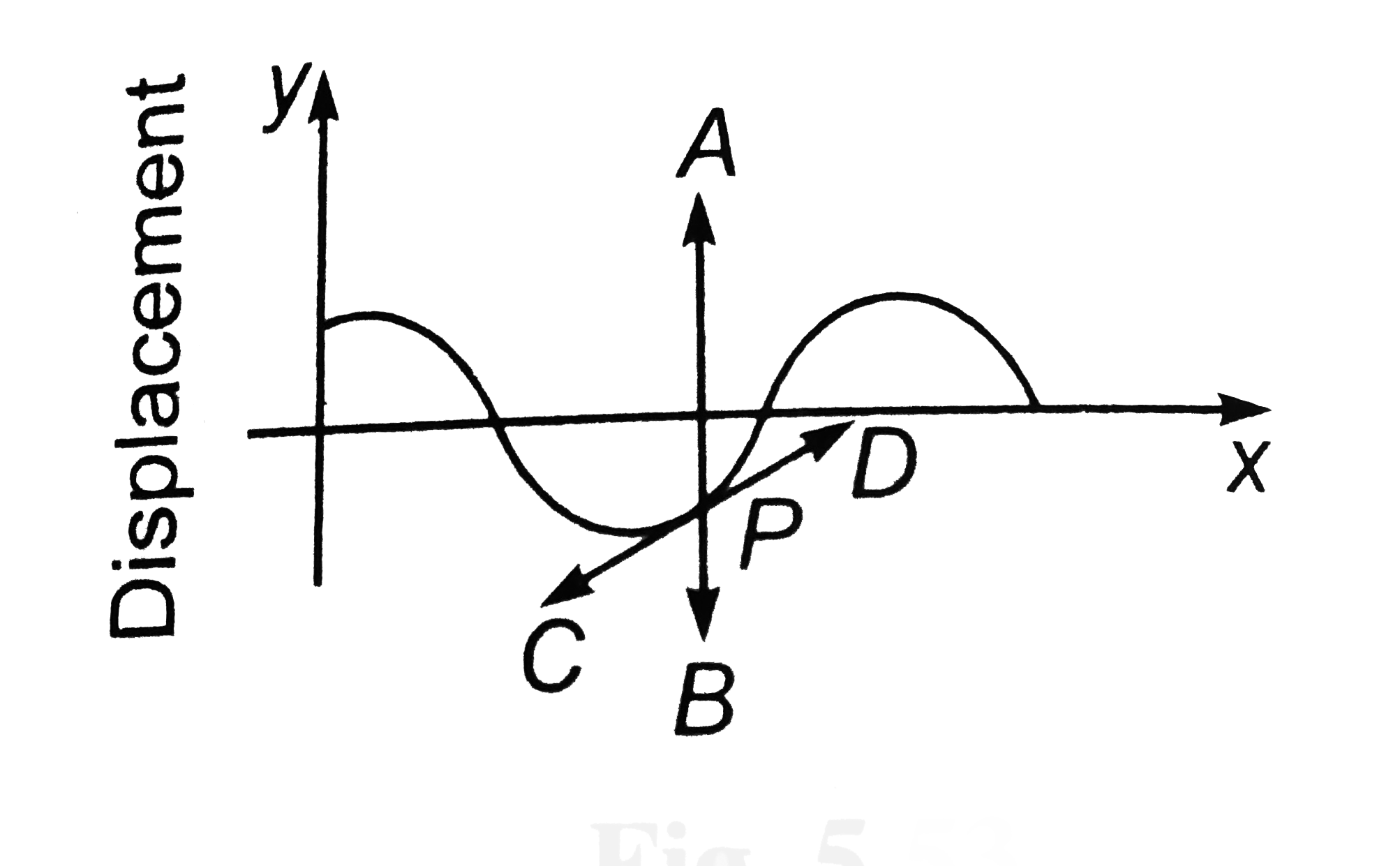
View Solution