The graph Shows the variation in magnetic flux ϕ(t) with time through a coil. Which of the statements given below is not correct
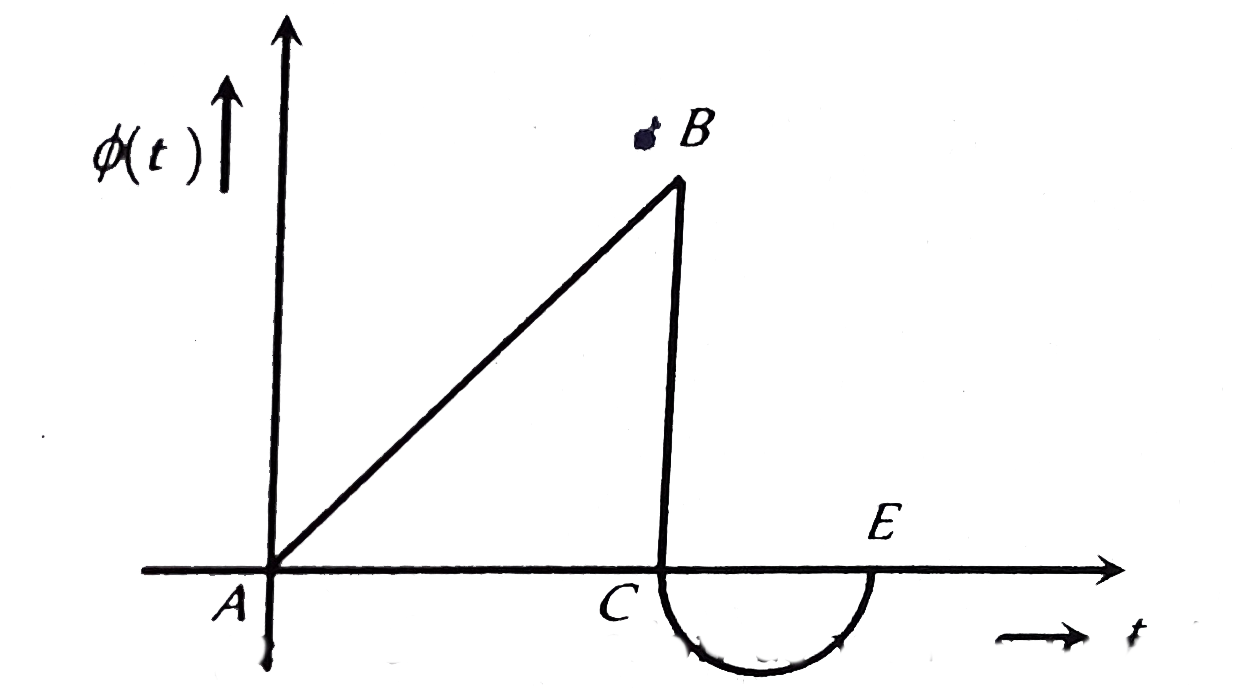
There is a change in the direction as well as magnitude of the induced emf between B and D
The magnitude of the induced emf is maximum between B and C
There is a change in the direction as well as magnitude of induced emf between A and C
The induced emf is zero at B
The correct Answer is:D
Topper's Solved these Questions
- BOOK - ERRORLESS CHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - Assertion & Reason5videos
ELECTRO MAGNETIC INDUCTION
- BOOK - ERRORLESS CHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - SET20videos
ELECTRO MAGNETIC INDUCTION
- BOOK - ERRORLESS CHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - CT3videos
ELECTRO MAGNETIC INDUCTION
- BOOK - ERRORLESS CHAPTER - CURRENT ELECTRICITYEXERCISE - Self Evaluation Test -1923videos
CURRENT ELECTRICITY
- BOOK - ERRORLESS CHAPTER - ELECTRON, PHOTON, PHOTOELECTRIC EFFECT AND X-RAYSEXERCISE - Self Evaluation Test3videos
ELECTRON, PHOTON, PHOTOELECTRIC EFFECT AND X-RAYS
Similar Questions
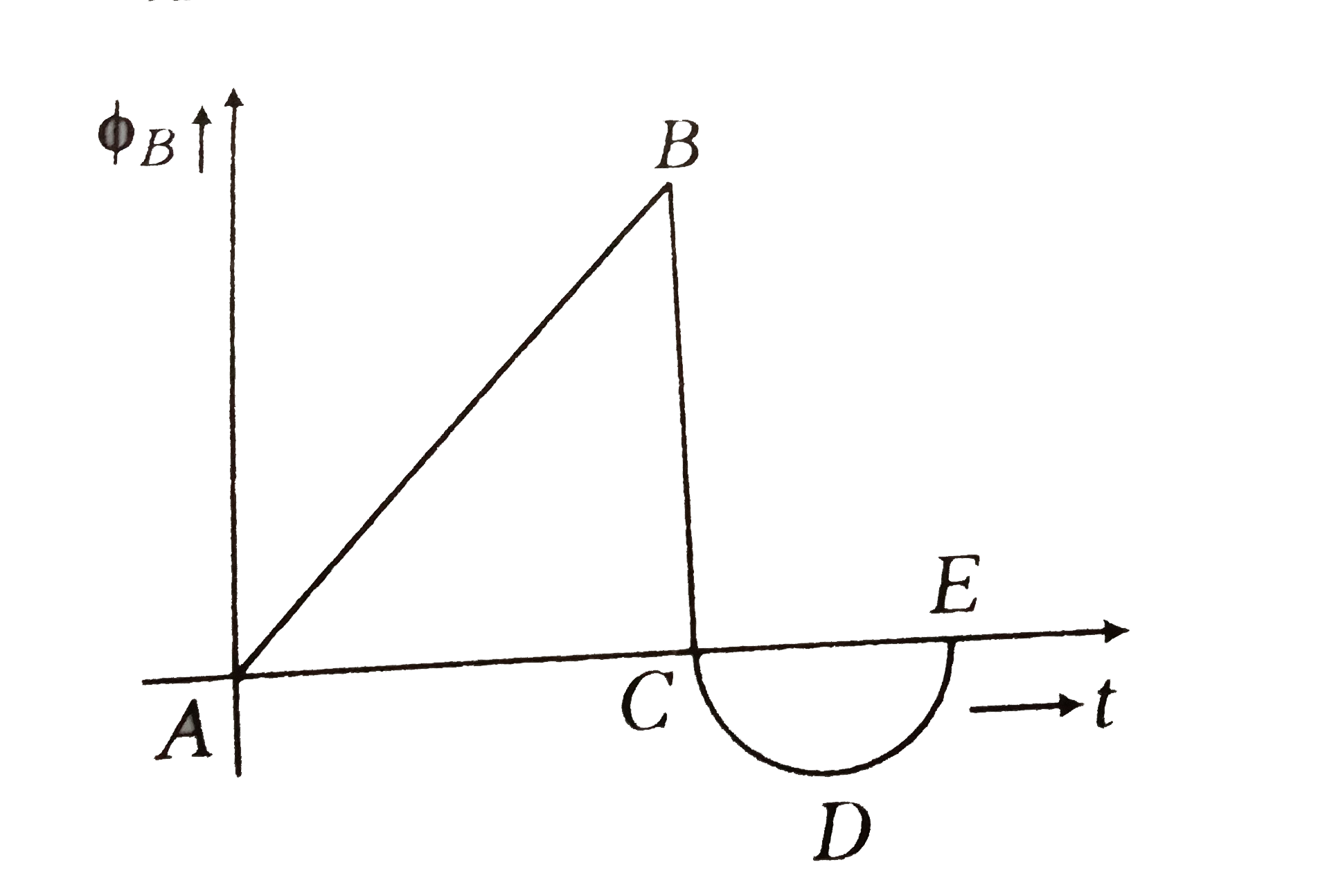
The graph shows the variation in magnetic flux ϕ(t) with time through a coil. Which of the statements given below in not correct?
 View Solution
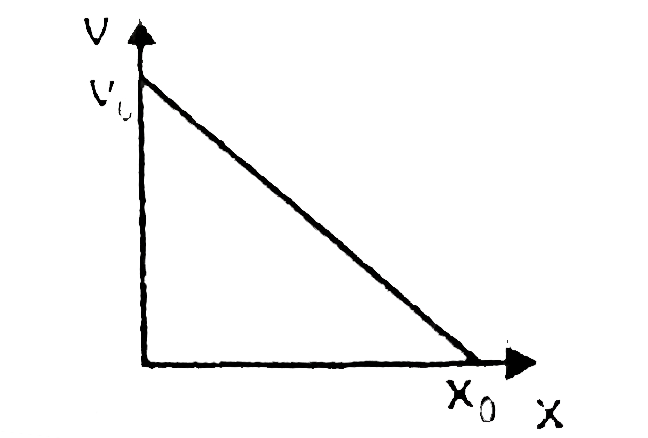
View SolutionThe given graph shows the variation of velocity with displacement. Which one of the graph given below correctly represents the variation of acceleration with displacement :-
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The graph shows the variation in magnetic flux ϕ(t) with time through a coil. Which of the statements given below in not correct?
 AThere is a change in the direction as well as magnitude of the induced emf between B and DBThe magnitude of the induced emf is maximum between B and CCThere is a change in the direction as well as magnitude of induced emf between A and CDThe induced emf is not zero at B
AThere is a change in the direction as well as magnitude of the induced emf between B and DBThe magnitude of the induced emf is maximum between B and CCThere is a change in the direction as well as magnitude of induced emf between A and CDThe induced emf is not zero at B - Question 2 - Select One
The given graph shows the variation of velocity with displacement. Which one of the graphs given below correctly represents the variation of acceleration with displacement ?
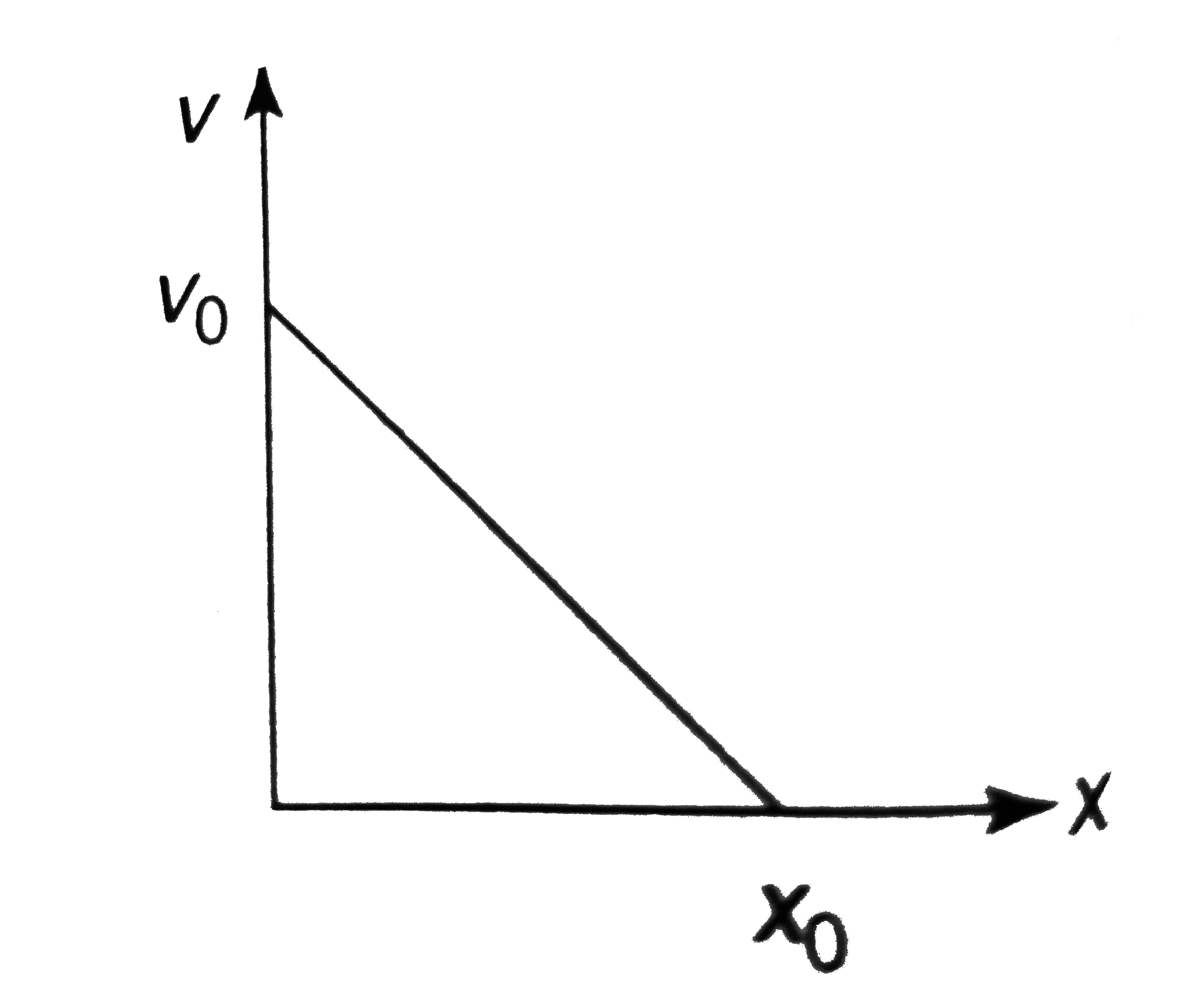 A
A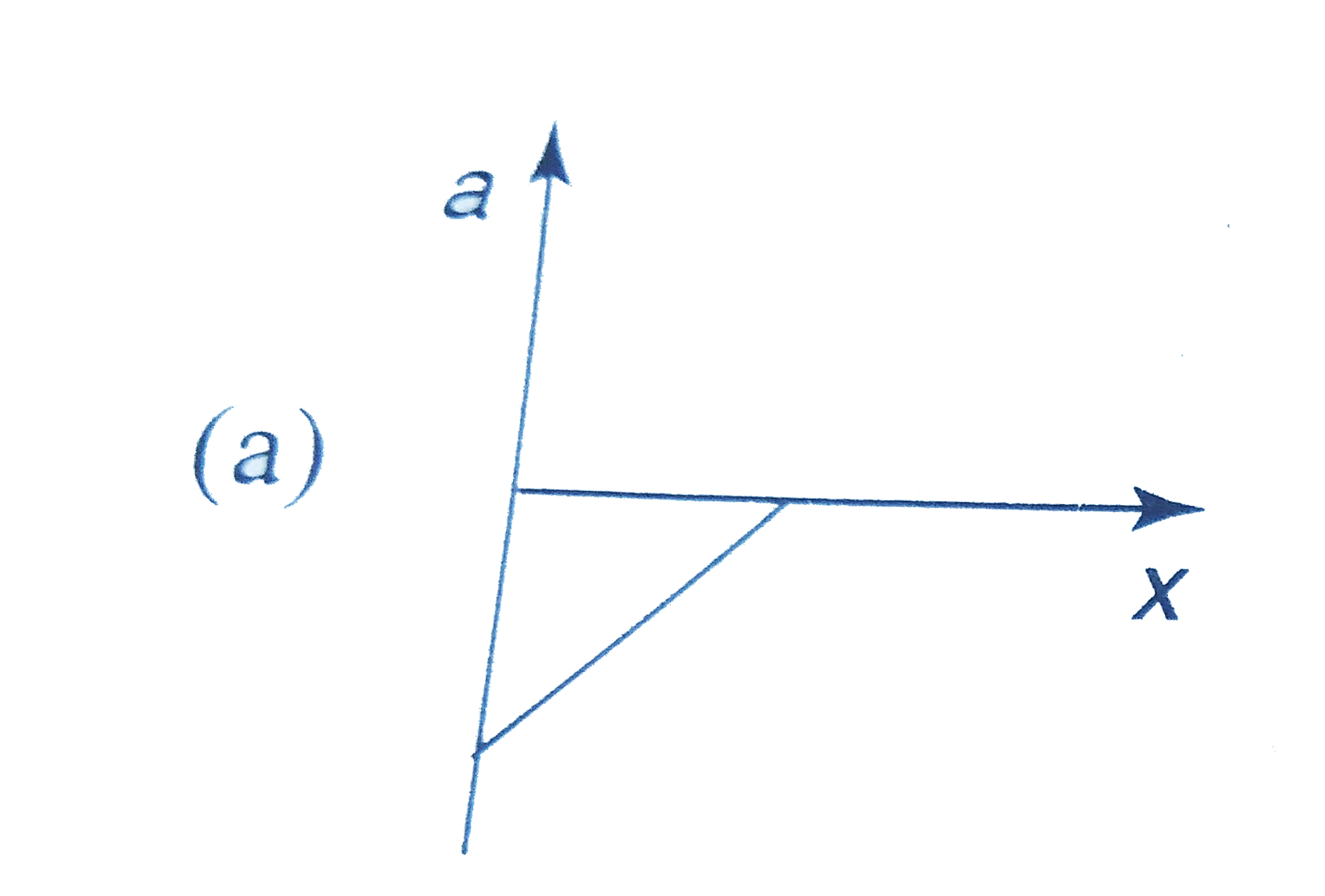 B
B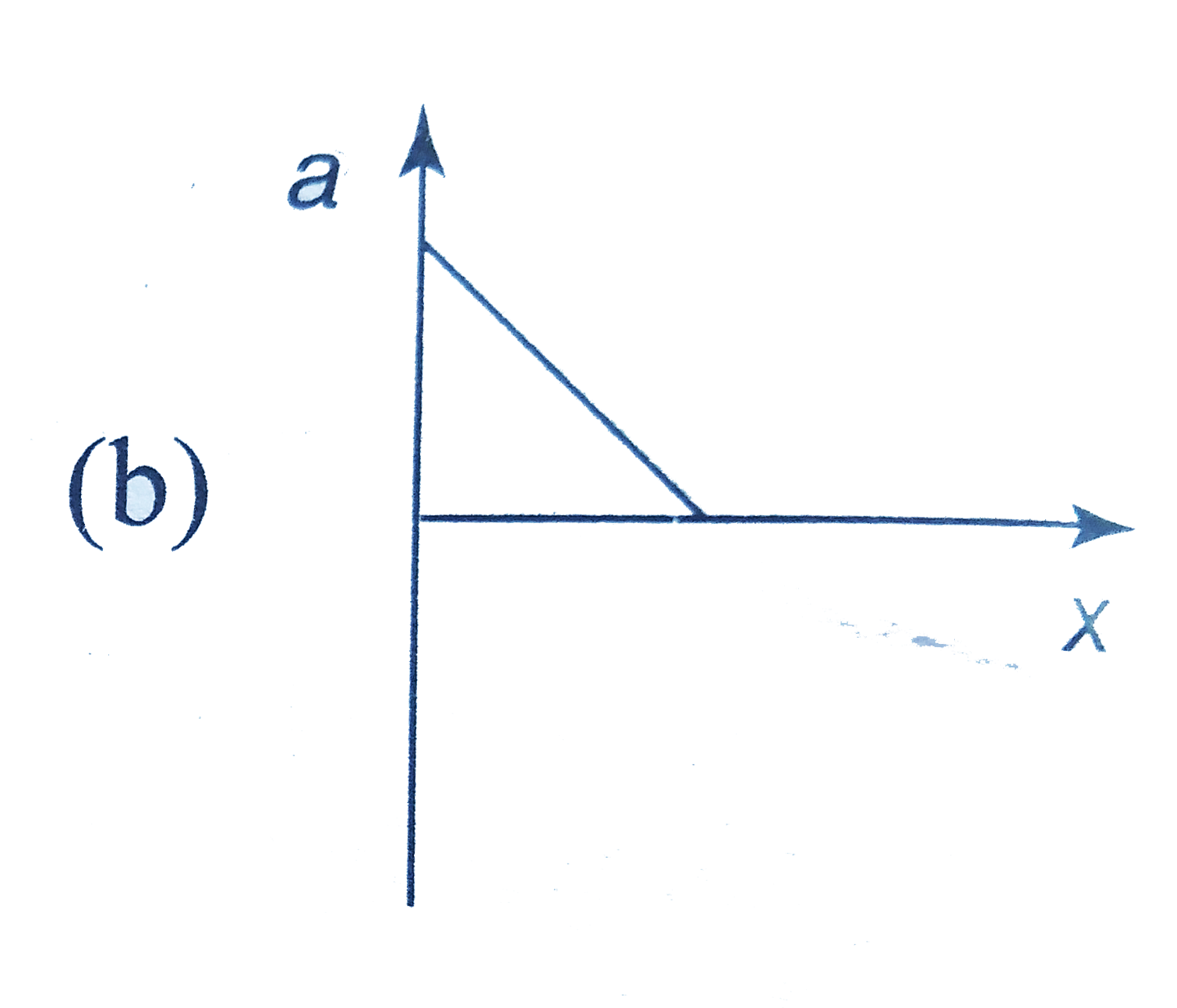 .C
.C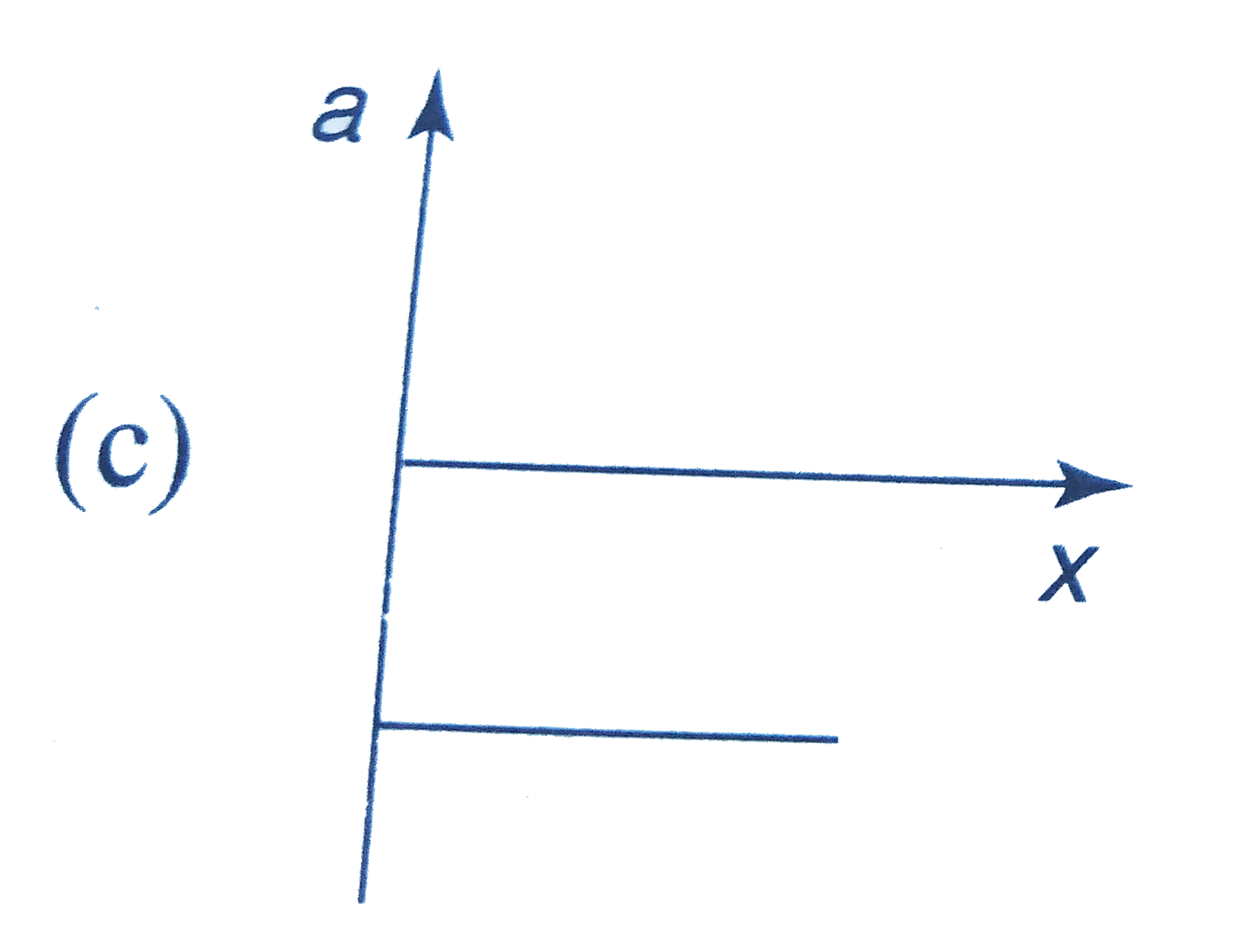 .D
.D .
. - Question 3 - Select One
Let magnetic flux be ϕB , induced emf be ξ and power P. All the three quantities related with each other, that is, if we vary the magnetic flux, induced emf and power change according the variation of magnetic flux. In the given table, Column I shows the variation of magnetic flux, Column II shows the effect on induced emf and Column III shows the effect on power.

What are the conditions for a coil entering the magnetic field?A(I) (iii) (L)B(IV) (iv) (M)C(IV) (iv) (K)D(I) (iii) (J)
The given graph shows the variation of velocity with displacement. Which one of the graphs given below correctly represents the variation of acceleration with displacement ?
 View Solution
View SolutionConsider a rectangular coil of wire carrying constant current I, forming a magnetic dipole. The magnetic flux through an infinite plane that contains the circular coil and excluding the rectangular area is given by ϕi. The magnetic flux through the area of the rectangular area is given by ϕ0. Which of the following option is correct?
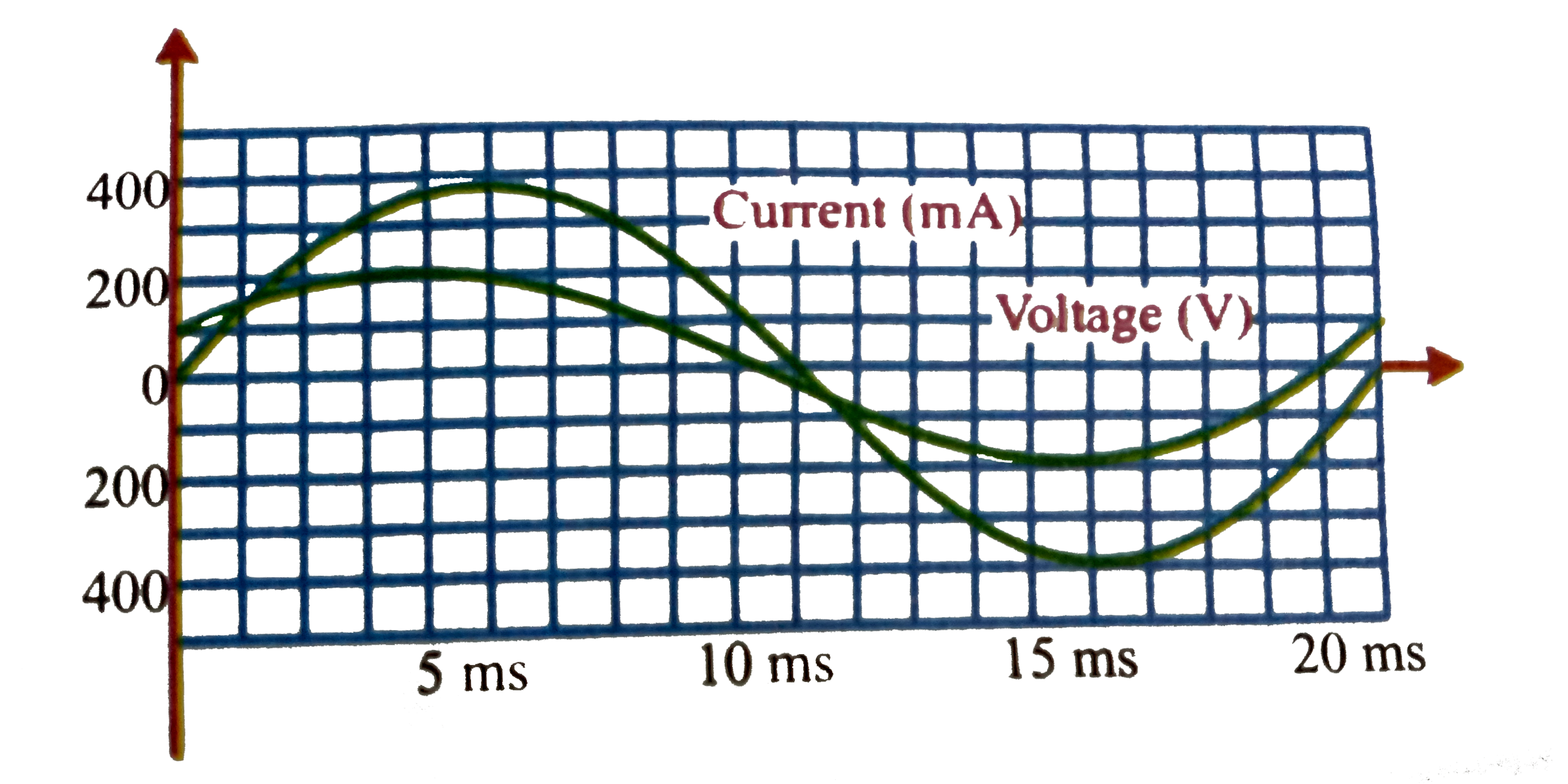
View SolutionThe given graph shows variation with time in the source voltage and steady state current drawn by a series RLC circuit. Given curve through origin is the current variation.
Which of the following statements is/are correct ?
 View Solution
View SolutionA closedc coil with a resitance R is placed in a magnetic field. The flux linked with the coil is ϕ. If the magnetic field is suddenly reversed in direction, the charge that flows through the coil will be
View SolutionLet magnetic flux be ϕB , induced emf be ξ and power P. All the three quantities related with each other, that is, if we vary the magnetic flux, induced emf and power change according the variation of magnetic flux. In the given table, Column I shows the variation of magnetic flux, Column II shows the effect on induced emf and Column III shows the effect on power.

What are the conditions for a coil moving in the magnetic field?View Solution

