The height of mercury which exerts the same pressure as 20 cm of water column, is
1.47 cm
14.8cm
148 cm
None of these
The correct Answer is:A
To find the height of mercury that exerts the same pressure as a 20 cm column of water, we will use the formula for pressure:
P=ρ⋅g⋅h
where:
- P = pressure
- ρ = density of the fluid
- g = acceleration due to gravity
- h = height of the fluid column
Step 1: Calculate the pressure exerted by the water column
Given:
- Height of water column, hw=20cm=0.2m
- Density of water, ρw=1000kg/m3 (or 1g/cm3)
Using the formula for pressure:
Pw=ρw⋅g⋅hw
Pw=1000⋅9.8⋅0.2
Pw=1960Pa
Step 2: Set up the equation for the pressure exerted by the mercury column
Let hm be the height of the mercury column. The density of mercury is:
- ρm=13600kg/m3 (or 13.6g/cm3)
The pressure exerted by the mercury column is:
Pm=ρm⋅g⋅hm
Step 3: Equate the pressures
Since both pressures are equal:
Pw=Pm
1000⋅9.8⋅0.2=13600⋅9.8⋅hm
Step 4: Simplify the equation
We can cancel 9.8 from both sides:
1000⋅0.2=13600⋅hm
200=13600⋅hm
Step 5: Solve for hm
hm=20013600
hm=168
hm≈0.0147m=1.47cm
Final Answer
The height of mercury that exerts the same pressure as a 20 cm water column is approximately 1.47 cm.
---
- P = pressure
- ρ = density of the fluid
- g = acceleration due to gravity
- h = height of the fluid column
Given:
- Height of water column, hw=20cm=0.2m
- Density of water, ρw=1000kg/m3 (or 1g/cm3)
Pw=ρw⋅g⋅hw
Pw=1000⋅9.8⋅0.2
Pw=1960Pa
Let hm be the height of the mercury column. The density of mercury is:
- ρm=13600kg/m3 (or 13.6g/cm3)
Pm=ρm⋅g⋅hm
Since both pressures are equal:
Pw=Pm
1000⋅9.8⋅0.2=13600⋅9.8⋅hm
We can cancel 9.8 from both sides:
1000⋅0.2=13600⋅hm
200=13600⋅hm
hm=20013600
hm=168
hm≈0.0147m=1.47cm
The height of mercury that exerts the same pressure as a 20 cm water column is approximately 1.47 cm.
Topper's Solved these Questions
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - LAWS OF MOTION, FRICTION AND ARCHIMEDES' PRINCIPLEEXERCISE - HIGHER ORDER THINKING QUESTIONS34videos
LAWS OF MOTION, FRICTION AND ARCHIMEDES' PRINCIPLE
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEATING AND MAGNETIC EFFECTS OF CURRENT EXERCISE - HIGHER ORDER THINKING QUESTIONS34videos
HEATING AND MAGNETIC EFFECTS OF CURRENT
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - MEASUREMENTEXERCISE - ELEMENTARY QUESTIONS (HIGHER ORDER THINKING QUESTIONS)13videos
MEASUREMENT
Similar Questions
Knowledge Check
- Question 1 - Select One
The height of mercury column of a barometer at the sea level is
A76 mB76 cmC1 mD0.76 mm - Question 2 - Select One
Which of the following water columns exerts the highest pressure on the bottom of the container?
A10 kmB100 mC1000 mmD5000 m
The height of the mercury column in a barometer at a place is 75 cm. If a liquid of unknown density is used the height of the liquid column is 150 cm, find its density.
View SolutionThe height of the mercury column in a barometer at a place is 75 cm. If a liquid of unknown density is used the height of the liquid column is 150 cm, find its density.
View SolutionA barometer tube is inclined at an angle of 30∘ with horizontal direction. Calculate length of mercury column in tube if atmospheric pressure is 76 cm of mercury.
View SolutionThe length of water column that can exert 1 atm pressure is ________ .
View SolutionSuppose water is used in a barometer instead of mercury. If the barometric pressure is 760mmHg, what is the height of the water column in the barometer at 0∘C. The densities of water and mercury at 0∘C are 0.99987gcm−3 and 13.596gcm−3 , respectively.
Strategy : The prtessure exerted by a column of liquid h whose density is d is hdg. Because the pressure are equal , we can equate the expressions for water (W) and mercury (M):
hWdWg=hMdMg
or hWdW=hMdM
Rearranging gives
hWhM=dMdW
This implies that the height of the liquid column in inversely proportional to its density . Solve the equation to find the height of the water column, hW.View SolutionThe liquids shown in figure in the two arms are mercury (specific gravity =13.6) and water. If the difference of heights of the mercury columns is 2 cm, find the height of the water column.
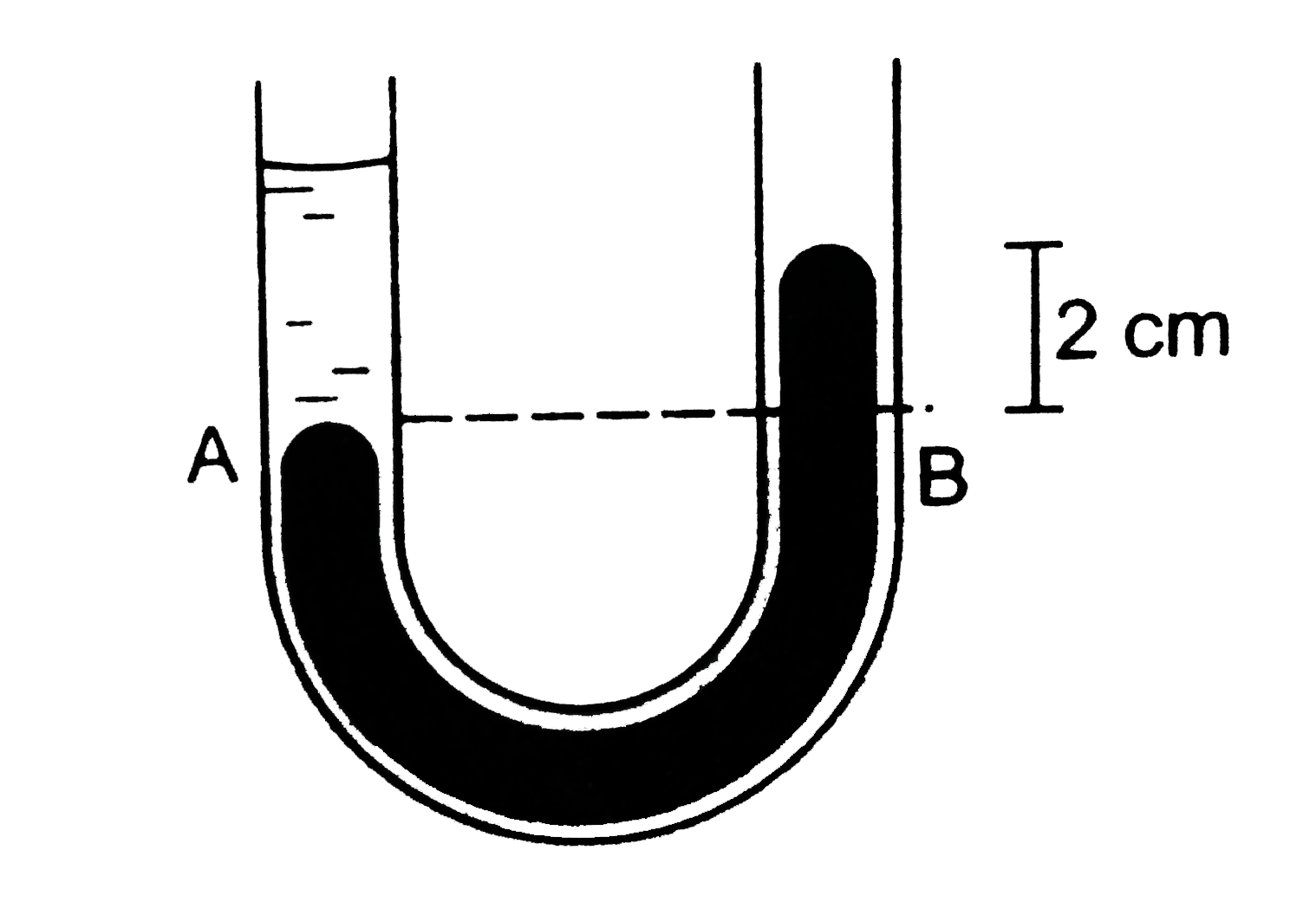 ,View Solution
,View Solution

