The ideal gas equation for an adiabatic process is
=constant
=constant
=constant
T=constant
The correct Answer is:A
To solve the question regarding the ideal gas equation for an adiabatic process, we need to understand the relationship between pressure (P), volume (V), and temperature (T) in such processes.
1. Understanding Adiabatic Processes:
- An adiabatic process is one in which no heat is exchanged with the surroundings. This means that all the work done on or by the system results in a change in internal energy.
2. Ideal Gas Law:
- The ideal gas law is given by the equation PV=nRT, where P is pressure, V is volume, n is the number of moles of gas, R is the universal gas constant, and T is temperature.
3. Adiabatic Condition:
- For an ideal gas undergoing an adiabatic process, the relationship between pressure and volume can be expressed as:
PVγ=constant
- Here, γ (gamma) is the ratio of specific heats, defined as γ=CpCv, where Cp is the specific heat at constant pressure and Cv is the specific heat at constant volume.
4. Conclusion:
- Therefore, the ideal gas equation for an adiabatic process is:
PVγ=constant
Final Answer:
The ideal gas equation for an adiabatic process is PVγ=constant.
---
- An adiabatic process is one in which no heat is exchanged with the surroundings. This means that all the work done on or by the system results in a change in internal energy.
- The ideal gas law is given by the equation PV=nRT, where P is pressure, V is volume, n is the number of moles of gas, R is the universal gas constant, and T is temperature.
- For an ideal gas undergoing an adiabatic process, the relationship between pressure and volume can be expressed as:
PVγ=constant
- Here, γ (gamma) is the ratio of specific heats, defined as γ=CpCv, where Cp is the specific heat at constant pressure and Cv is the specific heat at constant volume.
- Therefore, the ideal gas equation for an adiabatic process is:
PVγ=constant
The ideal gas equation for an adiabatic process is PVγ=constant.
Topper's Solved these Questions
- THERMODYNAMICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - HOTs
8 Videos
- THERMODYNAMICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Assertion & Reason
15 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Assertion And Reason
10 Videos
- UNITS AND MEASUREMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - UNITS AND MEASUREMENTSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
Write ideal gas equation.
View SolutionThe work done by 1 mole of ideal gas during an adiabatic process is (are ) given by :
View Solutionρ-T equation of a gas in adiabatic process is given by
View SolutionThe relation between U, p and V for an ideal gas in an adiabatic process is given by relation U=a+bpV. Find the value of adiabatic exponent (γ) of this gas.
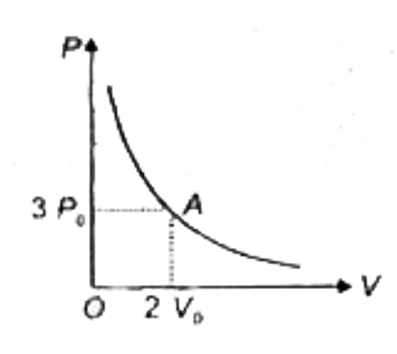
View SolutionThe variation of pressure P with volume V for an ideal monatomic gas during an adiabatic process is shown in figure . At point A the magnitude of rate of change of pressure with volume is
View SolutionA : The specific heat of an ideal gas is zero in an adiabatic process.
R : Specific heat of a gas is process independent.
View SolutionA rigid diatomic ideal gas undergoes an adiabatic process at room temperature .The relation between temperature and volume for this process in TVx= constant ,then x is :
View SolutionA rigid diatomic ideal gas undergoes an adiabatic process at room temperature .The relation between temperature and volume for this process in TVx= constant ,then x is :
View SolutionTwo ideal monoatomic and diatomic gases are mixed with one another to form an ideal gas mixture. The equation of the adiabatic process of the mixture is PVγ= constant, where γ=117 If n1 and n2 are the number of moles of the monoatomic and diatomic gases in the mixture respectively, find the ratio n1n2
View SolutionWhich equation is correct for adiabatic process ?
View Solution
