The length of potentiometer wire is 1m and its resistance is 4Ω. Acurrent of 5mA is flowing in it. An unknown emf is balanced on 40 cm length of this wire. The unknown emf is x mv. What is the value of .x. ?
The correct Answer is:8
To solve the problem step by step, we will follow the outlined approach in the video transcript.
Step 1: Identify the given values
- Length of the potentiometer wire (L) = 1 m
- Resistance of the wire (R) = 4 Ω
- Current flowing through the wire (I) = 5 mA = 0.005 A
- Length at which the unknown EMF is balanced (l1) = 40 cm = 0.4 m
Step 2: Calculate the total potential (voltage) across the potentiometer wire using Ohm's law
Ohm's law states that V=I×R.
V=0.005A×4Ω=0.02V=20mV
Step 3: Calculate the potential gradient (k) of the wire
The potential gradient (k) is defined as the potential per unit length of the wire.
k=VL=20mV1m=20mV/m
Step 4: Calculate the unknown EMF (E) using the potential gradient
The unknown EMF can be calculated using the formula:
E=k×l1
Substituting the values we have:
E=20mV/m×0.4m=8mV
Step 5: Conclusion
The unknown EMF is 8 mV, thus the value of x is:
8
---
- Length of the potentiometer wire (L) = 1 m
- Resistance of the wire (R) = 4 Ω
- Current flowing through the wire (I) = 5 mA = 0.005 A
- Length at which the unknown EMF is balanced (l1) = 40 cm = 0.4 m
Ohm's law states that V=I×R.
The potential gradient (k) is defined as the potential per unit length of the wire.
The unknown EMF can be calculated using the formula:
The unknown EMF is 8 mV, thus the value of x is:
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE SHEET (EXERCISE - VI LEVEL-I (MAIN) (Straight Objective Type Questions))
10 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE SHEET (EXERCISE - VI LEVEL-II (ADVANCED) (Straight Objective Type Questions))
3 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE SHEET (EXERCISE - V LEVEL-II (ADVANCED) (More than One correct answer Type Questions))
3 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PROBLEMS (LEVEL-II)
27 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
Similar Questions
The length of the potentiometer wire is 600 cm and a current of 40 mA is flowing in it. When a cell of emf 2 V and internal resistance 10Ω is balanced on this potentiometer the balance length is found to be 500 cm. The resistance of potentiometer wire will be
View SolutionIn fig. battery E is balanced on 55cm length of potentiometer wire but when a resistance of 10Ω is connected in parallel with the battery then it balances on 50cm length of the potentiometer wire then internal resistance r of the battery is:-
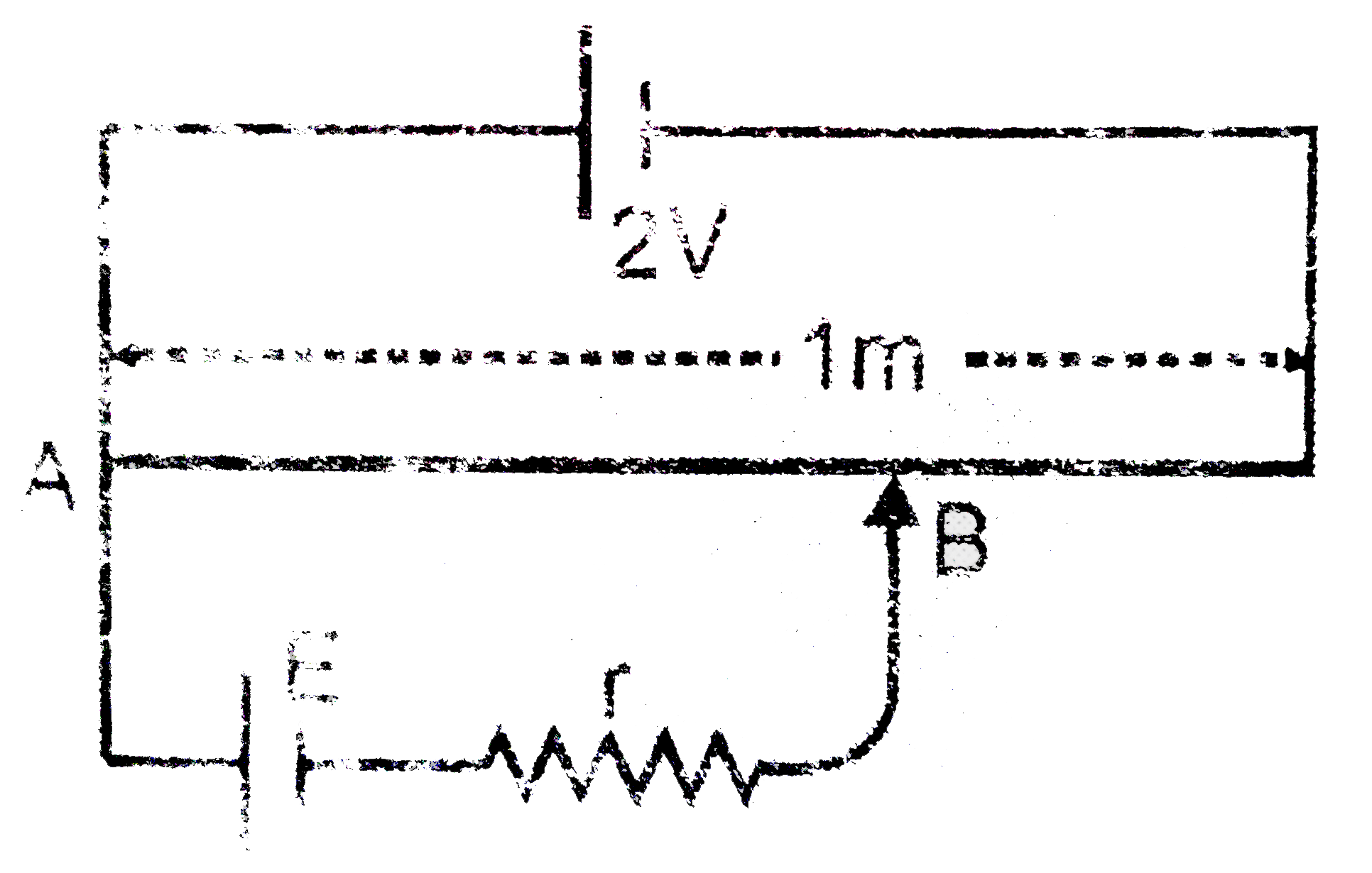
View Solution
Knowledge Check
A potentiometer wire of length 100cm has a resistance of 10Ω. It is connected in series with a resistance and a cell of emf 2V and of negligible interal resistance. A source of emf 10mV is balanced against a length of 40cm of the potentiometer wire. What is the value of external resistance?
A790ΩB890ΩC990ΩD1090Ω
AB is a potentiometer wire of length 100 cm and its resistance is 10Ω. It is connected in series with a resistance R = 40 Ω and a battery of emf 2 V and negligible internal resistance. If a source of unknown emf E is balanced by 40 cm length of the potentiometer wire, the value of E is
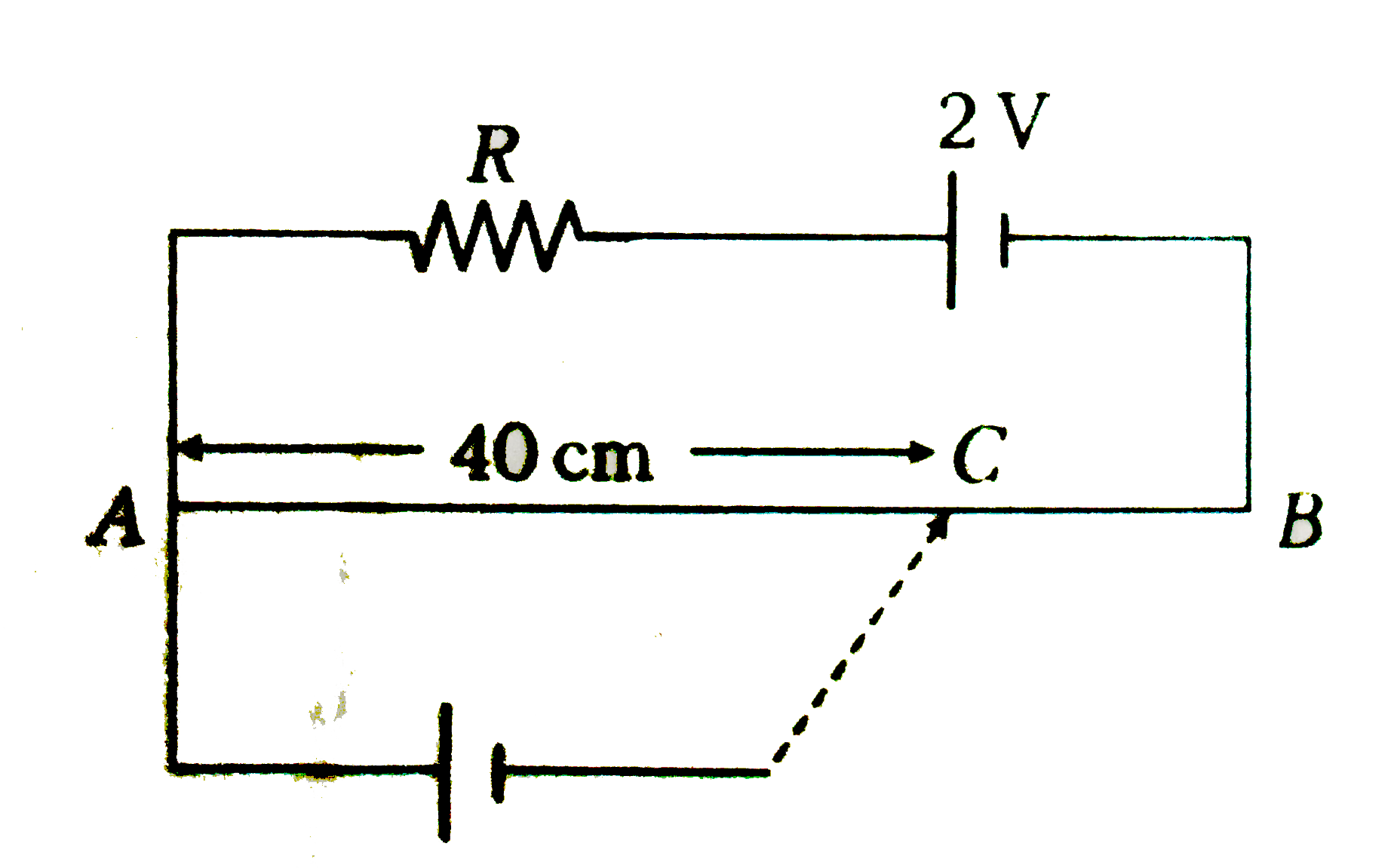
View SolutionA potentiometer wire of length 100 cm has a resistance of 10Ω. It is connected in series with a resistance R and cell of emf 2V and negligible resistance. A source of emf 10 mV is balanced against a length of 40cm of the potentiometer wire. What is the value of R?
View SolutionA potentiometer wire of length 100cm having a resistance of 10Ω is connected in series with a resistance R and a cell of emf 2V of negligible internal resistance. A source of emf

of 10mV is balanced against a length of 40cm of the potentiometer wire. What is the value of resistance R ?
View SolutionA potentiometer wire of length 100cm having a resistance of 10Ω is connected in series with a resistance R and a cell of emf 2V of negligible internal resistance. A source of emf

of 10mV is balanced against a length of 40cm of the potentiometer wire. What is the value of resistance R ?
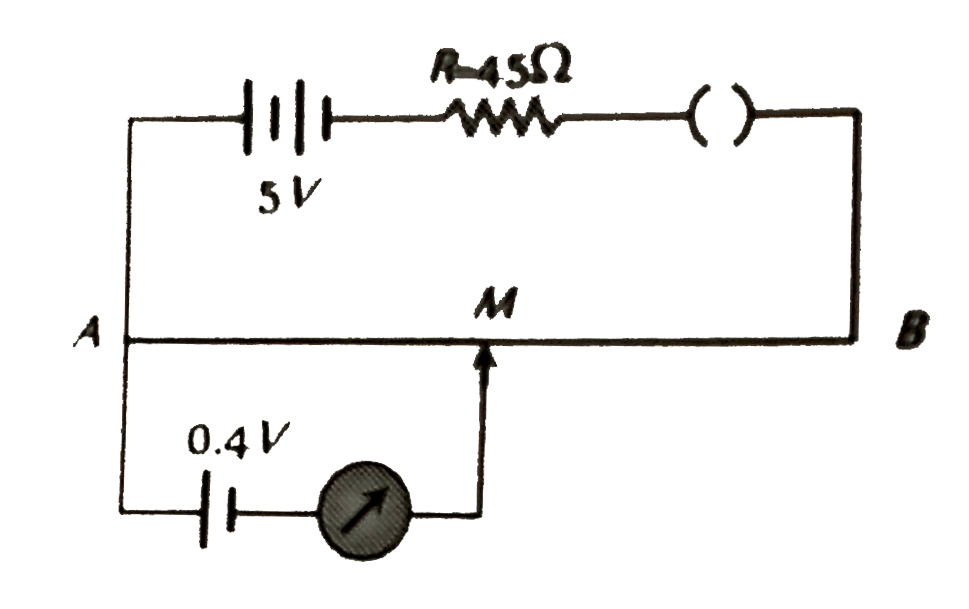
View SolutionIn given figure, the potentiometer wire AB has a resistance of 5Ω and length 10 m . The balancing length AM for the emf of 0.4 V is
View Solutionis made of uniform material and cross-sectional area, and it has uniform resistance per unit length. The potential gradient depends upon the current in the wire.
A potentiometer with a cell of emf 2V and internal resistance 0.4Ω is used across the wire AB. A standard cadmium cell of emf 1.02V gives a balance point at 66cm length of wire. The standard cell is then replaced by a cell of unknows emf e (internal resistance r), and the balance. Point found similarly turns out to be 88cm length of the wire. The length of potentiometer wire AB is 1m.
The reading of the potentiometer, if a 4V battery is used instead of e is
View SolutionThe length of a potentiometer wire is 600 cm and it carries a current of 40mA. For a cell of emf 2V and internal resistance 10Ω, the null point is found to be at 500cm. On connecting a voltmeter acros the cell, the balancing length is decreased by 10cm
The resistance of the voltmeter is
View Solution
