The only force acting on a 2.0−kg body as it moves along the x-axis varies as shown in figure. The velocity of the body at x=0 is 4.0ms−1.
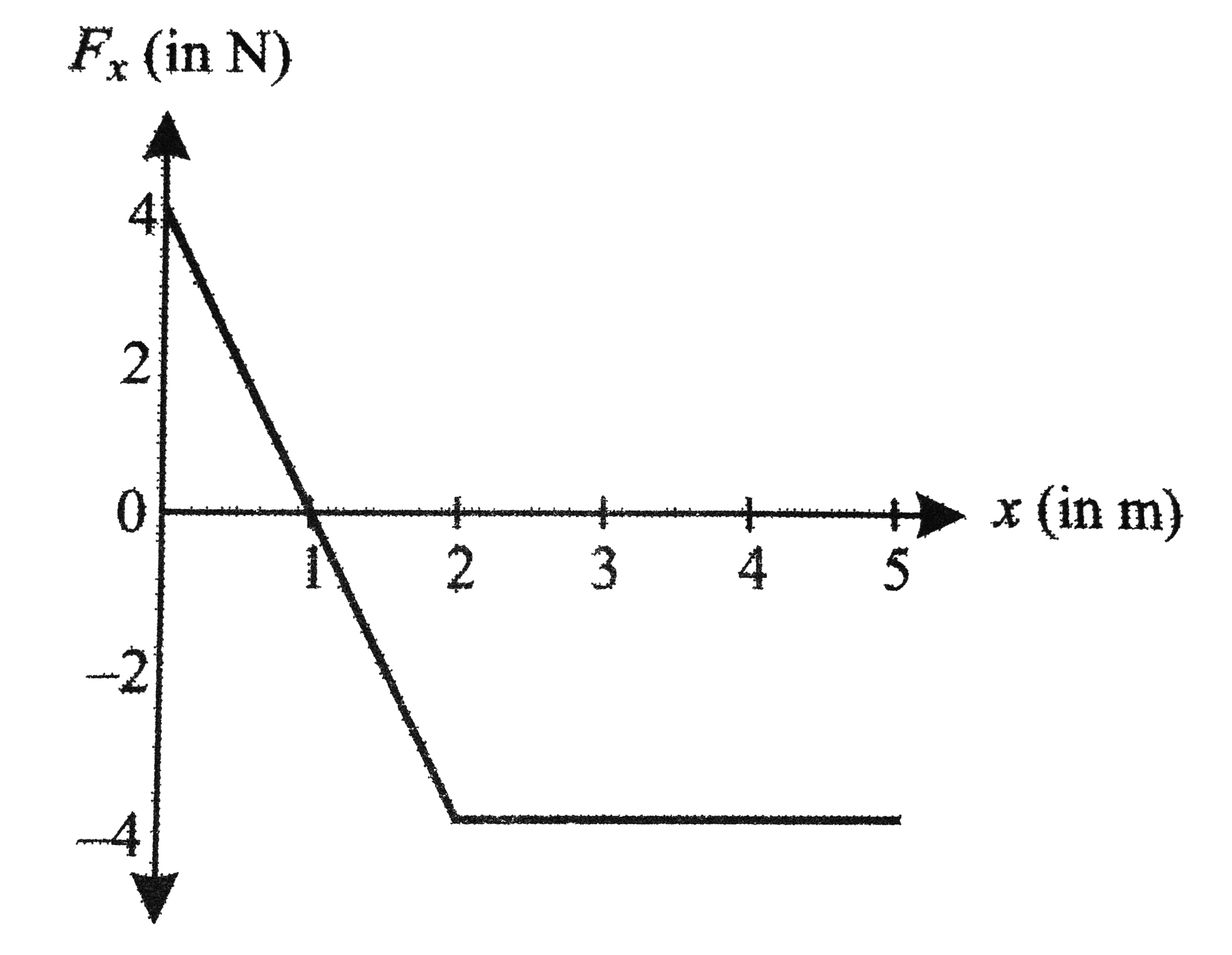
a. What is the kinetic energy of the body at x=3.0m?
b. At what value of x will the body have a kinetic energy of 8.0J?
c. What is the maximum kinetic energy attained by the body between x=0 and x=5.0m?
Video Solution
Text Solution
Kinetic energy of the body at x=0 is
K1=12×2.0×(4.0)2=16.0J
Work done by the force on the body is given by the area bounded by the curve and x-axis in figure. From x=0 to x=1.0m, the force decreases linearly from Fx=4N to 0, but it is directed along positive x-axis. Work done by the force is positive. For 1.0m<x≤5.0m, the force is negative, so it is directed along the negative x-axis for any interval in this region. the work done by the force will be negative.
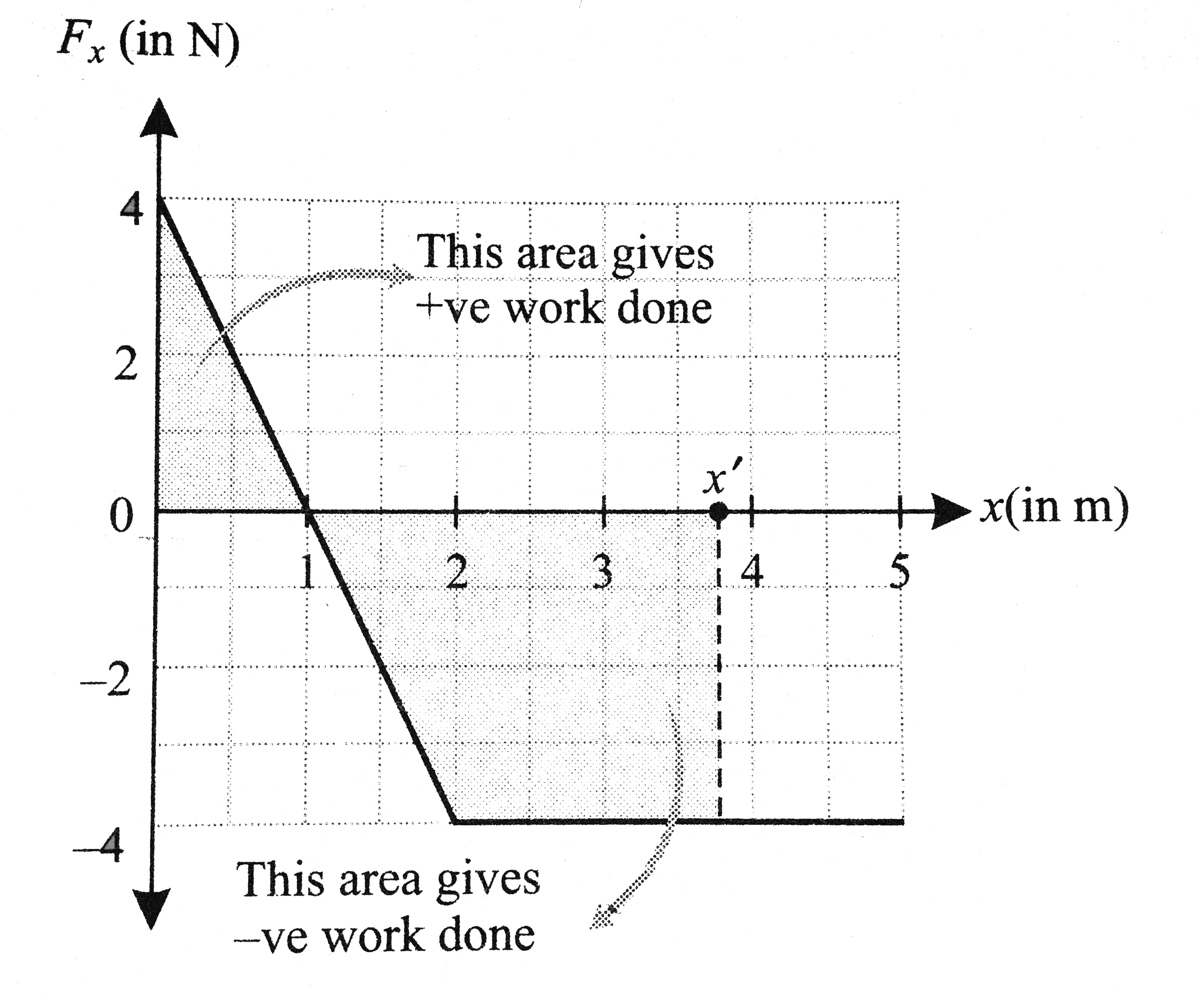
a. From x=0 to 3.0m, work done by the force on the body
=Area of Fx−x diagram between x=0 to x=3.0m region in figure.
12×4×1.0−12×4×1.0−4×1.0=−4.0Nm=−4.0J
Let the kinetic energy of the body at x=3.0m be K3. From the work-energy theorem, W=ΔK.
−4.0=K3−16.0J⇒K3=12.0J
b. Let the kinetic energy of the body be 8.0J at x=x′.
From x=0 to x′, work done by the force=Area of the graph between x=0 to x′ shown in figure, i.e.,
12×4×1.0−12×4×1.0−4×(x′−2.0)Nm=−4×(x′−2.0)J
Using the work-energy theorem, we get
−4×(x′−2.0)=8.0−16.0(∑W=K2−K1)
x′=4.0m
Incidentally, in the diagram, x′ has been drawn between 3.0m and 4.0m. You must not infer from this that x′ lies between 3.0m and 4.0m
c. From x=0 to 5.0m, when the work done by the force is positive, kinetic energy of the block increases. Subsequently, when the work done by the force is negative, kinetic energy of the block decreases. Then, clearly, the kinetic energy of the block is maximum at x=1.0m. Using the work-energy theorem again, we get
12×4×1.0=Kmax−K1
Kmax=K1+2.0=16.0+2.0=18.0J
|
Topper's Solved these Questions
- WORK, POWER & ENERGYBook:CENGAGE PHYSICSChapter:WORK, POWER & ENERGYExercise:Solved Examples
Explore 15 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICSChapter:WORK, POWER & ENERGYExercise:Exercise 8.1
Explore 25 Videos
- VECTORSBook:CENGAGE PHYSICSChapter:VECTORSExercise:Exercise Multiple Correct
Explore 5 Videos
Similar Questions
Knowledge Check
The kinetic energy of a body depends
Aon its mass onlyBon its speed onlyCon its mass as well as on its speedDneither on its mass nor on its speedThe kinetic energy of rolling body is
A1/2Iω2B1/2Iω2+1/2mv2C1/2mv2D1/2mR2The kinetic energy of a body moving along a straight line varies with time as shown in figure. The force acting on the body:
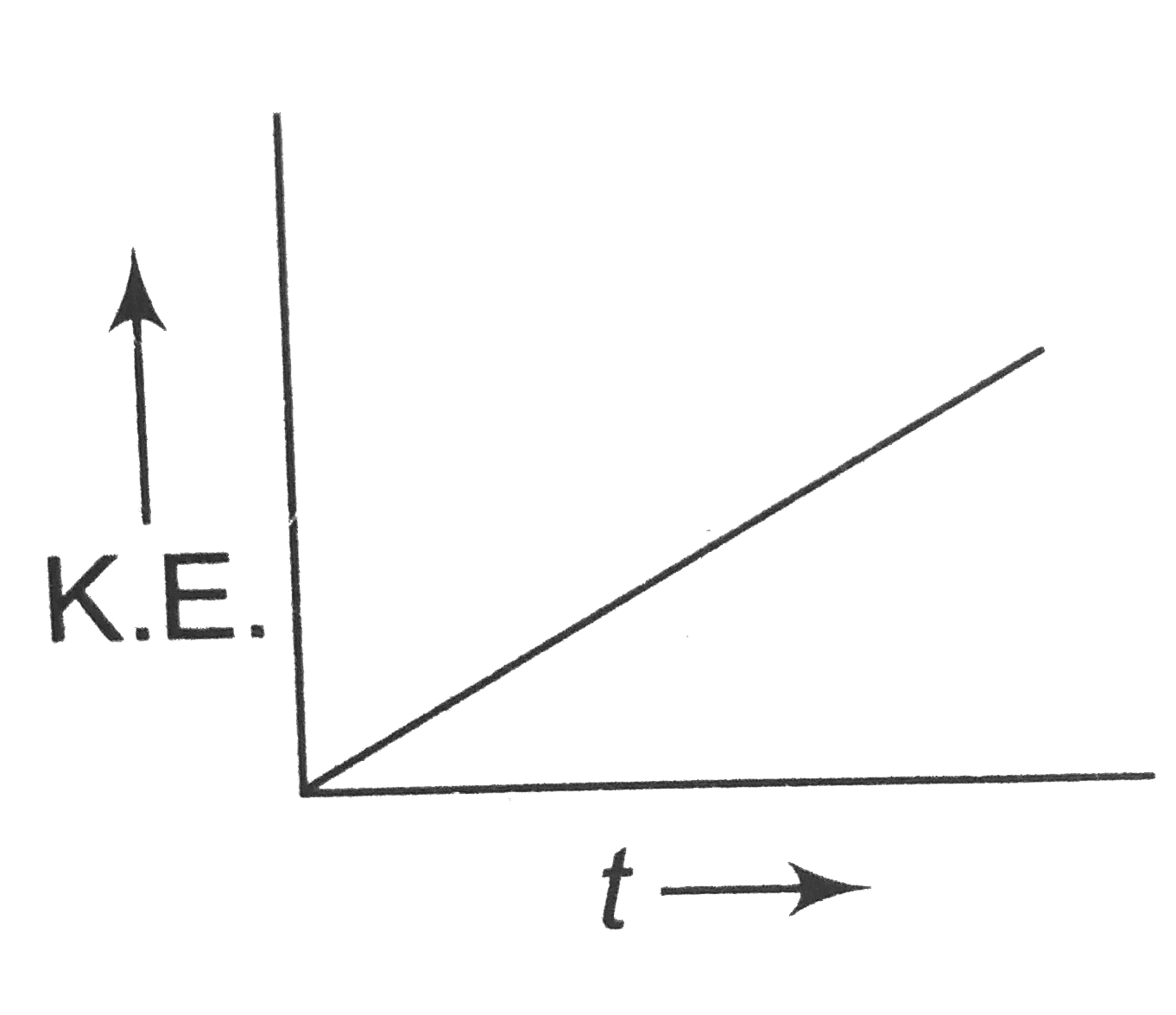 AzeroBconstantCdirectly proportional to velocityDinversely proportional to velocity
AzeroBconstantCdirectly proportional to velocityDinversely proportional to velocity
What will be the velocity of a body of mass 100 g having a kinetic energy of 20 J ?
View SolutionIf kinetic energy of a body is increasing then.
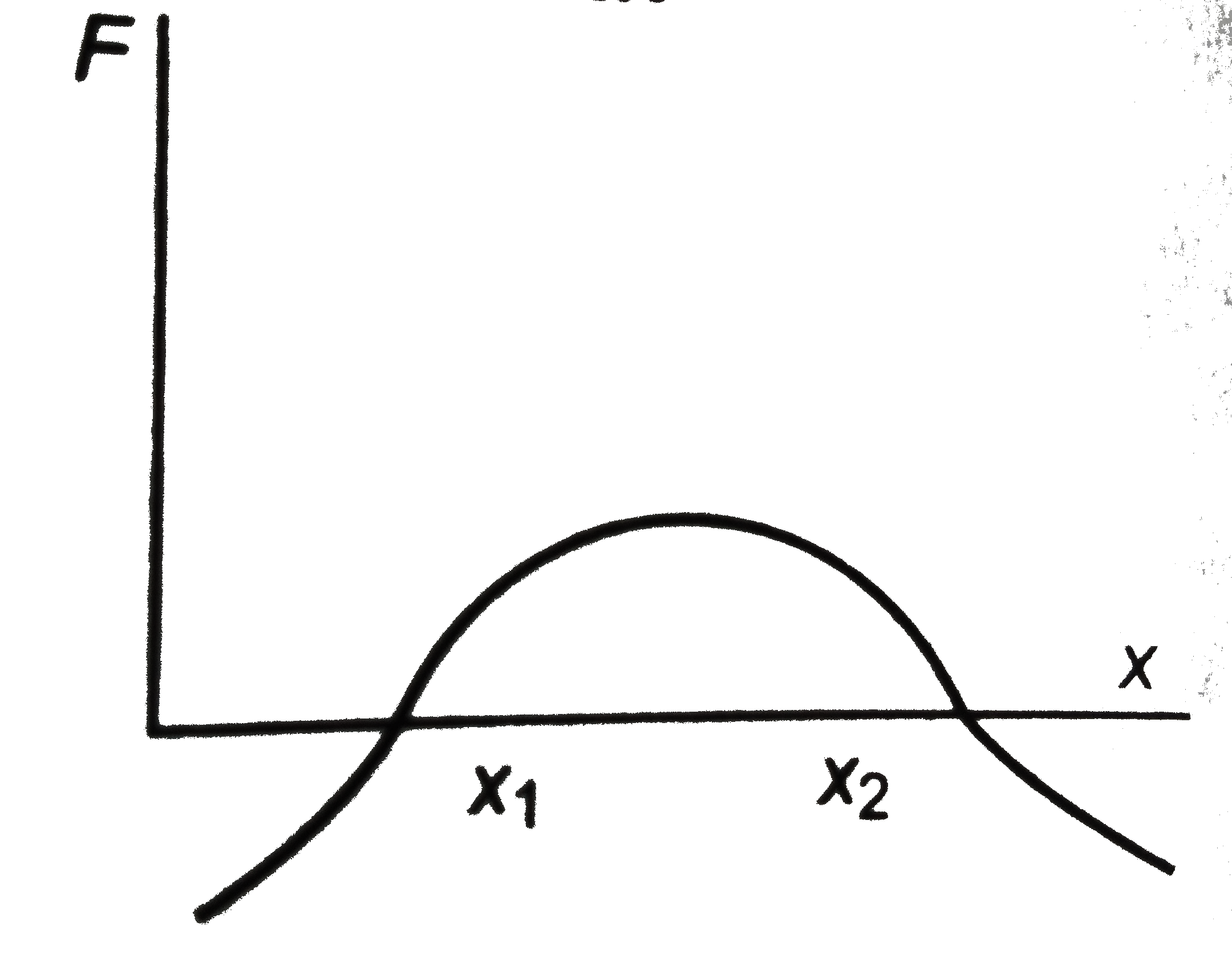
View SolutionThe force acting on a body moving along x-axis varies with the position of the particle shown in the figure. The body is in stable equilibrium at
 .
.
View SolutionThe variation of force F acting on a body moving along x - axis varies with its position (x) as shown in figure
The body is in stable equilibrium stable at
View SolutionWhat will be the kinetic energy of a body when its velocity is halved ?
View Solution
