The potential energy of a particle having mass m is 12mω2x2 where ω is constant. Prove that its motion is SHM.Solution in Bengali
Step by step video & image solution for The potential energy of a particle having mass m is 1/2 momega^2x^2 where omega is constant. Prove that its motion is SHM. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
What does speedometer record?
View SolutionThe dimensional formula for the modulus of elasticity is same as that for
View SolutionA small particle of mass m moves in such a way that the potential energy U=12mω2r2, where ω is a constant and r is the distance of the particle from the origin. Assuming Bohr's model of quantisation of angular momentum and circular orbits. Find the radius of the nth orbit.
View Solution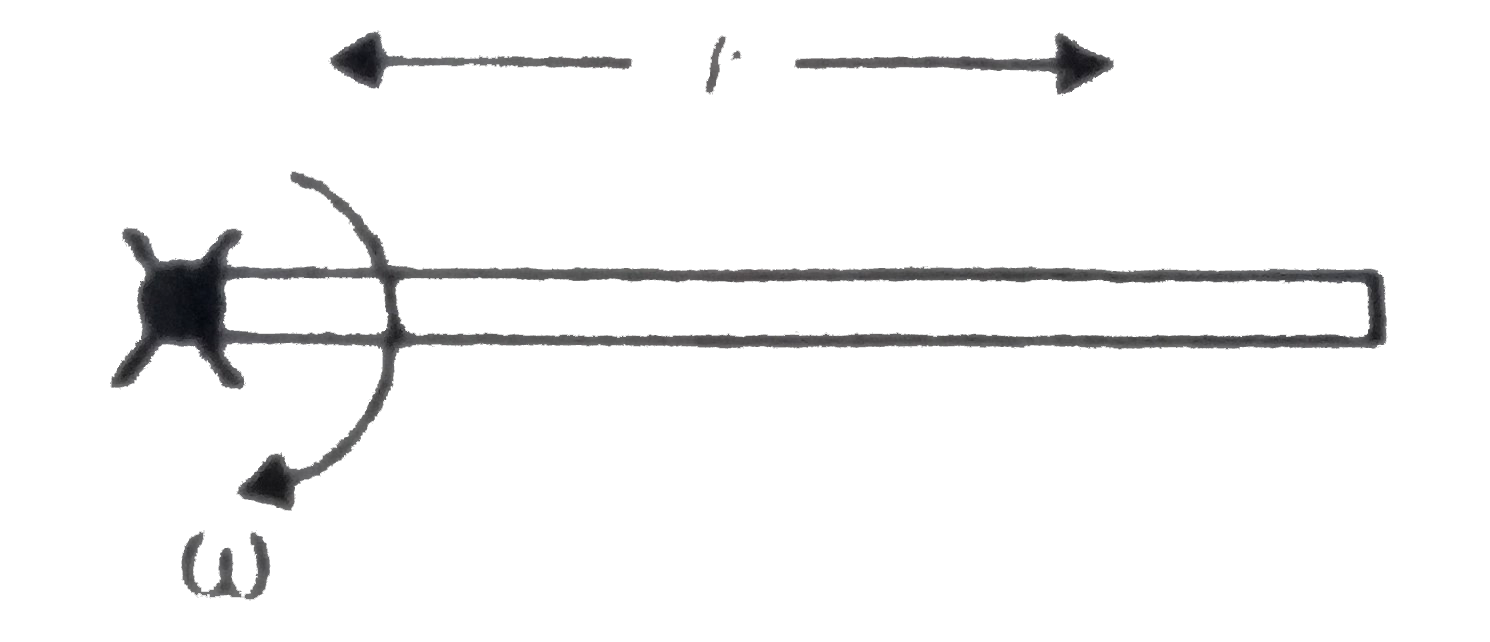
The kinetic energy of a uniform rod of mass m rotating with constant angular velocit ω about one of its end which is fixed as shown in figure is mω2l2N find N
View SolutionTwo simple harmonic motions y_(1) = Asinomegat and y_(2) = Acos omega t are superimposed on a particle of mass m. The total mechanical energy of the particle is
View SolutionThe total mechanical energy of a particle of mass m executing SHM with the help of a spring is E=(1/2)mω2A2. If the particle is replaced by another particle of mass m/2 while the amplitude A remains same. New mechanical energy will be :
View SolutionA particle of mass m in a unidirectional potential field have potential energy U(x)=alpha+2betax^(2) , where alpha and beta are positive constants. Find its time period of oscillations.
View SolutionA particle of mass m executing SHM with amplitude A and angular frequency ω. The average value of the kinetic energy and potential energy over a period is
View SolutionA particle is executing SHM. At a displacement y1 its potential energy is U1 and at a displacement y2 its potential energy is U2. The potential energy of the particle at displacement (y1+y2) is
View SolutionThe potential energy of particle of mass 1kg moving along the x-axis is given by U(x)=16(x2−2x) J, where x is in meter. Its speed at x=1 m is2m/s. Then,
View SolutionThe kinetic energy of a particle is 1/2momega^(2)(A^(2)-x^(2)) , where m, omega and A are constants. Prove that the motion of the particle is simple harmonic.
View SolutionIf the mass of a particle executing SHM is m and its angular frequency is omega , then the force constant of that SHM will be
View SolutionPotential energy of a particle is 12mω2x2, where m and ω are constants. Prove that the motion of the particle is simple harmonic.
View SolutionA particle of mass m is executing SHM about the origin on X-axis with frequency sqrt((ka)/(p m)) ,where k is a constant and a is the amplitude. Find its potential energy, if x is the displacement at time t
View SolutionA particle of mass m executing SHM with amplitude A and angular frequency ω. The average value of the kinetic energy and potential energy over a period is
View SolutionTwo simple harmonic motions y_(1) = Asinomegat and y_(2) = Acos omega t are superimposed on a particle of mass m. The total mechanical energy of the particle is
View Solution
