The power in dioptre of an equi-convex lens with radii of curvature of 10cm and refractive index 1.6 is
+12
+18
+1.2
+1.8
The correct Answer is:A
To find the power of an equi-convex lens with given radii of curvature and refractive index, we can follow these steps:
1. Identify the Given Values:
- Radius of curvature (R) = 10 cm
- Refractive index (μ) = 1.6
2. Understand the Lens Formula:
The formula to find the focal length (F) of a lens is given by:
1F=(μ−1)(1R1−1R2)
For an equi-convex lens, R1=R (positive) and R2=−R (negative). Thus:
R1=10cmandR2=−10cm
3. Substitute the Values into the Lens Formula:
1F=(1.6−1)(110−1−10)
This simplifies to:
1F=0.6(110+110)
1F=0.6(210)=0.6×0.2=0.12
4. Calculate the Focal Length (F):
F=10.12m
Converting this to centimeters:
F=10012cm≈8.33cm
5. Convert Focal Length to Meters:
F=10012×1100m=112m
6. Calculate the Power (P):
The power of the lens in diopters (D) is given by:
P=1F(in meters)
P=1112=12D
Final Answer:
The power of the equi-convex lens is 12 diopters.
---
- Radius of curvature (R) = 10 cm
- Refractive index (μ) = 1.6
The formula to find the focal length (F) of a lens is given by:
1F=(μ−1)(1R1−1R2)
For an equi-convex lens, R1=R (positive) and R2=−R (negative). Thus:
R1=10cmandR2=−10cm
1F=(1.6−1)(110−1−10)
This simplifies to:
1F=0.6(110+110)
1F=0.6(210)=0.6×0.2=0.12
F=10.12m
Converting this to centimeters:
F=10012cm≈8.33cm
F=10012×1100m=112m
The power of the lens in diopters (D) is given by:
P=1F(in meters)
P=1112=12D
The power of the equi-convex lens is 12 diopters.
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Objective Questions
1 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 1 Subjective
29 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 1 Assertion And Reason
13 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
If the plane surface of a plano-convex lens of radius of curvature R and refractive index μ is silvered, then its focal length would be
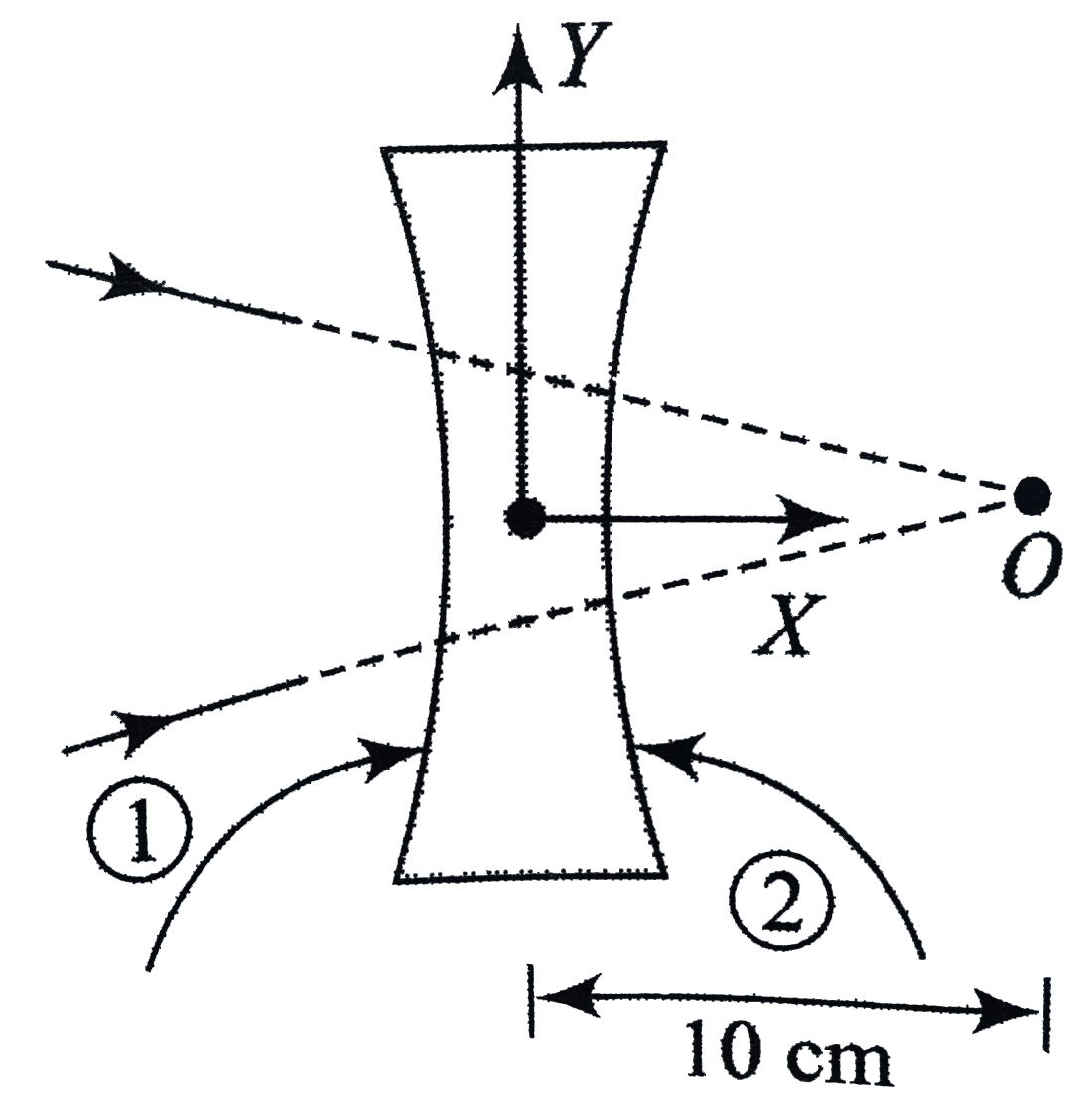
View SolutionA converging bundle of rays is intercepted by a biconcave lens. The radii of curvature of both surfaces are 20cm and the refractive index of the material of the lens is 1.5. If the rays originially converged to a point 10cm in front of the lens, where will they now converge after passing through the lens?
View Solution
Knowledge Check
The power of a biconvex lens is 10 dioptre and the radius of curvature of each surface is 10 cm. Then the refractive index of the material of the lens is
A32B43C98D53What is the refractive index of material of a plano-convex lens , if the radius of curvature of the convex surface is 10 cm and focal length of the lens is 30 cm ?
A65B74C23D43
The focal length of an equi-concave lens is 34 times of radius of curvature of its surfaces. Find the refractive index of the material of lens. Under what condition will this lens behave as a converging lens?
View SolutionCalculate the focal length of a convex lens whose radii of curvature of two surfaces is 10cm and 15cm respectively and its refractive index is 1.5.
View SolutionA plano convex lens of radius of curvature 30 cm and refractive index 1.5 is kept in air. Find its focal length (in cm).
View SolutionA diverging meniscus lens of radii of curvatures 25 cm and 50 cm has a refractive index 1.5. Its focal length is (in cm)
View SolutionImage distance |v| vs object distance |u| curve for two biconvex lenses with same radii of curvatures is shown in the figure. If refractive index of lens 1 is 52, then refractive index of lens 2 is.

View SolutionFind the refractive index of the material of a plano-convex lens, if the radius of curvature of the convex surface is 20 cm and focal length of the lens is 60 cm?
View Solution
