The representation of Highway Underpass whose one zero is 6 and sum of the zeroes is 0, is
(i) x2-6x+2
(ii)x2-36
(iii)x2−6
(iv) x2−3
To solve the problem, we need to find the quadratic equation based on the given information about its roots.
1. Identify the given information:
- One root (α) is given as 6.
- The sum of the roots (α + β) is given as 0.
2. Find the other root (β):
- Since the sum of the roots is 0, we can write the equation:
α+β=0
- Substituting the value of α:
6+β=0
- Solving for β:
β=−6
3. Calculate the product of the roots (α * β):
- Now we can find the product of the roots:
α⋅β=6⋅(−6)=−36
4. Form the quadratic equation:
- The standard form of a quadratic equation based on its roots is:
x2−(sum of roots)x+(product of roots)=0
- Substituting the values we have:
- Sum of roots = 0
- Product of roots = -36
- The equation becomes:
x2−0⋅x−36=0
- Simplifying this gives:
x2−36=0
5. Identify the correct option:
- From the options provided, we can see that the equation x2−36=0 matches option (ii).
Final Answer:
The representation of the highway underpass is given by the equation:
x2−36=0
Thus, the correct option is (ii) x2−36.
- One root (α) is given as 6.
- The sum of the roots (α + β) is given as 0.
- Since the sum of the roots is 0, we can write the equation:
α+β=0
- Substituting the value of α:
6+β=0
- Solving for β:
β=−6
- Now we can find the product of the roots:
α⋅β=6⋅(−6)=−36
- The standard form of a quadratic equation based on its roots is:
x2−(sum of roots)x+(product of roots)=0
- Substituting the values we have:
- Sum of roots = 0
- Product of roots = -36
- The equation becomes:
x2−0⋅x−36=0
- Simplifying this gives:
x2−36=0
- From the options provided, we can see that the equation x2−36=0 matches option (ii).
The representation of the highway underpass is given by the equation:
x2−36=0
Thus, the correct option is (ii) x2−36.
Topper's Solved these Questions
- SAMPLE PAPER (MATHEMATICS STANDARD)BOOK - CBSE MODEL PAPERCHAPTER - SAMPLE PAPER (MATHEMATICS STANDARD)EXERCISE - PART - B
16 Videos
- QUESTION BANK 2021BOOK - CBSE MODEL PAPERCHAPTER - QUESTION BANK 2021EXERCISE - QUESTIONS
60 Videos
- SAMPLE PAPER 2022BOOK - CBSE MODEL PAPERCHAPTER - SAMPLE PAPER 2022EXERCISE - ALTERNATIVE QUESTIONS(SECTION C)
4 Videos
Similar Questions
Knowledge Check
The sum of the zeroes of the polynomial 2x2−8x+6 is
A−3B3C−4D4
Factorise
(i) x2+9x+18 (ii) 6x2+7x−3
(iii) 2x2−7x−15 (iv)84−2r−2r2
View SolutionFactorise:(i) 12x2−7x+1 (ii) 2x2+7x+3 (iii) 6x2+5x−6 (iv) 3x2−x−4
View SolutionThe quadratic polynomial, the sum of whose zeroes is -5 and their product is 6,is
(a) x2+5x+6
(b) x2−5x+6
(c) x2−5x−6
(d) −x2+5x+6
View SolutionCase Study Based- 3
Applications of Parabolas-Highway Overpasses/Underpasses A highway underpass is parabolic in shape.
Parabola
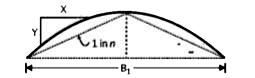
A parabola is the graph that results from p(x)=ax2+bx+c
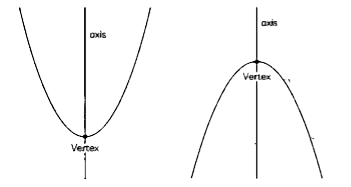
Parabolas are symmetric about a vertical line known as the Axis of Symmetry. The Axis of Symmetry runs through the maximum or minimum point of the parabola which is called the Vertex.
The representation of Highway Underpass whose one zero is 6 and sum of the zeroes is 0, is
View SolutionThe sum of the zeroes of the polynomial 2x2−8x+6 is :
View SolutionFind the zeroes of the polynomial 6x2−3.
View SolutionFind the zeroes of the polynomial 6x2−3
View Solution
