The speed of the bob of a simple pendulum is maximum at its ______.
The correct Answer is:mean position
To solve the question, "The speed of the bob of a simple pendulum is maximum at its ______," we can follow these steps:
1. Understanding the Pendulum Motion: A simple pendulum consists of a mass (the bob) attached to a string that swings back and forth under the influence of gravity. The motion can be analyzed in terms of potential energy and kinetic energy.
2. Identifying Positions: The pendulum has two extreme positions (maximum displacement from the mean position) and one mean position (the lowest point in the swing).
- Extreme positions are where the pendulum is at its highest points on either side.
- The mean position is the lowest point of the swing.
3. Energy Considerations:
- At the extreme positions, the pendulum has maximum potential energy and minimum kinetic energy. This is because the bob is momentarily at rest at these points.
- At the mean position, the pendulum has minimum potential energy and maximum kinetic energy. This is because the bob is moving the fastest at this point.
4. Kinetic Energy and Speed: Kinetic energy (KE) is given by the formula:
KE=12mv2
where m is the mass of the bob and v is its speed. Since kinetic energy is maximum at the mean position, it implies that the speed of the bob is also maximum at this position.
5. Conclusion: Therefore, the speed of the bob of a simple pendulum is maximum at its mean position.
Final Answer:
The speed of the bob of a simple pendulum is maximum at its mean position.
---
- Extreme positions are where the pendulum is at its highest points on either side.
- The mean position is the lowest point of the swing.
- At the extreme positions, the pendulum has maximum potential energy and minimum kinetic energy. This is because the bob is momentarily at rest at these points.
- At the mean position, the pendulum has minimum potential energy and maximum kinetic energy. This is because the bob is moving the fastest at this point.
KE=12mv2
where m is the mass of the bob and v is its speed. Since kinetic energy is maximum at the mean position, it implies that the speed of the bob is also maximum at this position.
The speed of the bob of a simple pendulum is maximum at its mean position.
Topper's Solved these Questions
- KINEMATICSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - KINEMATICSEXERCISE - CONCEPT APPLICATION (LEVEL 1 (SELECT THE CORRECT ALTERNATIVE))
19 Videos
- KINEMATICSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - KINEMATICSEXERCISE - CONCEPT APPLICATION (LEVEL 1 (MATCH THE COLUMN))
3 Videos
- KINEMATICSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - KINEMATICSEXERCISE - CONCEPT APPLICATION (LEVEL 1 (TRUE OR FALSE))
8 Videos
- HEAT BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - HEAT EXERCISE - Test 2
14 Videos
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - ASSESSMENT TEST (TEST 2 (SELECT THE CORRECT ALTERNATIVE))
15 Videos
Similar Questions
Knowledge Check
Kinetic energy of the bob of a simple pendulum is maximum
Aat the mean positionBat the extreme left positionCat the extreme right positionDnone of theseThe velocity of simple pendulum is maximum at
AExtremesBHalf displacementCMean positionDEvery whereIf the speed (v) of the bob in a simple pendulum is plotted against the tangential acceleration (a), the correct graph will be represented by
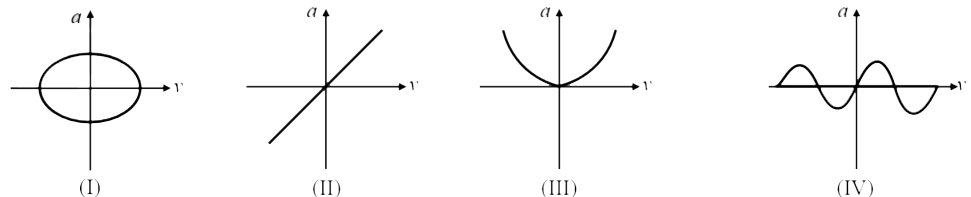 AIBIICIIIDIV
AIBIICIIIDIV
The tension in the string of a simple pendulum is maximum, when the bob of the pendulum
View SolutionThe velocity of the bob of a simple pendulum in the mean position is v. If its amplitude is doubled, by keeping the same length, then its velocity in the mean position will be
View SolutionThe periodic time of a simple pendulum is 3 sec. The mass of its hollow spherical bob is 100 gram. The bob is then filled with sand, so that the mass of the bob becomes 200 gram. Then the new period of oscillation of the simple pendulum wil be
View SolutionThe centre of gravity C.G. of the bob of a given simple pendulum is at position 'x' on earth. What is its new position on the surface of the moon?
View SolutionThe periodic time of a simple pendulum, oscillating in air is T. But if the bob of the simple pendulum is completely immersed in a nonviscous liquid, whose density is 120 th of the material of the bob, then its new period will be
View Solution
