The two uniform discs rotate separately on parallel axles. The upper disc (radius $a$ and momentum of inertia $I_{1}$ ) is given an angular velocity $ω_{0}$ and the lower disc of (radius $b$ and momentum of inertia $I_{2}$ ) is at rest. Now the two discs are moved together so that their rims touch. Final angular velocity of the upper disc is.
.

Video Solution

Text Solution

Verified by Experts

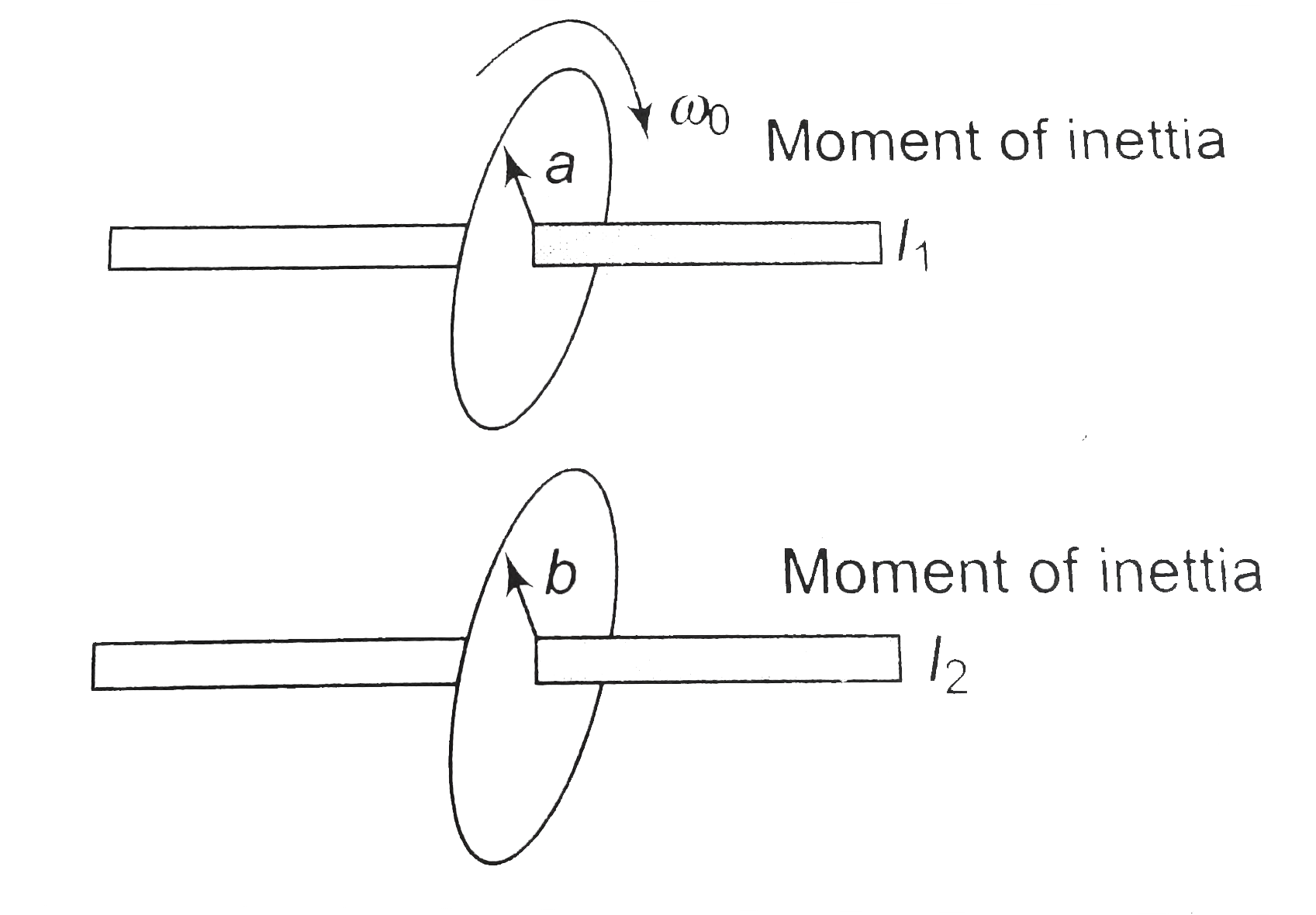The two discs exert equal and opposite forces on each other when in contact. The torque due to these forces change the angular momentum of each disc. Form angular impulse angular momentum theorem, we have

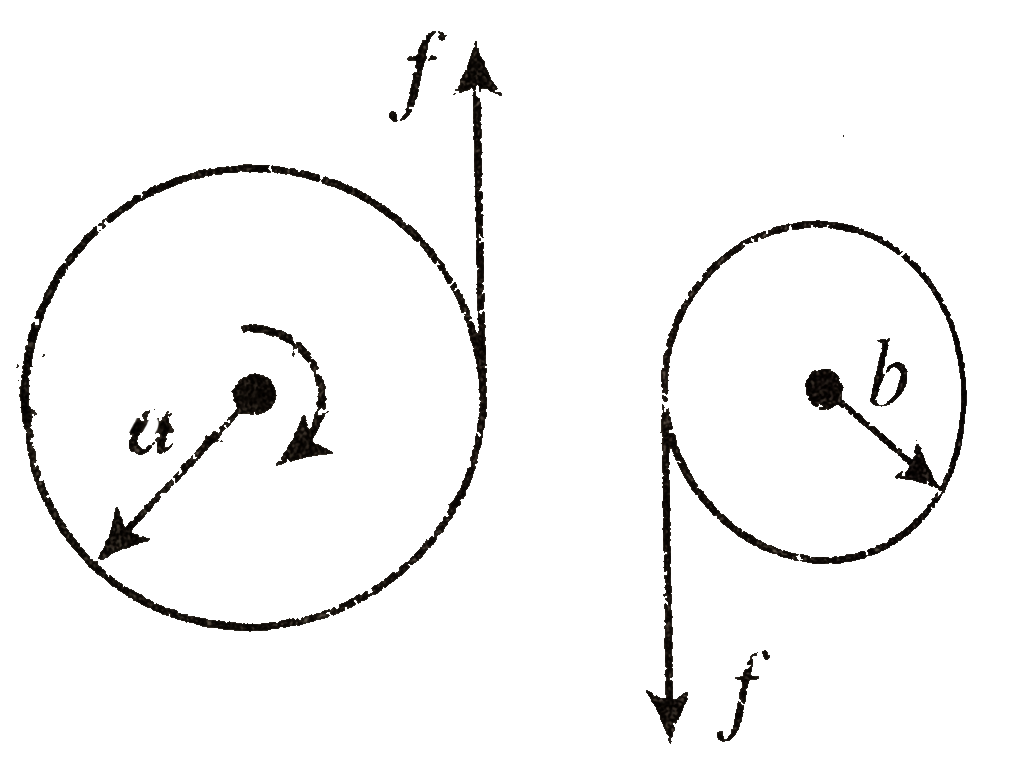
$f a △ t = I_{1} (ω_{0} - ω_{1})$
and $f b △ t = I_{2} ω_{2}$
From eqn i and ii we get
$\frac{a}{b} = \frac{I_{1} (ω_{0} - ω_{1})}{I_{2} ω_{2}}$
When slipping ceaes between the disc, the contact points the two dics have the same linear velocity i.e.,
$a ω_{1} = b ω_{2}$
on substituting $ω_{2}$ in eqn iii we get
$ω_{1} = \frac{(I_{1} ω_{0})}{[I_{1} + (a^{2} I_{2} / b^{2})]}$

Updated on:21/07/2023