The width of a river is 2√3 km. A boat is rowed in direction perpendicular to the banks of river. If the drift of the boat due to flow is 2 km, the displacement of the boat is
3 km
6 km
5 km
4 km
The correct Answer is:D
To solve the problem, we need to find the displacement of the boat as it crosses the river while being affected by the flow of the river. The width of the river and the drift due to the current will help us form a right triangle, where we can use the Pythagorean theorem to find the displacement.
1. Identify the given values:
- Width of the river (W) = 2√3 km
- Drift of the boat (D) = 2 km
2. Visualize the scenario:
- The boat is rowed straight across the river, which means it moves perpendicular to the banks.
- Due to the river's current, the boat drifts downstream while crossing.
3. Set up the right triangle:
- The width of the river (W) is one leg of the triangle.
- The drift (D) is the other leg of the triangle.
- The displacement (d) of the boat is the hypotenuse of the triangle.
4. Apply the Pythagorean theorem:
- According to the Pythagorean theorem:
d2=W2+D2
- Substitute the values:
d2=(2√3)2+(2)2
- Calculate W2 and D2:
(2√3)2=4×3=12
(2)2=4
- Now substitute these values back into the equation:
d2=12+4=16
5. Calculate the displacement (d):
- Take the square root of both sides:
d=√16=4 km
6. Conclusion:
- The displacement of the boat is 4 km.
Final Answer:
The displacement of the boat is 4 km.
---
- Width of the river (W) = 2√3 km
- Drift of the boat (D) = 2 km
- The boat is rowed straight across the river, which means it moves perpendicular to the banks.
- Due to the river's current, the boat drifts downstream while crossing.
- The width of the river (W) is one leg of the triangle.
- The drift (D) is the other leg of the triangle.
- The displacement (d) of the boat is the hypotenuse of the triangle.
- According to the Pythagorean theorem:
d2=W2+D2
- Substitute the values:
d2=(2√3)2+(2)2
- Calculate W2 and D2:
(2√3)2=4×3=12
(2)2=4
- Now substitute these values back into the equation:
d2=12+4=16
- Take the square root of both sides:
d=√16=4 km
- The displacement of the boat is 4 km.
The displacement of the boat is 4 km.
Similar Questions
The width of a river is 3√3 km. A boat has a velocity of √3 km/s and starts to cross the river in a direction perpendicular to the bank of the river. If the river is flowing with a velocity 2km/sec, find the distance drifted by the boat.
View SolutionWe know that when a boat travels in water, its net velocity w.r.t. ground is the vector sum of two velocities. First is the velocity of boat itself in river and other is the velocity of water w.r.t. ground. Mathematically:
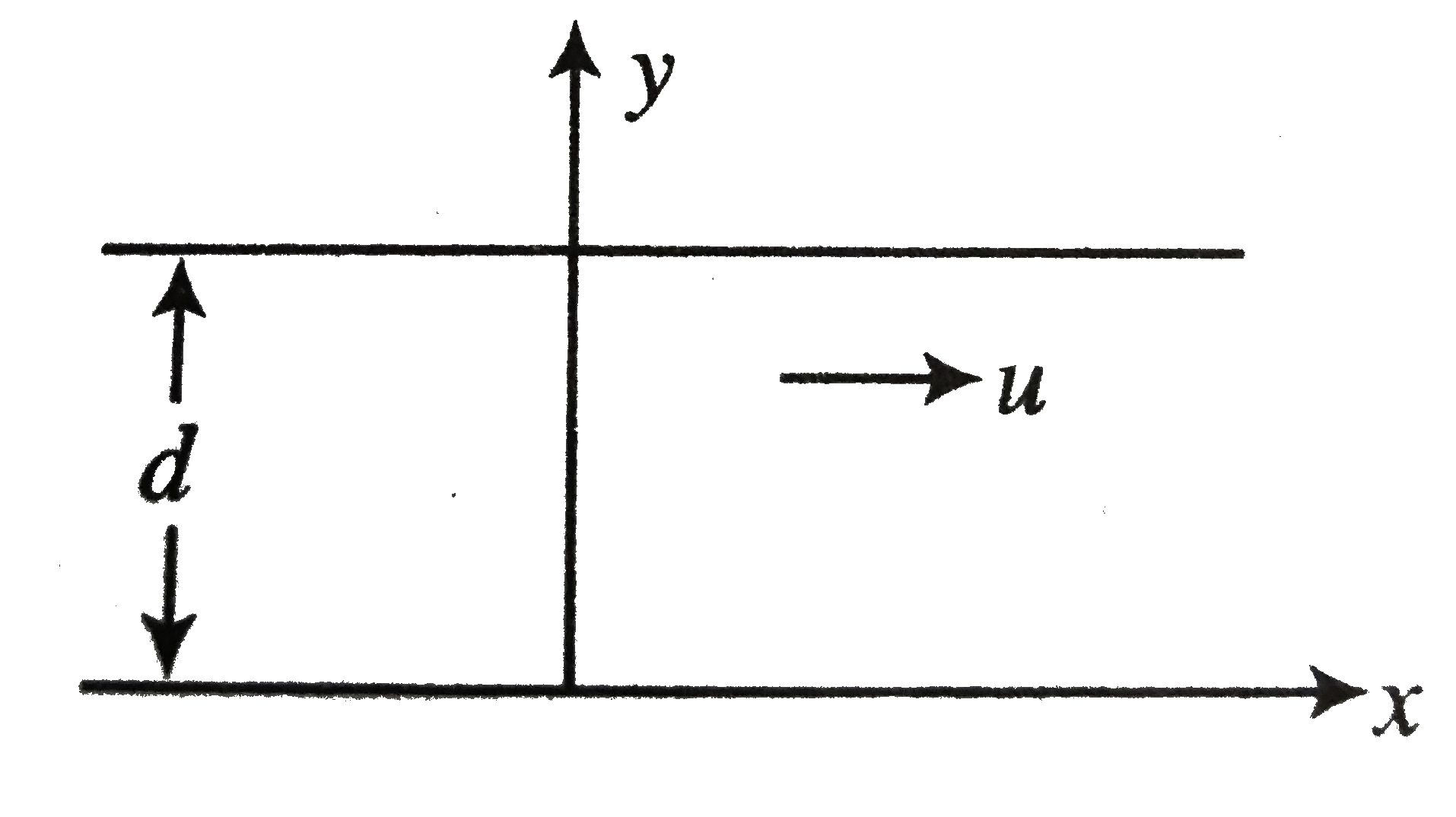
→vboat=→vboat,water+→vwater.
Now given that velocity of water w.r.t. ground in a river is u. Width of the river is d.
A boat starting from rest aims perpendicular to the river with an acceleration of a = 5t, where t is time. The boat starts from point (1,0) of the coordinate system as shown in figure. Assume SI units.
View SolutionWe know that when a boat travels in water, its net velocity w.r.t. ground is the vector sum of two velocities. First is the velocity of boat itself in river and other is the velocity of water w.r.t. ground. Mathematically:

→vboat=→vboat,water+→vwater.
Now given that velocity of water w.r.t. ground in a river is u. Width of the river is d.
A boat starting from rest aims perpendicular to the river with an acceleration of a = 5t, where t is time. The boat starts from point (1,0) of the coordinate system as shown in figure. Assume SI units.
View SolutionThe current velocity of river grows in proportion to the distance from its bank and reaches the maximum value v0 in the middle. Near the banks the velocity is zero. A boat is moving along the river in such a manner that the boatman rows his boat always perpendicular to the current. The speed of the boat in still water is u. Find the distance through which the boat crossing the river will be carried away by the current, if the width of the river is c. Also determine the trajectory of the boat.
View SolutionWe know that when a boat travels in water, its net velocity w.r.t. ground is the vector sum of two velocities. First is the velocity of boat itself in river and other is the velocity of water w.r.t. ground. Mathematically:
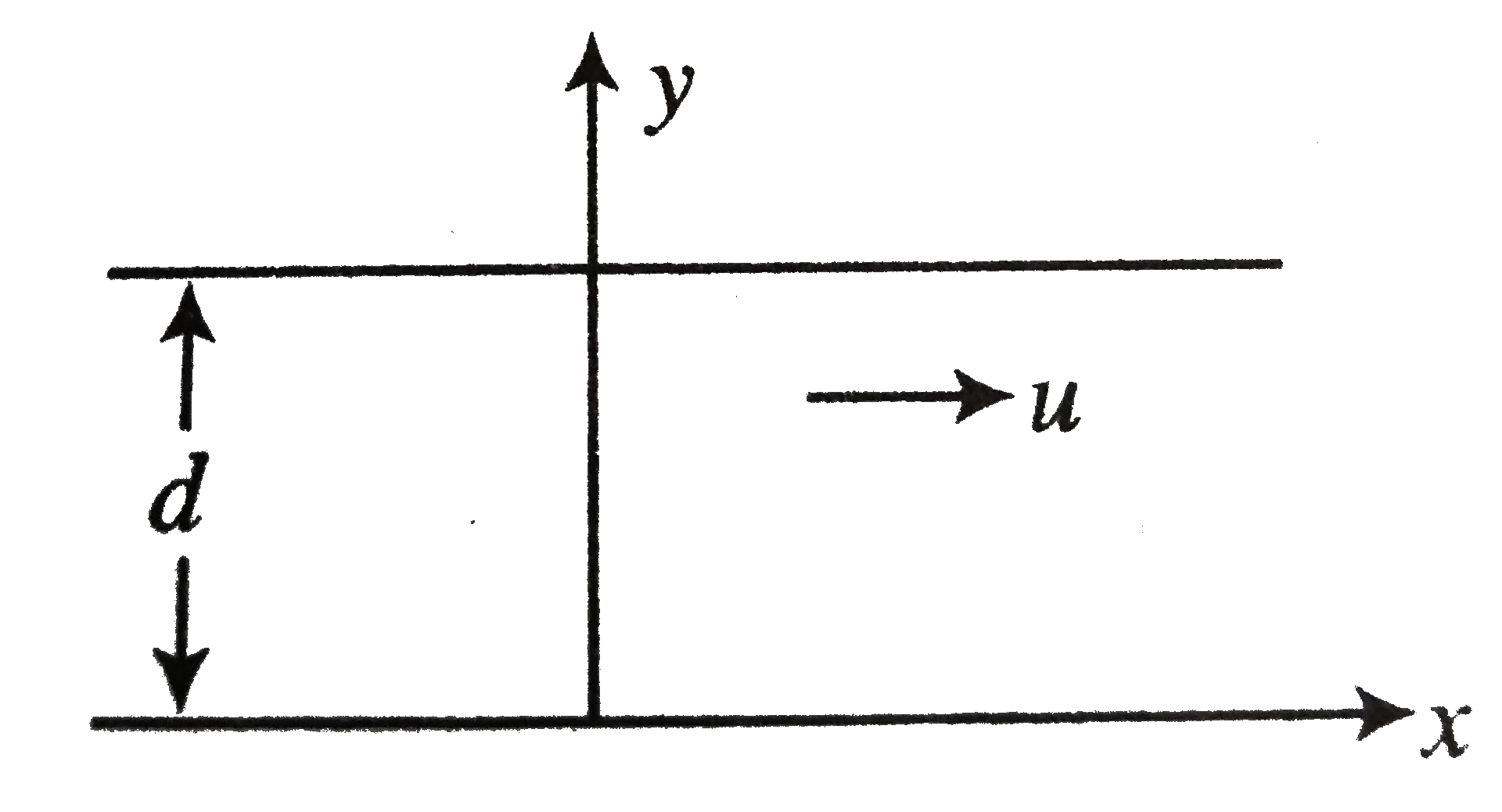
→vboat=→vboat,water+→vwater.
Now given that velocity of water w.r.t. ground in a river is u. Width of the river is d.
A boat starting from rest aims perpendicular to the river with an acceleration of a = 5t, where t is time. The boat starts from point (1,0) of the coordinate system as shown in figure. Assume SI units. Find time taken by him to across the river.
View SolutionA 400m wide river is flowing at a rate of 2.0ms−1. A boat is sailing with a velocity of 10ms−1 with respect to the water, in a direction perpendicular to the river.
(a) Find the time taken by the boat to reach the opposite bank.
(b) How far from the point directly opposite to the starting point does the boat reach the opposite bank ?
( c) In what direction does the boat actually move ?
View SolutionA river 400m wide is flowing at a rate of 2.0m/s. A boat is sailing at a velocity of 10.0m/s with respect to the water In a direction perpendicular to the river.
(a) Find the time taken by the boat to reach the opposite bank.
(b) How far from the point directly opposite to the starting point does the boat reach the opposite bank?
View SolutionA boat can be rowed at 5 m/s on still water. It is used to cross a 200m wide river from the south bank to the north bank. The river current has uniform velocity of 3 m/s due east.
(a) In which direction must it be steered to cross the river perpendicular to current ?
(b) How long will it take to cross the river in a direction perpendicular to the river flow ?
(c) In which direction must the boat be steered to cross the river in minimum time ? How far will it drift ?
View SolutionA river 500m wide is flowing at a rate of 4m/s. A boat is sailing at a velocity of 10m/s with respect to the water, in a direction perpendicular to the river. The time taken by the boat to reach the opposite bank
View SolutionA boat is rowed across a river at the rate of 4.5kmhr. The river flows at the rate of 6kmhr. The velocity of boat in ms is:
View Solution
