The x-component of the resultant of several vectors
is equal to the sum of the x-components of the vectors
may be smaller than the sum of the magnitudes of the vectors
may be greater than the sum of the magnitude of the vectors
may be equal to the sum of the magnitude of the vectors.
The correct Answer is:A, B, D
To solve the question regarding the x-component of the resultant of several vectors, we will analyze the options provided step by step.
Step 1: Understanding the x-component of the resultant vector
The x-component of the resultant vector from multiple vectors is determined by summing the x-components of each individual vector.
Step 2: Analyzing Option 1
Option 1 states: "The x-component of the resultant vector is equal to the sum of the x-components of the vectors."
- If we have vectors A and B, with components Ax and Bx respectively, the x-component of the resultant vector Rx can be expressed as:
Rx=Ax+Bx
- This is true for any number of vectors. Thus, Option 1 is correct.
Step 3: Analyzing Option 2
Option 2 states: "The x-component of the resultant vector may be smaller than the sum of the magnitudes of the vectors."
- The magnitude of a vector is given by the square root of the sum of the squares of its components. Hence, the magnitude of vector A is:
|A|=√A2x+A2y
- The sum of the magnitudes of the vectors can be greater than the x-component of the resultant if the vectors are not aligned in the same direction. Thus, Option 2 is correct.
Step 4: Analyzing Option 3
Option 3 states: "The x-component of the resultant vector may be greater than the sum of the magnitudes of the vectors."
- This statement is false because the x-component of the resultant can never exceed the sum of the magnitudes of the vectors. The x-component is just one part of the vector, while the magnitude considers all components. Thus, Option 3 is incorrect.
Step 5: Analyzing Option 4
Option 4 states: "The x-component of the resultant vector may be equal to the sum of the magnitudes of the vectors."
- This can occur in a special case where all vectors are aligned along the x-axis. For example, if vector A has a magnitude of 3 and vector B has a magnitude of 4, and both are in the positive x-direction, then:
Rx=A+B=3+4=7
- In this case, the x-component of the resultant vector equals the sum of the magnitudes. Thus, Option 4 is correct.
Conclusion
The correct options are:
- Option 1: Correct
- Option 2: Correct
- Option 3: Incorrect
- Option 4: Correct
Summary of Correct Options
The correct statements regarding the x-component of the resultant vector are Options 1, 2, and 4.
---
The x-component of the resultant vector from multiple vectors is determined by summing the x-components of each individual vector.
Option 1 states: "The x-component of the resultant vector is equal to the sum of the x-components of the vectors."
- If we have vectors A and B, with components Ax and Bx respectively, the x-component of the resultant vector Rx can be expressed as:
Rx=Ax+Bx
- This is true for any number of vectors. Thus, Option 1 is correct.
Option 2 states: "The x-component of the resultant vector may be smaller than the sum of the magnitudes of the vectors."
- The magnitude of a vector is given by the square root of the sum of the squares of its components. Hence, the magnitude of vector A is:
|A|=√A2x+A2y
- The sum of the magnitudes of the vectors can be greater than the x-component of the resultant if the vectors are not aligned in the same direction. Thus, Option 2 is correct.
Option 3 states: "The x-component of the resultant vector may be greater than the sum of the magnitudes of the vectors."
- This statement is false because the x-component of the resultant can never exceed the sum of the magnitudes of the vectors. The x-component is just one part of the vector, while the magnitude considers all components. Thus, Option 3 is incorrect.
Option 4 states: "The x-component of the resultant vector may be equal to the sum of the magnitudes of the vectors."
- This can occur in a special case where all vectors are aligned along the x-axis. For example, if vector A has a magnitude of 3 and vector B has a magnitude of 4, and both are in the positive x-direction, then:
Rx=A+B=3+4=7
- In this case, the x-component of the resultant vector equals the sum of the magnitudes. Thus, Option 4 is correct.
The correct options are:
- Option 1: Correct
- Option 2: Correct
- Option 3: Incorrect
- Option 4: Correct
The correct statements regarding the x-component of the resultant vector are Options 1, 2, and 4.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises34videos
PHYSICS AND MATHEMATICS
- BOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Question for short Answer14videos
PHYSICS AND MATHEMATICS
- BOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Objective 16videos
PHYSICS AND MATHEMATICS
- BOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Questions for short Answer17videos
NEWTON'S LAWS OF MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer13videos
REST AND MOTION : KINEMATICS
Similar Questions
Three co-planar vectors (x−y plane ) having magnitude 3√2,6,10 are shown in the figure. Find the componenent of resultant of three vectors along the x axis as shown.
 .View Solution
.View SolutionThe component of a vector is
View SolutionFind the magnitude and direction of the resultant of given vectors
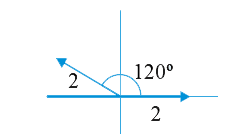 View Solution
View SolutionCan the resultant of two vector be zero
View SolutionThe component of ˆi in the direction of vector ˆi+ˆj+2ˆk is
View SolutionX- component of →a is twice of its Y- component. If the magnitude of the vector is 5√2 and it makes an angle of 135∘ with z-axis then the components of vector is:
View SolutionThe maximum and minimum magnitudes of the resultant of two vectors are 23 units and 7 units respectively. If these two vectors are acting at right angles to each other, the magnitude of their resultant will be
View SolutionThe maximum and minimum magnitudes of the resultant of two vectors are 23 units and 7 units respectively. If these two vectors are acting at right angles to each other, the magnitude of their resultant will be
View SolutionThe maximum and minimum magnitude of the resultant of two given vectors are 17 units and 7 unit respectively. If these two vectors are at right angles to each other, the magnitude of their resultant is
View SolutionThe angle between two vector A and B is θ. Vector R is the resultant of the two vectors. If R makes an angle θ2 with A, then
View Solution

