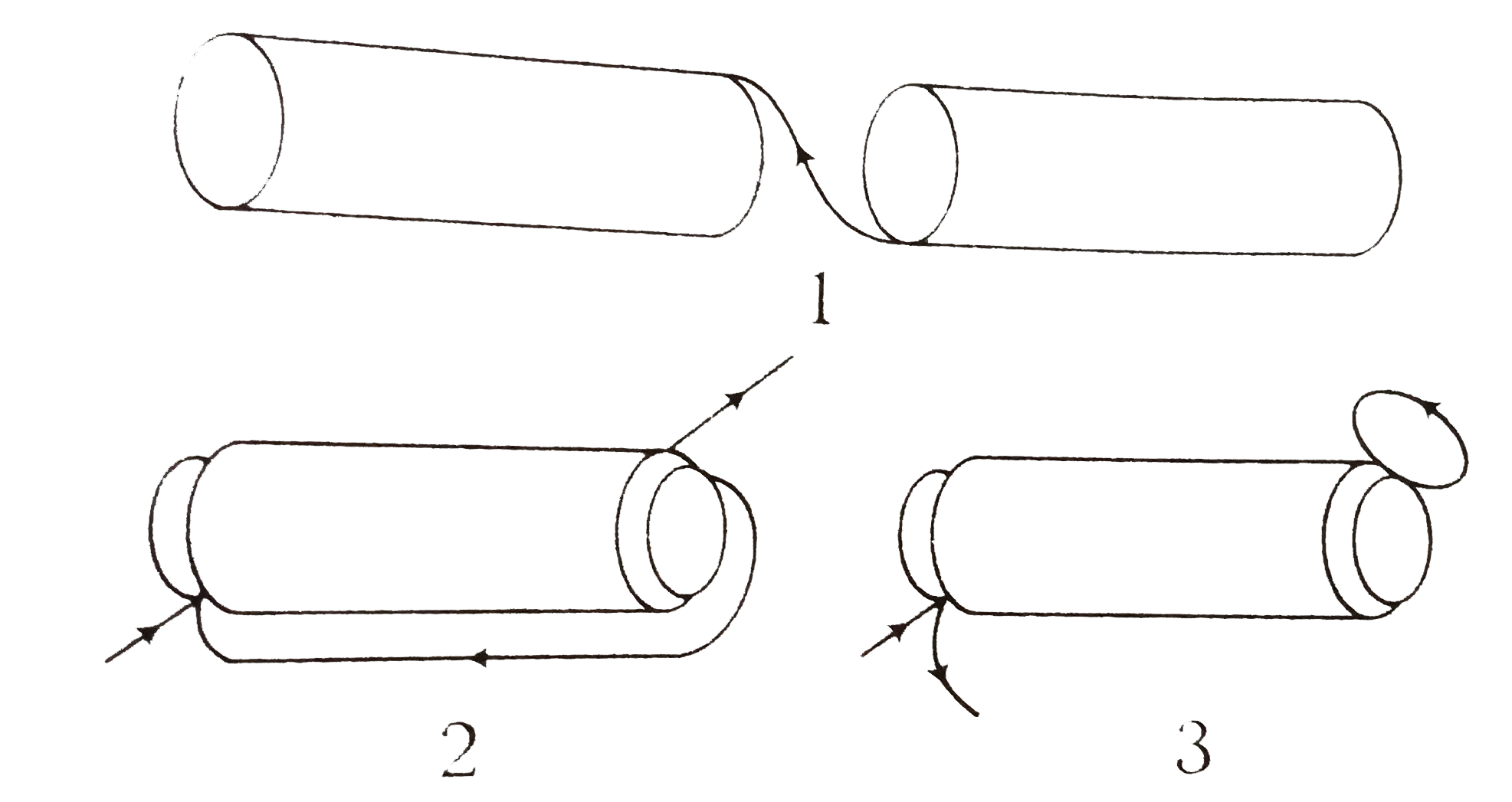
There are two solenoid of same length and inductance L but their diameters differ to the extent that one can just fit into the other. They are connected in three different ways in series.
(1) They are connected in series but separated by large distance.
(2) They are connected in series with one inside the other and senses of the turns coinciding.
(3) Both are connected in series with one inside the other with senses of the turns opposites,
as depicted in figures 1, 2 and 3 respectively. The total inductance of the solenoids in each of the case 1, 2 and 3 are respectively.
A
0,4L0,2L0
B
4L0,2L0,0
C
2L0,0,4L0
D
2L0,4L0,0
Video Solution
Text Solution
The correct Answer is:D
When two solenoids of inductance L0 are connected in series at large distance and current i is passed through them, then the totat flux linkage ϕtotal=L0i+L0i.
IF L be the equivalent inductance of the system, then
ϕtotal=Li
∴ Li=L0i+L0iorL=2L0
When solenoids are connected in series with one inside the other and senses of the turns coinciding, then there will be a mutual inductance L between them. In this case, the resultant induced emf in the coils is the sum of the emf's e1 and e2 in the respective coils, i.e.,
e=e1+e2
=(−L0didt±L0didt)+(−L0didt±L0didt)
where, (+) sign is for positive coupling and (-) sign for negative coupling.
But, e=−Ldidt
∴ −Ldidt=−L0didt−L0didt±2L0didt
i.e., L=L0+L0+2L0
=4L0 [for positive coupling ]
When solenoids are connected in series with one inside the other with senses of the turn opposite, then there is a negative coupling.
So, L=L0+L0−2L0=0
|
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrance special format questions
17 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Match the columns
5 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Check point
60 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
Knowledge Check
When the resistors are connected in series, then
Acurrent through each resistor is same as the total current in the circuitBvoltage across each resistor is same as the total voltage in the circuitCthe sum of the current passing through each resistor is same as the total current in the circuitDthe effective resistance is less than the smallest of the Individual resistanceThree different capacitors are connected in series then
Athey will have equal chargesBhey will have same potentialCboth (a) and (b)Dnone of theseIf the number of condensers are connected in series then
Acharge on each condenser is same and potential is differentBpotential is same but charge is differentCboth charge and potential is sameDboth charge and potential is different
Which electrical elements are connected in series
View SolutionThe same capacitors are connected in series. Find the resultant capacitance.
View SolutionAn inductance and a resistance are connected in series with an AC potential . In this circuit
View SolutionTwo pure inductors each of self inductance L are connected in series, the net inductance is
View SolutionThree wires each have resistance 2Ω, if we connect 2 in series with one parallel to the combination the equivalent resistance is
View Solution