There is a tower of height 20m and a particle is projected up from top of the tower with an initial speed 20m//s. Top of the tower is marked as point A, from where particle is projected. Point of maximum height a denoted as B. When particle reaches the point A during downward journey then we call the same point as C. Point at the bottom of tower is marked as D where particle finally strikes. Acceleration due to gravity g=10m/s2
Time taken by the particle to reach the ground is
2s
4s
2√2s
(2+2√2)s
The correct Answer is:d
To solve the problem of a particle projected from the top of a tower, we will follow these steps:
Step 1: Understand the problem
We have a tower of height h=20m and a particle is projected upwards from the top of the tower with an initial speed u=20m/s. The acceleration due to gravity is g=10m/s2. We need to find the time taken for the particle to reach the ground (point D).
Step 2: Set up the equation of motion
We will use the second equation of motion:
s=ut+12at2
Where:
- s is the displacement,
- u is the initial velocity,
- a is the acceleration,
- t is the time.
Step 3: Define the signs
Since we are considering the downward direction as negative:
- Displacement s=−20m (from point A to point D),
- Initial velocity u=20m/s (upwards, so positive),
- Acceleration a=−10m/s2 (downwards, due to gravity).
Step 4: Substitute values into the equation
Substituting the values into the equation:
−20=20t−12(10)t2
This simplifies to:
−20=20t−5t2
Rearranging gives us:
5t2−20t−20=0
Step 5: Simplify the equation
Dividing the entire equation by 5:
t2−4t−4=0
Step 6: Solve the quadratic equation
Using the quadratic formula t=−b±√b2−4ac2a:
Here, a=1,b=−4,c=−4:
t=−(−4)±√(−4)2−4⋅1⋅(−4)2⋅1
t=4±√16+162
t=4±√322
t=4±4√22
t=2±2√2
Step 7: Determine the positive time
Since time cannot be negative, we take the positive solution:
t=2+2√2seconds
Final Answer
The time taken by the particle to reach the ground is t=2+2√2seconds.
---
We have a tower of height h=20m and a particle is projected upwards from the top of the tower with an initial speed u=20m/s. The acceleration due to gravity is g=10m/s2. We need to find the time taken for the particle to reach the ground (point D).
We will use the second equation of motion:
s=ut+12at2
Where:
- s is the displacement,
- u is the initial velocity,
- a is the acceleration,
- t is the time.
Since we are considering the downward direction as negative:
- Displacement s=−20m (from point A to point D),
- Initial velocity u=20m/s (upwards, so positive),
- Acceleration a=−10m/s2 (downwards, due to gravity).
Substituting the values into the equation:
−20=20t−12(10)t2
This simplifies to:
−20=20t−5t2
Rearranging gives us:
5t2−20t−20=0
Dividing the entire equation by 5:
t2−4t−4=0
Using the quadratic formula t=−b±√b2−4ac2a:
Here, a=1,b=−4,c=−4:
t=−(−4)±√(−4)2−4⋅1⋅(−4)2⋅1
t=4±√16+162
t=4±√322
t=4±4√22
t=2±2√2
Since time cannot be negative, we take the positive solution:
t=2+2√2seconds
The time taken by the particle to reach the ground is t=2+2√2seconds.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE (ASSERTION REASON)9videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE (MATCHING TYPE QUESTIONS)2videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE ( C.(MULTIPLE CHOICE QUESTIONS))13videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test15videos
MOTION IN A PLANE
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)12videos
OSCILLATIONS
Similar Questions
There is one tower of height h. one particle A is projected upward from top of the tower with a speed u1 at time t=0 . At time t=t0 another particle B is projected upward from the bottom of the tower with a speed u2. When will the particles cross each other?
View SolutionA particle is dropped from the top of a tower. It covers 40 m in last 2s. Find the height of the tower.
View Solution
Knowledge Check
- Question 1 - Select One
There is a tower of height 20m and a particle is projected up from top of the tower with an initial speed 20m//s. Top of the tower is marked as point A, from where particle is projected. Point of maximum height a denoted as B. When particle reaches the point A during downward journey then we call the same point as C. Point at the bottom of tower is marked as D where particle finally strikes. Acceleration due to gravity g=10m/s2
How much time the particle takes to cross the point C after being projected from point A.A1sB2sC3sD4s - Question 2 - Select One
There is a tower of height 20m and a particle is projected up from top of the tower with an initial speed 20m//s. Top of the tower is marked as point A, from where particle is projected. Point of maximum height a denoted as B. When particle reaches the point A during downward journey then we call the same point as C. Point at the bottom of tower is marked as Dwhere particle finally strikes. Acceleration due to gravity g=10m/s2
Maximum height above the ground attained by particle isA20mB30mC40mD60m - Question 3 - Select One
At a point 20 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘ The height of the tower is
A20√3mB20√3mC√320mDNone of these
Height of a tower is 125m and a particle is dropped from rest from the top of the tower. After two seconds of its fall, another particle is projected downwards with a speed u such that both the particles reach the ground simultaneously. What is the value of u?
View SolutionA particle is dropped from the top of a tower. If it falls half of the height of the tower in its last second of journey, find the time of journey.
View SolutionA particle is thrown horizontally from a tower of height 20m. If the speed of projection is 20ms then the distance of point from the tower,where the particle hit the ground is:-
View SolutionA particle is dropped from the top of a tower. During its motion it covers 925 part of height of tower in the last 1 second Then find the height of tower.
View SolutionSuppose angle of depression from top of the tower to point A is 45∘ and height of tower is 10 m. What is the distance of point A from the building?
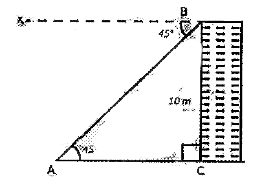 View Solution
View Solution

