Three balls of same masses are projected with equal speeds at angle 15∘,45∘,75∘, and their ranges are respectively R1,R2 and R3, then
R1>R2>R3
R1<R2<R3
R1=R2=R3
R1=R3<R2
The correct Answer is:D
To solve the problem, we need to find the relationship between the ranges R1,R2,R3 of three balls projected at angles 15∘,45∘, and 75∘ respectively. We will use the formula for the range of a projectile:
R=u2sin2θg
where:
- R is the range,
- u is the initial speed,
- θ is the angle of projection,
- g is the acceleration due to gravity.
Step 1: Calculate R1 for θ1=15∘
Using the range formula:
R1=u2sin2(15∘)g
Calculating sin2(15∘):
sin2(15∘)=sin30∘=12
Thus,
R1=u2⋅12g=u22g
Step 2: Calculate R2 for θ2=45∘
Using the range formula:
R2=u2sin2(45∘)g
Calculating sin2(45∘):
sin2(45∘)=sin90∘=1
Thus,
R2=u2⋅1g=u2g
Step 3: Calculate R3 for θ3=75∘
Using the range formula:
R3=u2sin2(75∘)g
Calculating sin2(75∘):
sin2(75∘)=sin150∘=sin(180∘−30∘)=sin30∘=12
Thus,
R3=u2⋅12g=u22g
Step 4: Compare the ranges
From our calculations, we have:
- R1=u22g
- R2=u2g
- R3=u22g
Now, we can see that:
R1=R3
And since R2 is greater than both R1 and R3:
R2>R1andR2>R3
Conclusion
The relationship between the ranges is:
R1=R3<R2
- R is the range,
- u is the initial speed,
- θ is the angle of projection,
- g is the acceleration due to gravity.
- R2=u2g
- R3=u22g
Topper's Solved these Questions
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - B. Medical entrance
1 Videos
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - Medical entrance
12 Videos
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - A. Taking it together
1 Videos
- MEASUREMENT AND ERRORSBOOK - DC PANDEY ENGLISHCHAPTER - MEASUREMENT AND ERRORSEXERCISE - Subjective
19 Videos
- MOTION IN A PLANEBOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery
32 Videos
Similar Questions
Two bodies of same mass are projected with the same velocity at an angle 30∘ and 60∘ respectively.The ration of their horizontal ranges will be
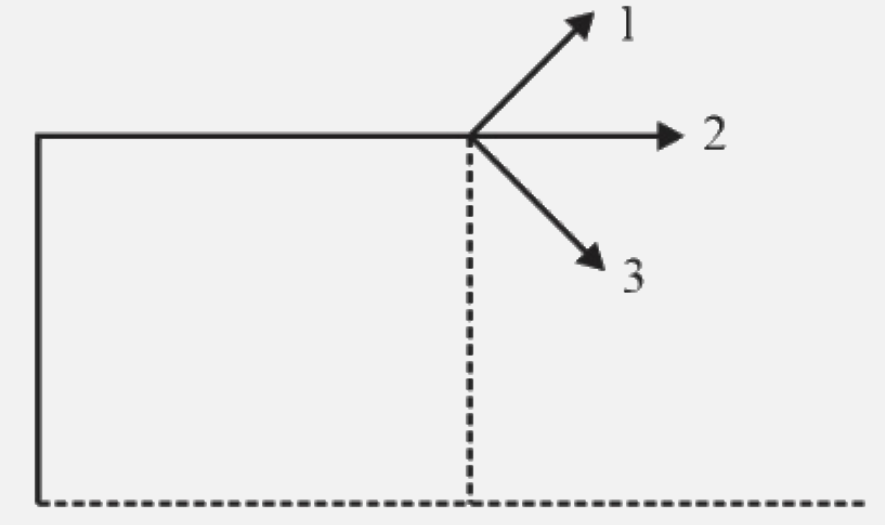
View SolutionThree balls are projected from the top of a building with equal speed but at difference angles. Balls strike the ground velocities v1,v2andv3 respectively , then
View SolutionFour projectiles are projected with the same speed at angles 20∘,35∘,60∘and75∘
with the horizontal. The range will be the maximum for the projectile whose angle of projection is
View SolutionTwo balls of equal mass are projected from a tower simultaneously with equal speeds. One at angle θ above the horizontal and the other at the same angle θ below the horizontal. The path of the center of mass of the two balls is
View SolutionThree planets of same density have radii R1,R2 and R3 such that R1=2R2=3R3. The gravitational field at their respective surfaces are g1,g2 and g3 and escape velocities from their surfeces are υ1,υ2 and υ3, then
View SolutionTwo particle are projected from an incliend plane as shown. A is projected up the incline and B down the incline. If RA and RB are range of A and B respectively then RBRA will be equal to :

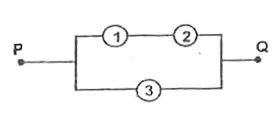
View SolutionThree ammeters 1, 2, and 3 of resistances R1.R2andR3 respectively are joined as shown. When some potential difference is applied across the terminals P and Q, their readings are I1,I2andI3, respectively. Then
View SolutionA ball is projected with speed u at an angle θ to the horizontal. The range R of the projectile is given by
R=u2sin2θg
for which value of θ will the range be maximum for a given speed of projection? (Here g= constant)
View SolutionTwo particles are projected obliquely from ground with same speed such that their range 'R' are same but they attain different maximum heights h1 and h2 then relation between R,h1 and h2 is:
View SolutionTwo particles are projected obliquely from ground with same speed such that their range 'R' are same but they attain different maximum heights h1 and h2 then relation between R,h1 and h2 is:
View Solution
