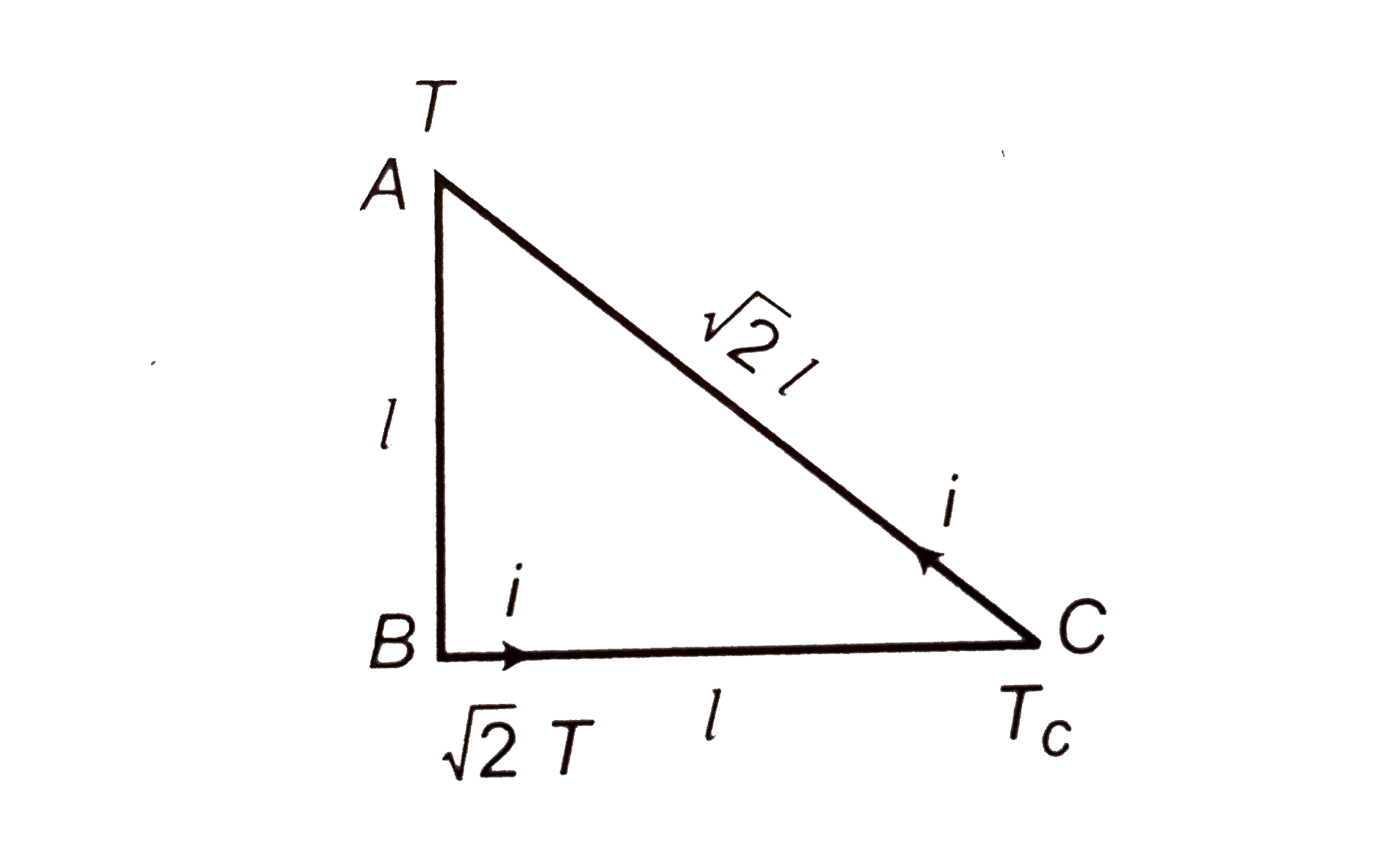
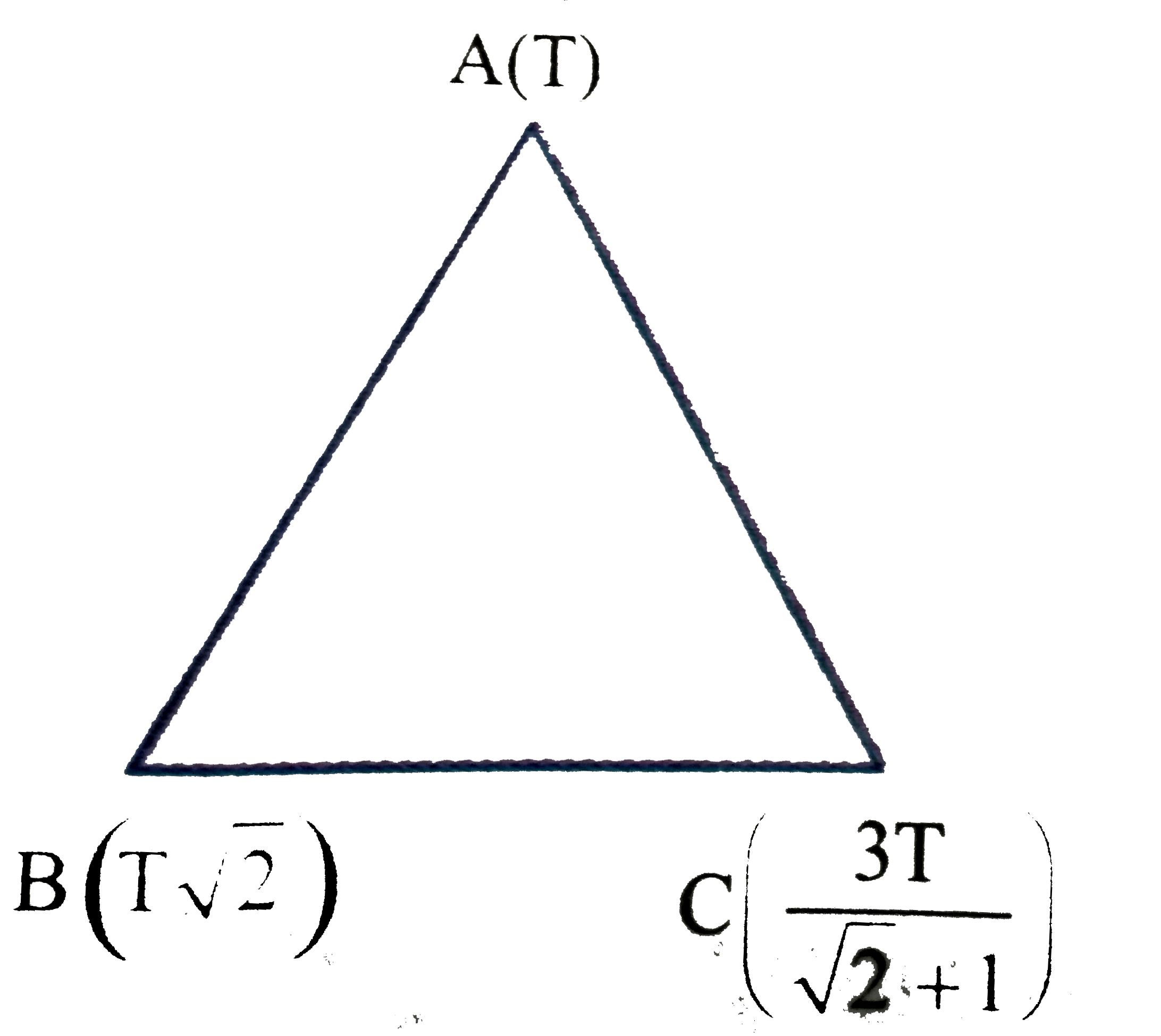
Three rods of identical cross-sectional area and made from the same metal from the sides of an isosceles triangle ABC, right-angled at B. The point A and B are maintained at temperatures T and √2 T respectively. In the steady state, the temperature of the point C is Tc. Assuming that only heat conduction takes places, Tc/T is
1(√2+1)
2(√2+1)
12(√2−1)
1√3(√21)
The correct Answer is:B
(2) RAB=RBC=R
RAC=√2R
Since TB>TA, heat will flow from B to A through two paths BA and BCA
In path BCA, current will be same in BC and CA
i=√2T−TCRBC=TC−TRAC
√2T−TCR=TC−T√2R
2T−2√2TC=TC−T
(√2+1)TC=3T
TCT=3(√2+1)
Topper's Solved these Questions
Similar Questions
Three rods of same cross sectional area are made of same material and form the sides of a right-angled isosceles triangle PQR, right angled at Q. P and Q are maintained at temperature T and 2T, respectively. If the temperature of end R is T', then what is the ratio of T'/T. Assume that only heat conduction takes place.
View SolutionFour rods of identical cross-sectional area and made from the same metal form the sides of square. The temperature of two diagonally opposite points are T and√2T respectively in the steady state. Assuming that only heat conduction takes place, what will be the temperature difference between other two points
View Solution
Knowledge Check
Three rods of identical area of cross-section and made from the same metal from the sides of an isosceles triangle. ABC, right angled at B. The points A and B are maintained at temperatures T and √2T respectively. In the steady state the temperature of the point C is TC.
Assuming that only heat conduction takes place , TCT is equal toA1√2+1B3√2+1C12(2√2−1)D1√3(√2−1)Three rods of identical cross-sectional area and made from the same metal form the sides of an equilateral triangle ABC The points A and B are maintained at temperature √3T and T respectively In the steady state, the temperature of the point C is TC Assuming that only heat conduction takes place, the value of TC/T is equal to .
A1+√32B−1√32C1+√22D1−√22Three rods of identical cross-sectional area and made from the same metal form the sides of an insosceles triangle ABC right angled at B The pints A and B are maintained at temperatures T and √T respectively in the steady state Assuming that only heat conduction takes place, temperature of point C is .
 .A3T√2+1BT√2+1CT3(√2−1)DT√2−1
.A3T√2+1BT√2+1CT3(√2−1)DT√2−1
Three rods of identical cross-sectional area and made from the same metal form the sides of an isosceles triangle ABC right angled at B as shown in the figure. The points A and B are maintained at temperature T and (√2)T respectively in the steady state. Assuming that only heat conduction takes place, temperature of point C will be

View Solution
Three rods of identical cross-sectional area and made from the same metal form the sides of an isosceles Delta ABC right angled at B. The points A and B are maintained at temperetures T and √2T, respectively in the steady state. Assuming that only heat conduction takes place, temperature of point C is
View SolutionThree rods of identical cross-sectional area and made from the same metal form the sides of an isosceles triangle ABC right angled at B as shown in the figure. The points A and B are maintained at temperature T and (√2)T respectively in the steady state. Assuming that only heat conduction takes place, temperature of point C will be

View SolutionThree rods of identical cross-sectional area and made from the same metal, from the sides of an isosceles triangle ABC right angled at B, as shown in Fig .7(CF).23. The points A and B are maintained at temperature T and √2T respectively in the steady. Assuming that only heat conduction takes place, temperature of point C will be
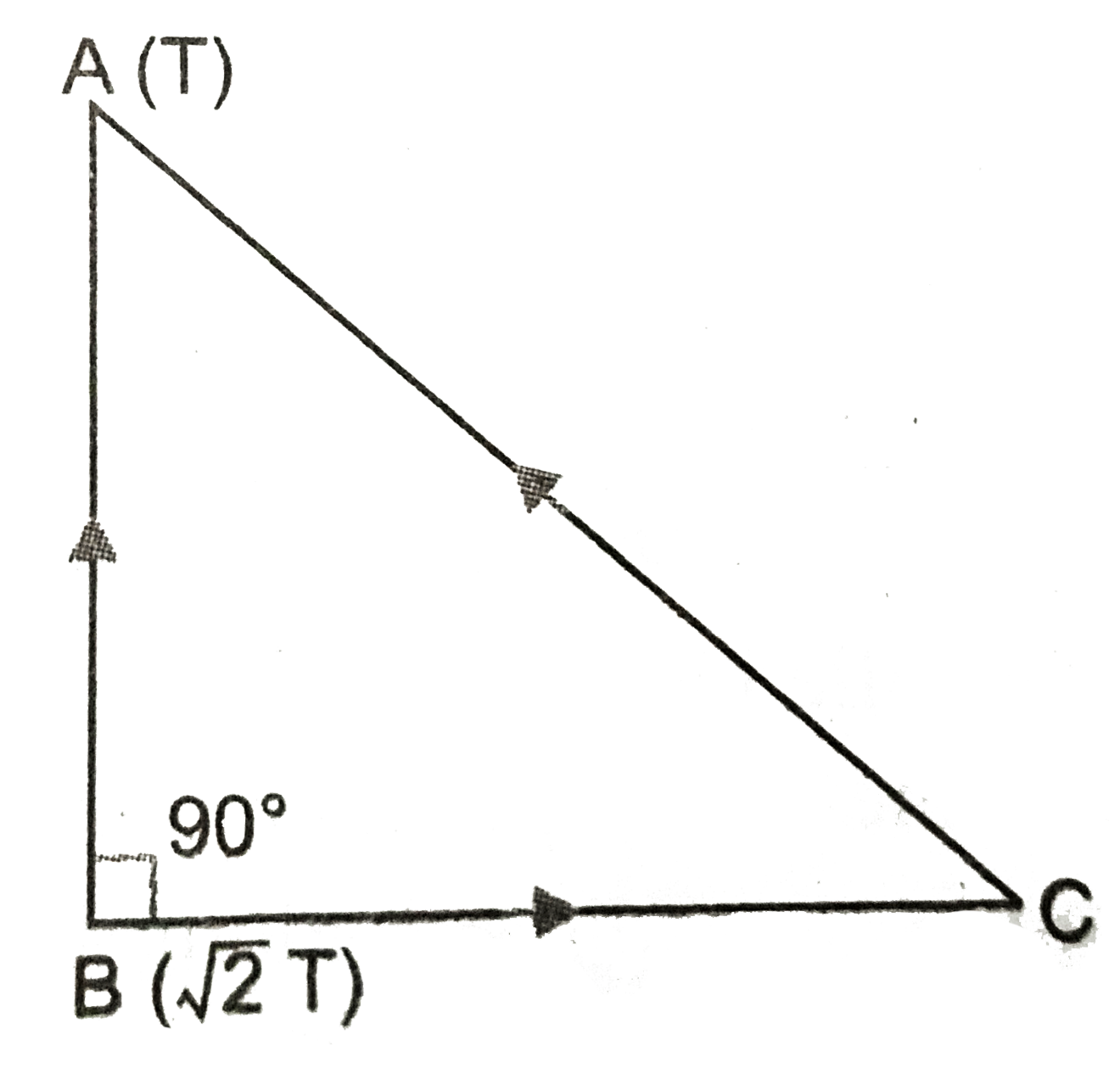
View SolutionThe three rods of same meterial and crosssectional area from the sides of a triangle ABC The points A,B and C are maintained at temperature TT√2 and 3T(√2+1) respectively Assuming that only heat conducting takes place the system is in steady state, find the angle at B The temperature difference per unit length along SB and CA is equal
 .
.
View Solution
