Two blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on one of the blocks pulling it away from the other as shown in the Fig. (i) Find the position of the centre of mass at time t. (ii) If the extension of the spring is x0 at time t, find the displacement of the two blocks at thin instant.

(i)Ft24m,(ii)x1=12(Ft22m−x0),x2=12(Ft22m+x0)
Topper's Solved these Questions
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - Hots Numerical Problems ( Based on Equilibrium of Force )
2 Videos
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - Hots Numerical Problems ( Based on Centre of Gravity )
2 Videos
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - Problem Set -II ( Miscellaneous )
1 Videos
- SIMPLE HARMONIC MOTIONBOOK - CHHAYA PUBLICATIONCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - CBSE SCANNER
16 Videos
- SUPERPOSITION OF WAVESBOOK - CHHAYA PUBLICATIONCHAPTER - SUPERPOSITION OF WAVESEXERCISE - CBSE Scanner
14 Videos
Similar Questions
Two blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on one of the blocks pulling it away from the other as shown in figure. (a) Find the displacement of the centre of mass at time t. (b) If the extension of the spring is x0 at time t, find the displacement of the two blocks at this instant.

View SolutionTwo blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on one of the blocks pulling it away from the other as shown in figure. (a) Find the displacement of the centre of mass at time t. (b) If the extension of the spring is x0 at time t, find the displacement of the two blocks at this instant.

View Solution
Knowledge Check
Two blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block pulling away from the other as shown in Fig.

Then the displacement of the centre of mass in at time t isAFt22mBFt23mCFt24mDFt2mTwo blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block pulling away from the other as shown in Fig.

If the extension of the spring is x0 at time t, then the displacement of the first block at this instant isA12(Ft22m+x0)B−12(Ft22m+x0)C12(Ft22m−x0)DFt22m+x0Two blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block pulling away from the other as shown in Fig.

If the extension of the spring is x0 at time t, then the displacement of the second block at this instant isAFt22m−x0B12(Ft22m+x0)C12(2F2m−x0)D12(Ft22m−x0)
Two block of equal mass m are connected by an unstretched spring and the sytem is kept at rest on a frictionless horizontal surface. A constant force F is applied on one of the blocks puling it away from the other as shown in figure. a.Find the positon of the centre of mass at time t. b.If the extansion of the spring is x0 at time t find the displacement of the two blocks at this instant.
View SolutionTwo block of equal mass m are connected by an unstretched spring and the sytem is kept at rest on a frictionless horizontal surface. A constant force F is applied on one of the blocks puling it away from the other as shown in figure. a.Find the positon of the centre of mass at time t. b.If the extansion of the spring is x0 at time t find the displacement of the two blocks at this instant.
View SolutionTwo blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block pulling away from the other as shown in Fig.

Then the displacement of the centre of mass in at time t is
View Solutiontwo blocks of equal mass m are connected by an unstretched· spring and the system is kept at rest on a frictionless horizontal surface. A constant force Fis applied on one of the blocks pulling it away from the other as shown in Fig. ,4E.21(a)
(a) find tyhe position of CM at time t,
(b) if the extension of the spring is x0 at time t, find the displacement of the blocks at that instant.
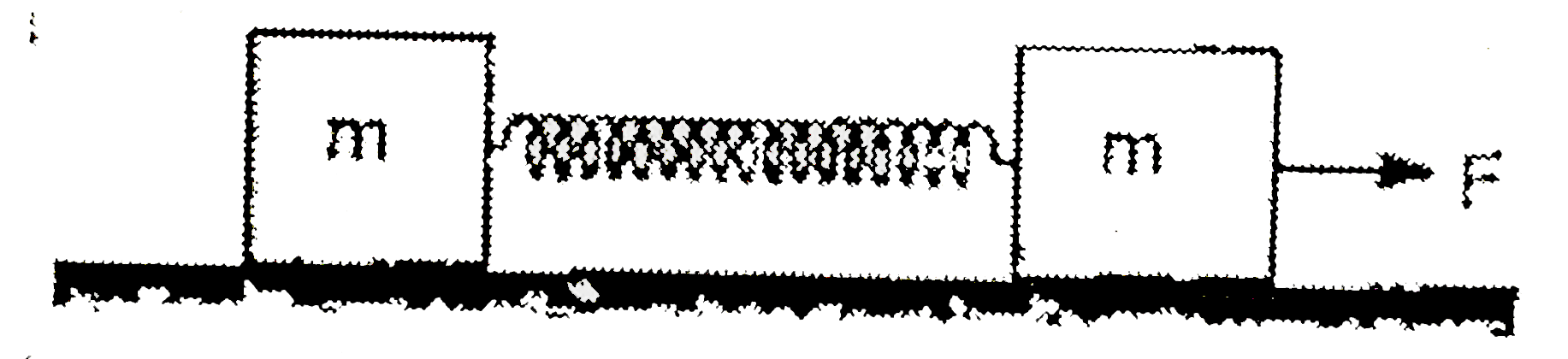
View SolutionTwo blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block pulling away from the other as shown in Fig.

If the extension of the spring is x0 at time t, then the displacement of the first block at this instant is
View Solution
