Two boats of base areas A1 and A2, connected by a string are being pulled by an external force F0. The viscosity is η and depth of the water body is H. when the system attains a constant speed. The tension in the thead will be
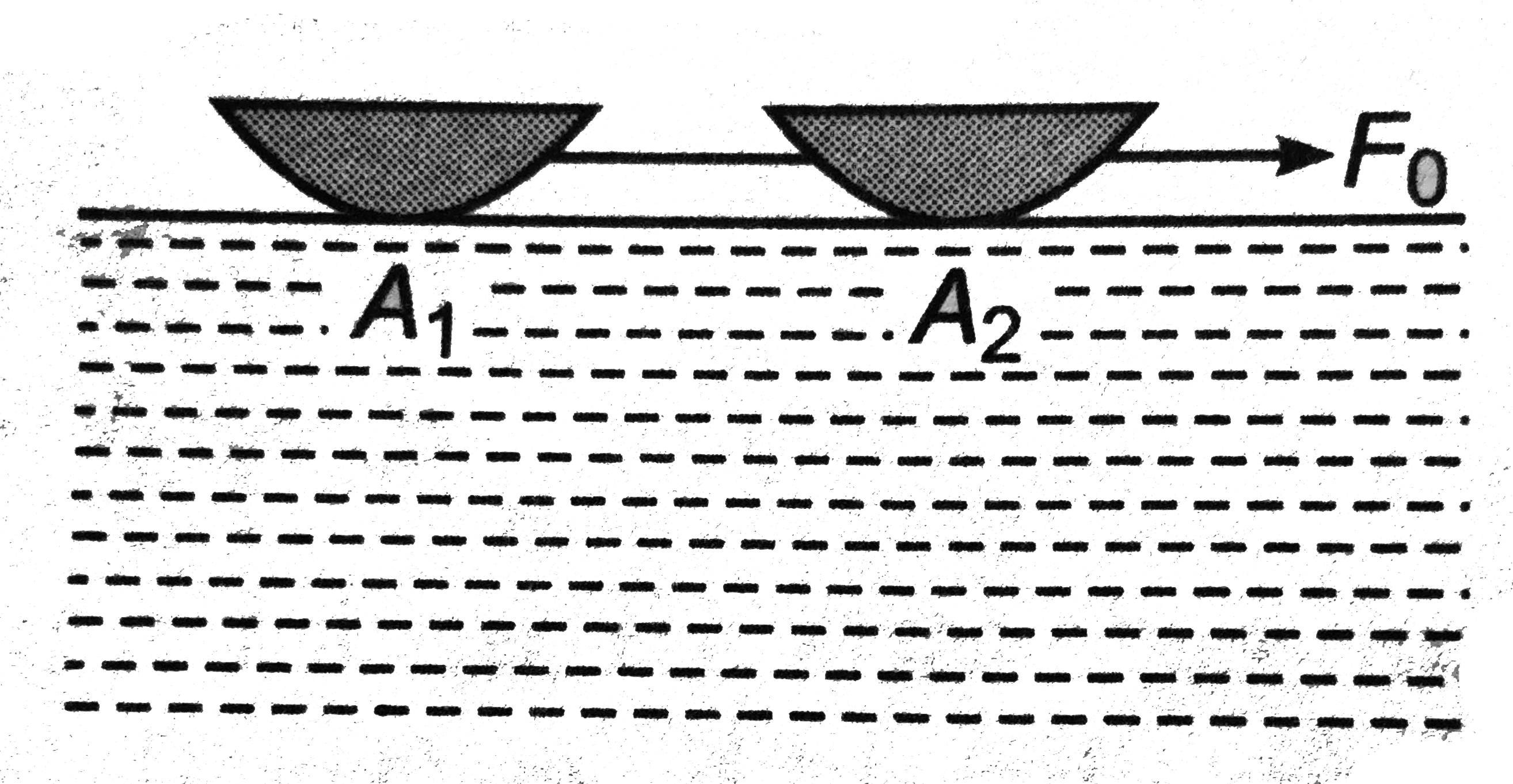
F0(A1A2)
F0A2(A1+A2)
F0A1(A1+A2)
F0(A2A1)
The correct Answer is:C
Viscous force F∝(area)
So let F=kA
F0=k(A1+A2) …(i)
and T=kA1 ….(ii)
Divinding Eq, (ii) by Eq. (i) we get,
TF0=A1A1+A2
∴T=F0A1A1+A2.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 More Than One Correct
10 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Comprehension Based
7 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question
2 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
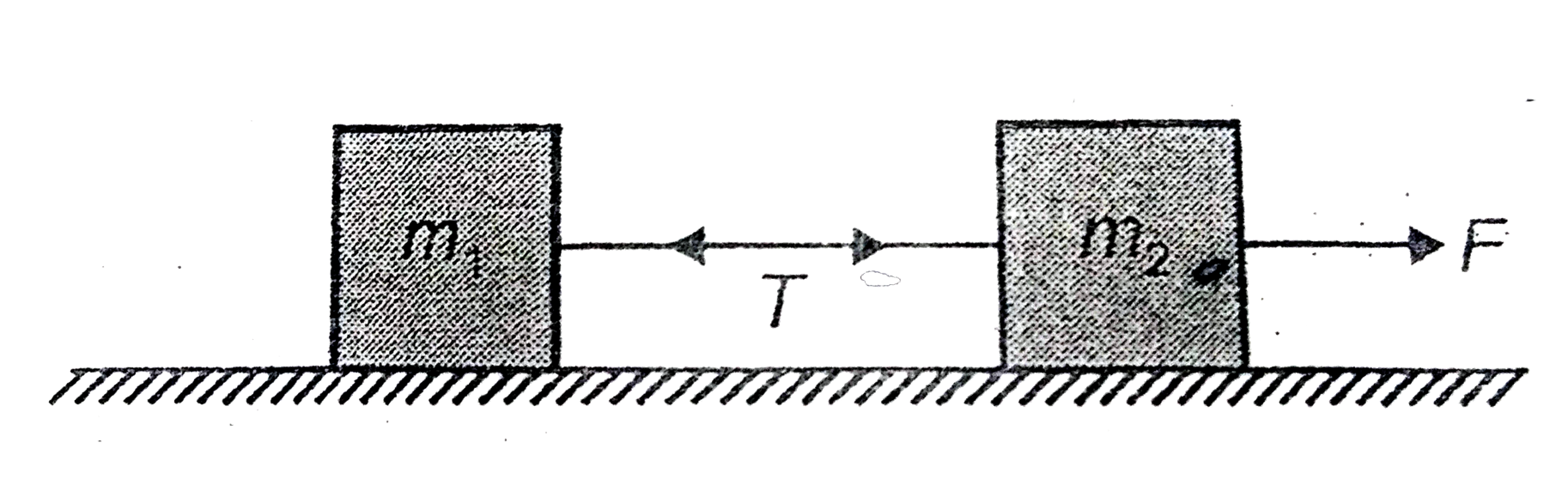
Two block of masses m1andm2 are lying on a frictionless horizontal table connected by a light string m2 is pulled by horizontal force F. Calculate the tension in the string .
View Solution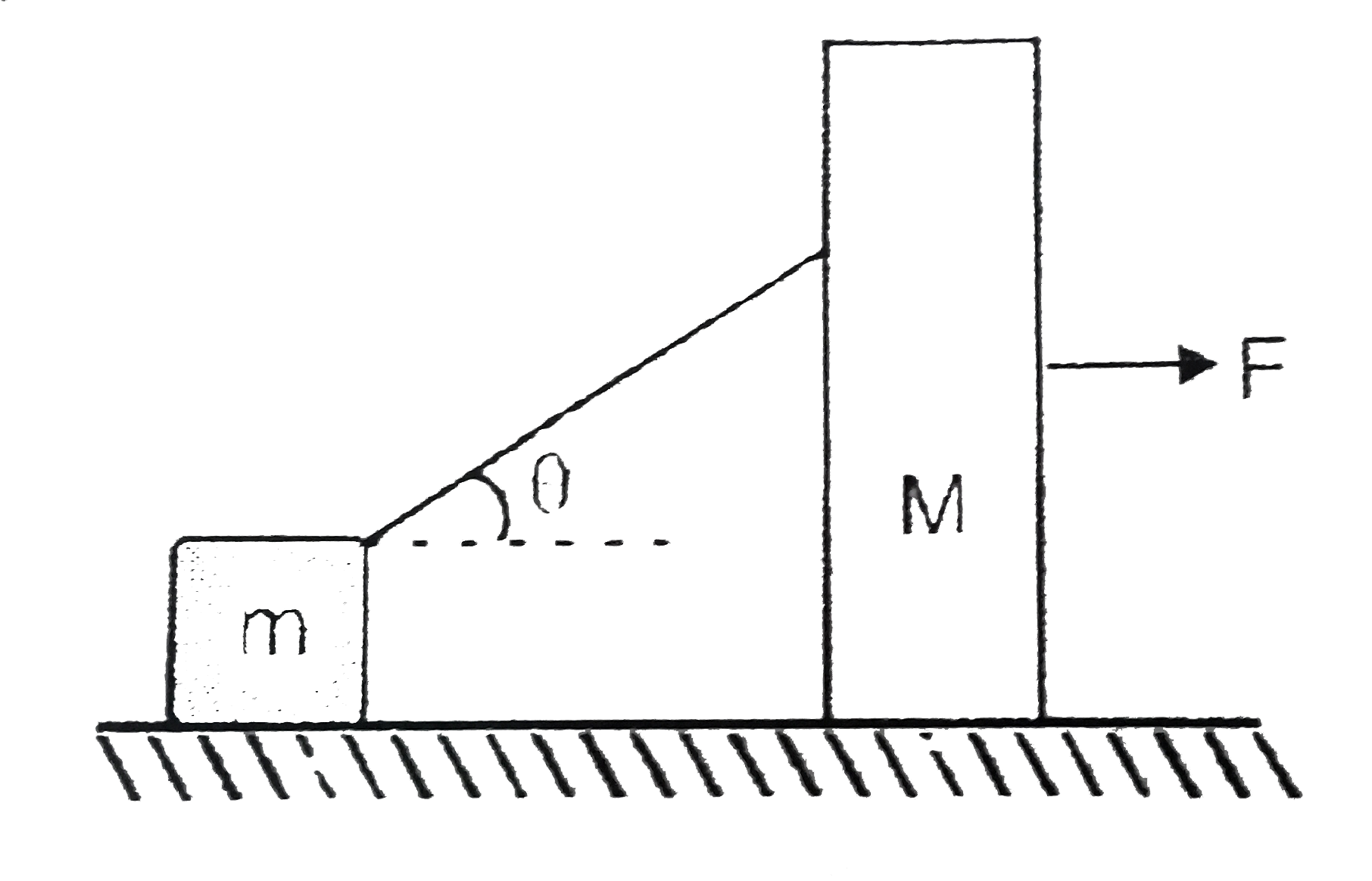
Two blocks of masses m and M are connected by an inextensible light string as shown in the figure. When a constant horizontal force F acts on the block of the mass M, then tension in the string: (Assume that contact between the ground and the two blocks are frictionless) (given m=1kg,M=4kg,F=10N,θ=60∘)
View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionTwo bodies of masses 4 kg and 6 kg connected by means of a light string are lying on a smooth horizontal surface. A horizontal pulling force is applied on the lighter body. Two seconds later the string connecting the two masses is cut. After two more seconds if the velocity of the heavier mass is 2ms−1, the force initially applied is
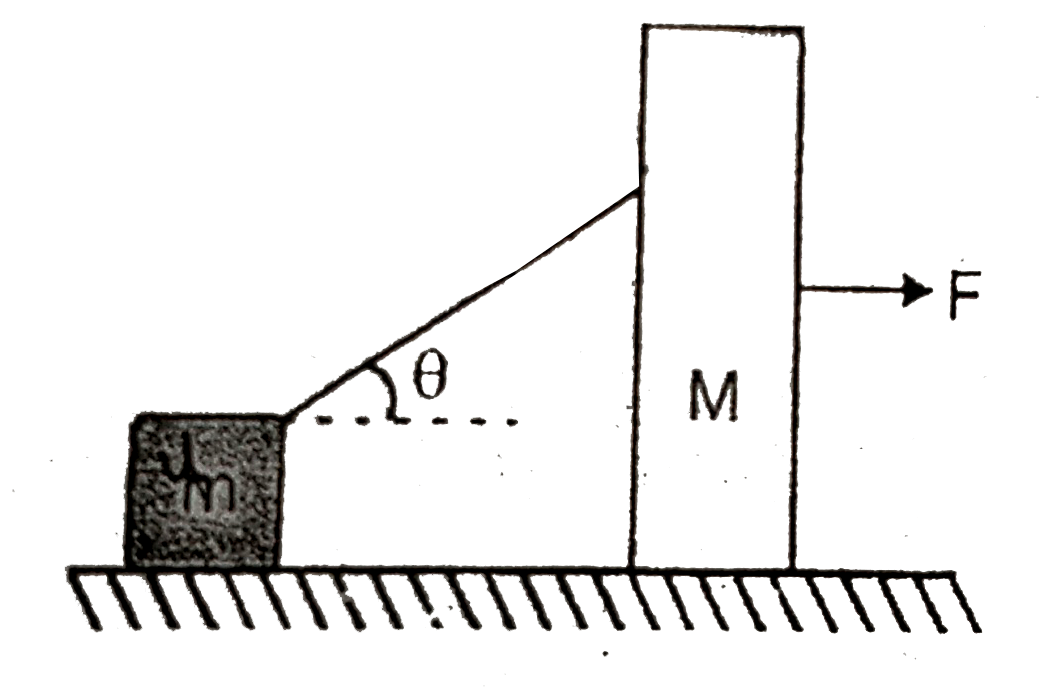
View SolutionTwo blocks of masses m and M are connected by an inextensible light string as shown in the figure. When a constant horizontal force F acts on the block of the mass M, then tension in the string is : (Assume that constact between the ground and the two blocks are frictionless) and blocks will not topple. (Given: m=1kg,M=4kg,F=10N,θ=60∘)
View SolutionTwo point masses m1 and m2 are connected by a spring of natural length l0. The spring is compressed such that the two point masses touch each other and then they are fastened by a string. Then the system is moved with a velocity v0 along positive x-axis. When the system reached the origin, the string breaks (t=0). The position of the point mass m1 is given by x1=v0t−A(1−cosωt) where A and ω are constants. Find the position of the second block as a function of time. Also, find the relation between A and l0.
View SolutionTwo blocks of mass 4kg and 2kg are connected by a heavy string and placed on rough horizontal plane. The 2 kg block is pulled with a constant force F. the coefficent of friction between the blocks and the ground is 0.5. what is the value of F so that tension in the string is constant throughout the length? (g=10m/s2)
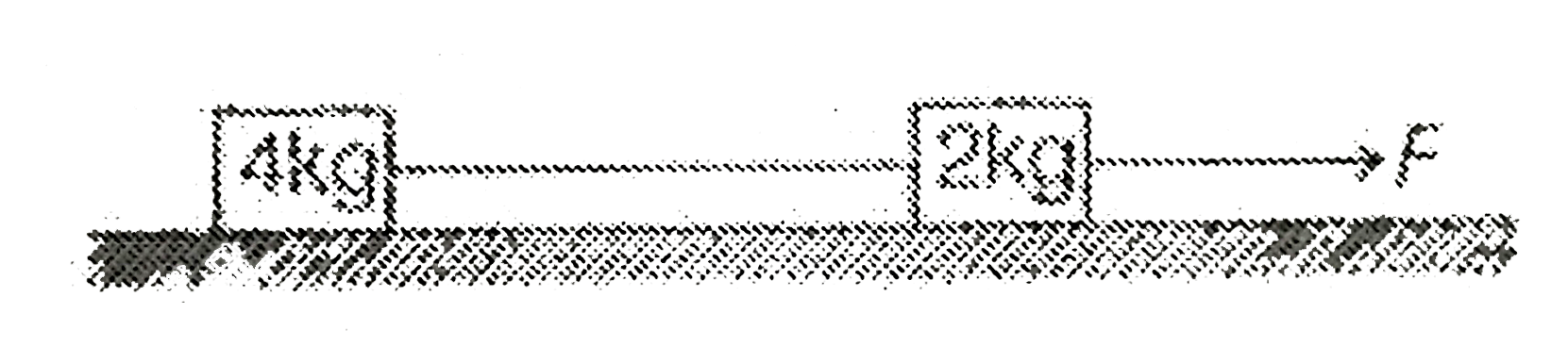
View SolutionA system consists of two cubes of mass m1, and m2 respectively connected by a spring of force constant k. force (F) that should be applied to the upper cube for which the lower one just lifts after the force is removed, is
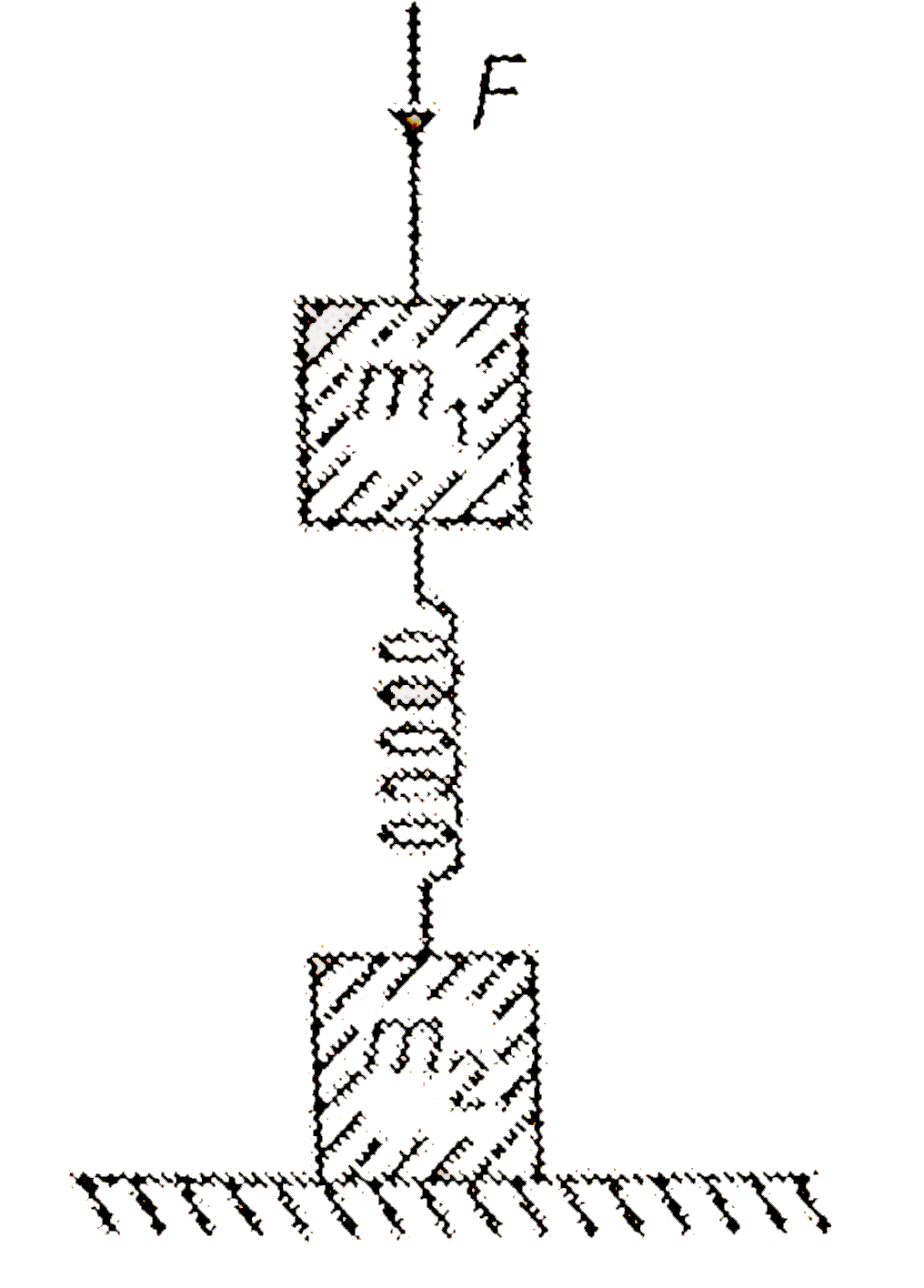
View SolutionTwo bodies A (30 kg) and B (50 kg) tied with a light string are placed on a friction less table. A force F acting at B pulls this system with an acceleration of 2ms−2. The tension in the string is:
View SolutionTwo masses are connected by a string which passes over a pulley acceleration upward at a rate A shown. If a1 and a2 be the accelerations of bodies 1 and 2 respectively then,
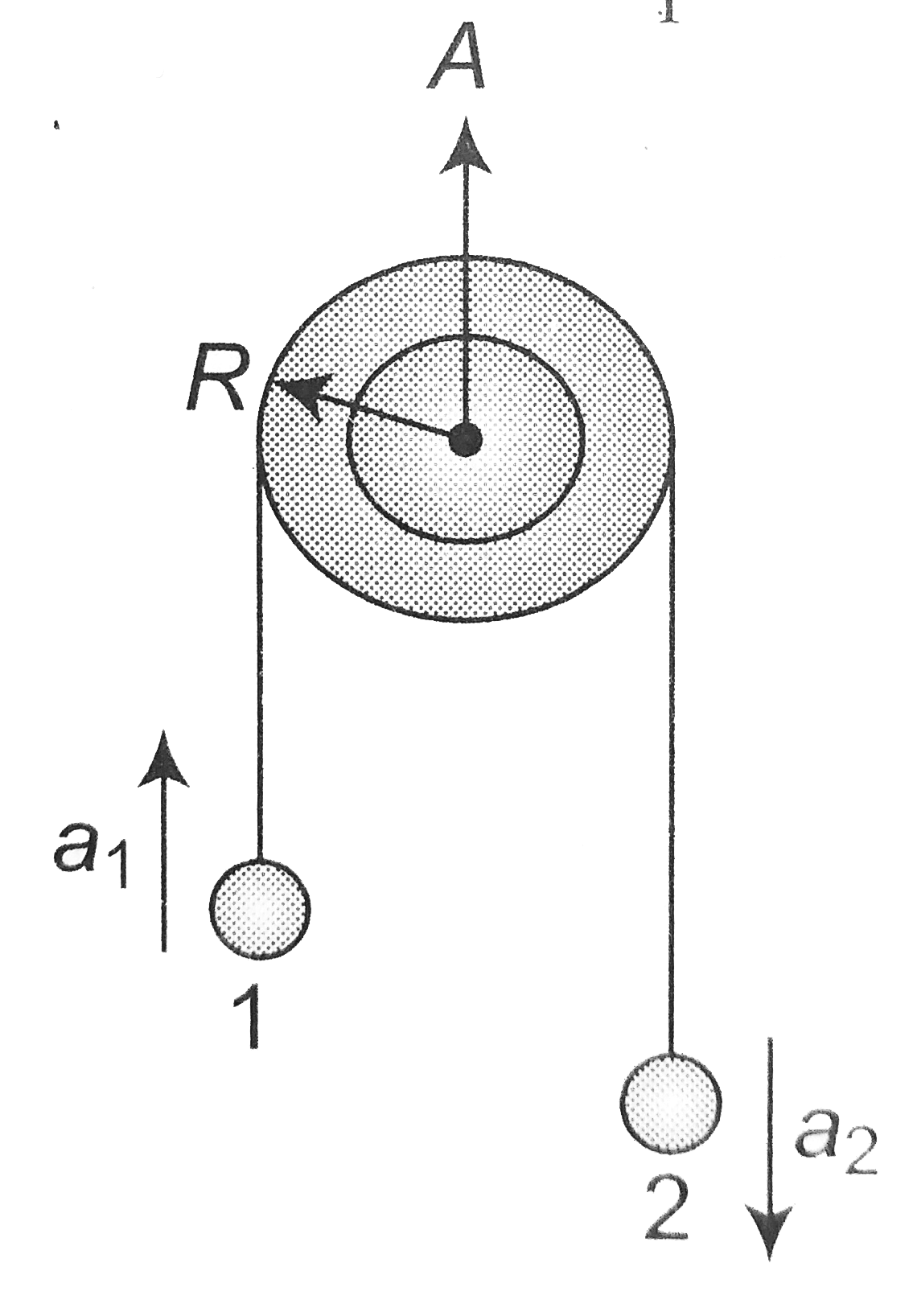
View Solution
