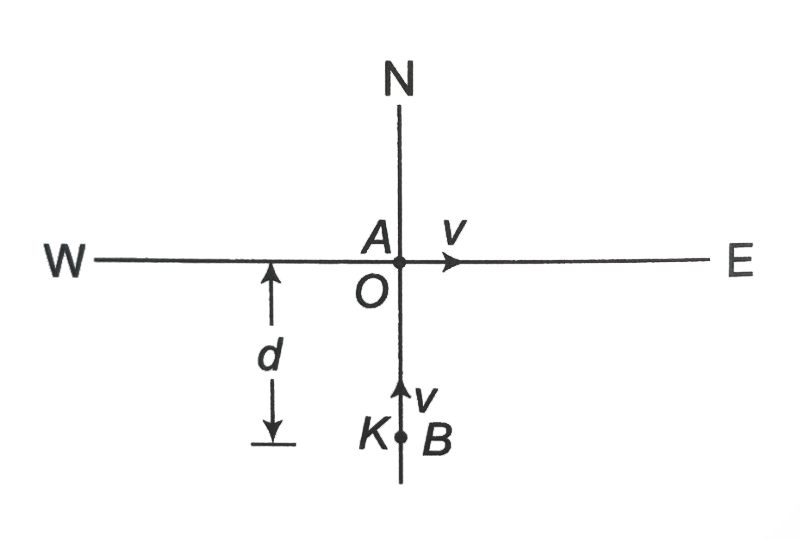
Two cars $A$ and $B$ are running with same speed $v$ . A along east and $B$ towards north. Car $A$ is at origin $O$ and $B$ is at distance $d$ as shown. After how much time, the distance between cars is minimum and what is the minimum distance?

Video Solution

Text Solution

Verified by Experts

First, find the velocity of $B$ w.r.t. $A$

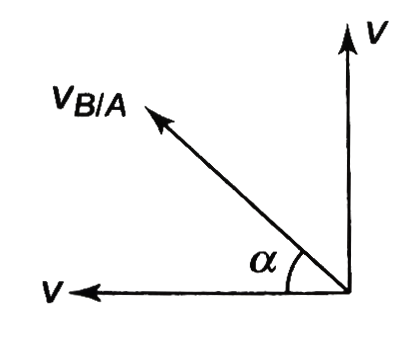
$v_{B / A} = \sqrt{2} v$
$α = 45^{\circ}$
Now, $A$ is at rest and car $B$ is moving on line $K P$ .

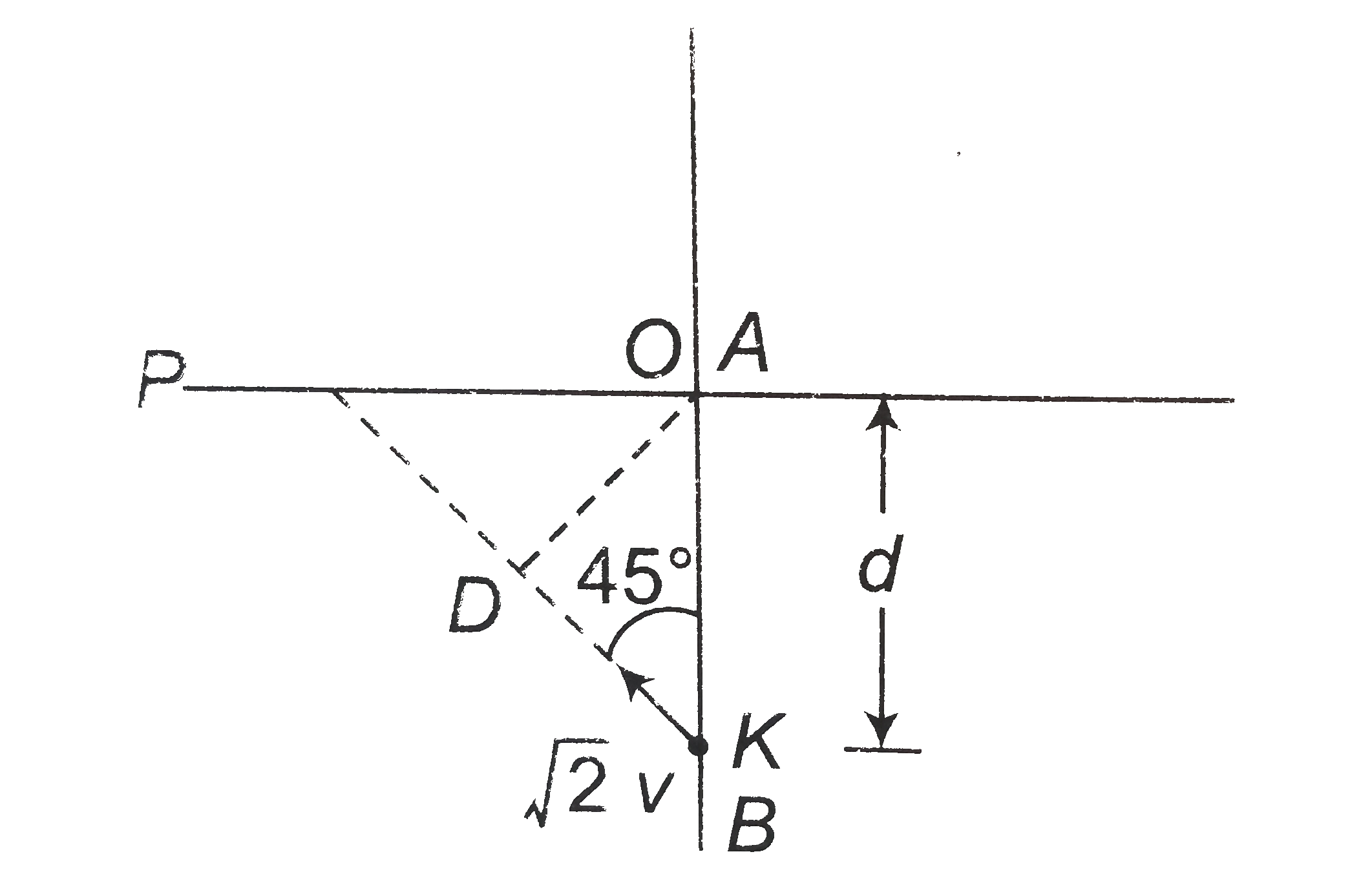
Drop a perpendicular on line $K P$ from $O$ .
$O D$ : minimum distance between cars
$O D = d {sin 45}^{\circ} = \frac{d}{\sqrt{2}}$
The time taken by car $B$ to move from $K$ to $D$
$t = \frac{K D}{\sqrt{2} v} = \frac{d {cos 45}^{\circ}}{\sqrt{2} v} = \frac{d}{2 v}$
Alternatively
At time $t$ , we have

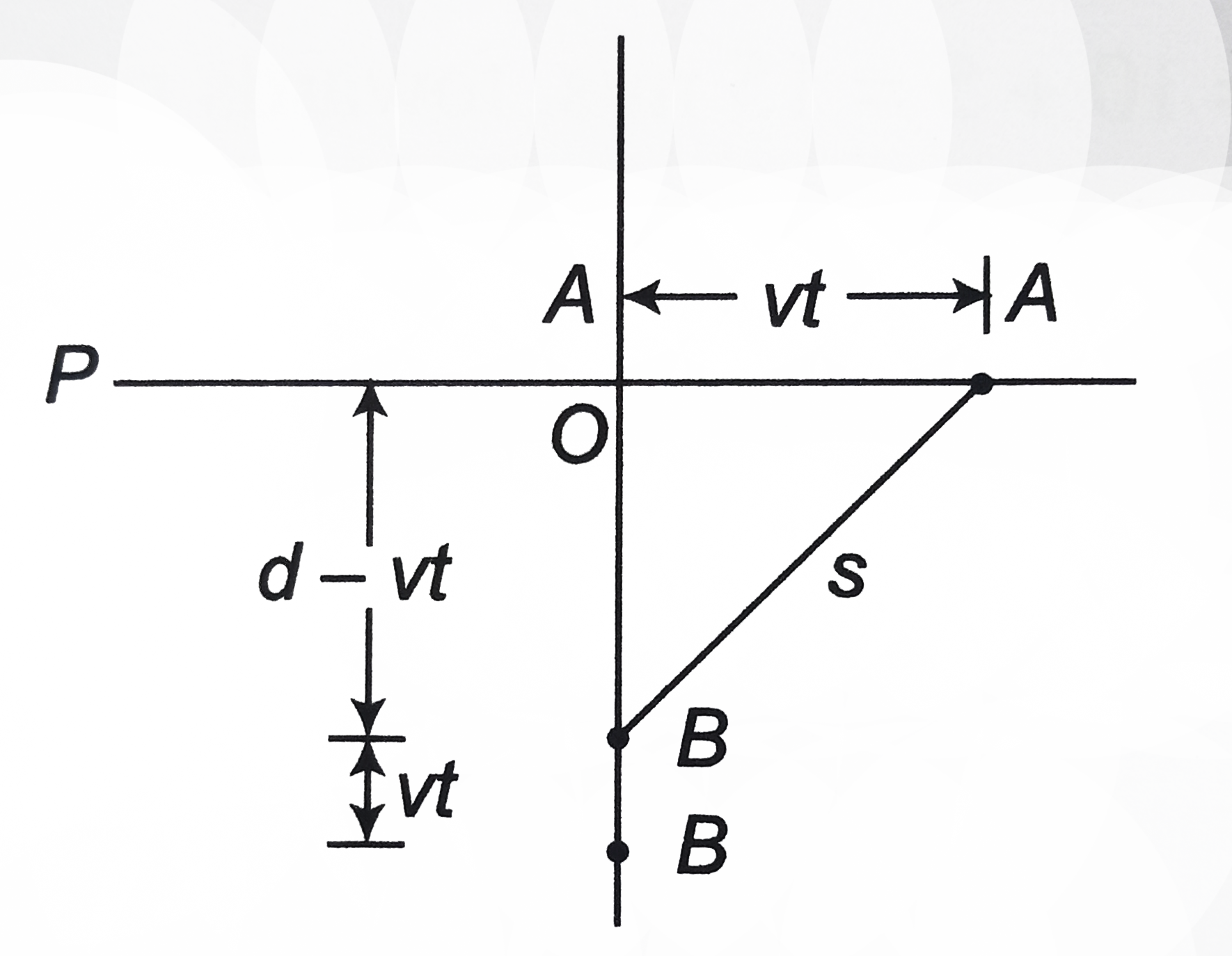
Distance beween the cars
$s^{2} = {(v t)}^{2} + {(d - v t)}^{2}$
For $s$ to be minimum
$\frac{d s}{d t}$ or $\frac{d (s^{2})}{d t} = 2 v t \times v + 2 (d - v t) (- v) = 0$
$v t = d - v t \Rightarrow t = \frac{d}{2 v}$
$s_{min}^{2} = {(v \times \frac{d}{2 v})}^{2} + {(d - v \times \frac{d}{2 v})}^{2}$
$= {(\frac{d}{2})}^{2} + {(\frac{d}{2})}^{2} = \frac{d^{2}}{2}$
$s_{min} = \frac{d}{\sqrt{2}}$

Updated on:21/07/2023

A particle $A$ is at origin and particle $B$ is at distance $y = - d$ at $t = 0 .$ They move with constant velocity $v$ , A towards positive x-axis and $B$ towards origin. The time at which distance between them is minimum and minimum distance will be
A $\frac{d}{2 v}, d$
B $\frac{d}{v}, \frac{d}{\sqrt{2}}$
C $\frac{d}{2 v}, \frac{d}{\sqrt{2}}$
D $\frac{d}{v}, d$
A car is traveling at a speed of 40 kmph and at the same time, another car is also traveling in the same direction at a speed of 50 kmph. Find the distance between both car after 3 hours.
A20 km
B25 km
C30 km
D35 km
A car moving with a speed of 40 km/h can be stopped by applying brakes after at least 2m. If the same car is moving with a speed of 80 km/h., what is the minimum stopping distance ?
A2 m
B4 m
C6 m
D8 m