Two concentric shells have masses M and m and their radii are R and r, respectively, where R>r. What is the gravitational potential at their common centre?
−GMR
−GMr
−G[MR−mr]
−G[MR+mr]
The correct Answer is:D
To find the gravitational potential at the common center of two concentric shells with masses M and m and radii R and r respectively, we can follow these steps:
Step 1: Understanding Gravitational Potential
The gravitational potential V at a distance d from a mass M is given by the formula:
V=−GMd
where G is the gravitational constant.
Step 2: Gravitational Potential Inside a Shell
According to the shell theorem, the gravitational potential inside a uniform spherical shell is constant and equal to the potential at its surface. Therefore, the gravitational potential inside each shell at the center (which is also at a distance less than the radius of the shell) can be calculated using the radius of the shell.
Step 3: Calculate the Potential Due to Each Shell
1. For the larger shell (mass M and radius R):
The gravitational potential at the center due to this shell is:
VM=−GMR
2. For the smaller shell (mass m and radius r):
The gravitational potential at the center due to this shell is:
Vm=−Gmr
Step 4: Total Gravitational Potential at the Center
The total gravitational potential V at the center is the sum of the potentials due to both shells:
V=VM+Vm=−GMR−Gmr
This can be simplified to:
V=−G(MR+mr)
Final Answer
Thus, the gravitational potential at the common center of the two concentric shells is:
V=−G(MR+mr)
---
The gravitational potential V at a distance d from a mass M is given by the formula:
V=−GMd
where G is the gravitational constant.
According to the shell theorem, the gravitational potential inside a uniform spherical shell is constant and equal to the potential at its surface. Therefore, the gravitational potential inside each shell at the center (which is also at a distance less than the radius of the shell) can be calculated using the radius of the shell.
1. For the larger shell (mass M and radius R):
The gravitational potential at the center due to this shell is:
VM=−GMR
The gravitational potential at the center due to this shell is:
Vm=−Gmr
The total gravitational potential V at the center is the sum of the potentials due to both shells:
V=VM+Vm=−GMR−Gmr
This can be simplified to:
V=−G(MR+mr)
Thus, the gravitational potential at the common center of the two concentric shells is:
V=−G(MR+mr)
Topper's Solved these Questions
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Multiple Correct
24 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Assertion- Reasoning
13 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Subjective
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
Similar Questions
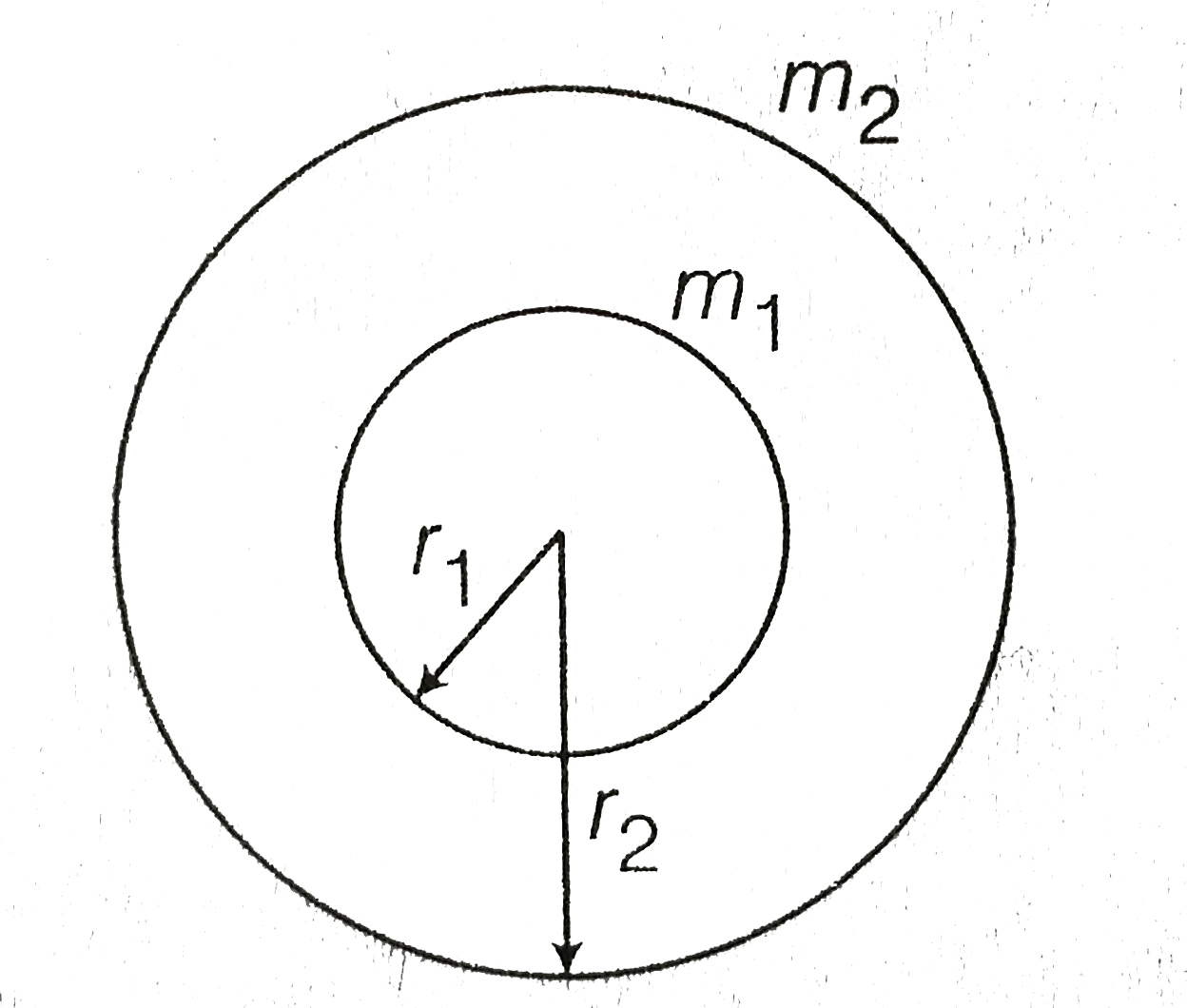
Two concentric spherical shells of masses and radii m1,r1andm2,r2 respectively are as shown. The gravitational field intensity at point P is

View SolutionTwo concentric spherical shells have masses m1andm2 and radii r1andr2(r2>r1). What is the force exerted by this system on a particle of mass m3. If it is placed at a distance r(r1<r<r2) from the centre ?
View Solution
Knowledge Check
Two concentric spherical shells A and B of radii R and 2R and mases 4M, and M, respectively are placed in space as shown in Fig. The gravitational potential at P at a distance r(R<r<2R)from the centre of shells is
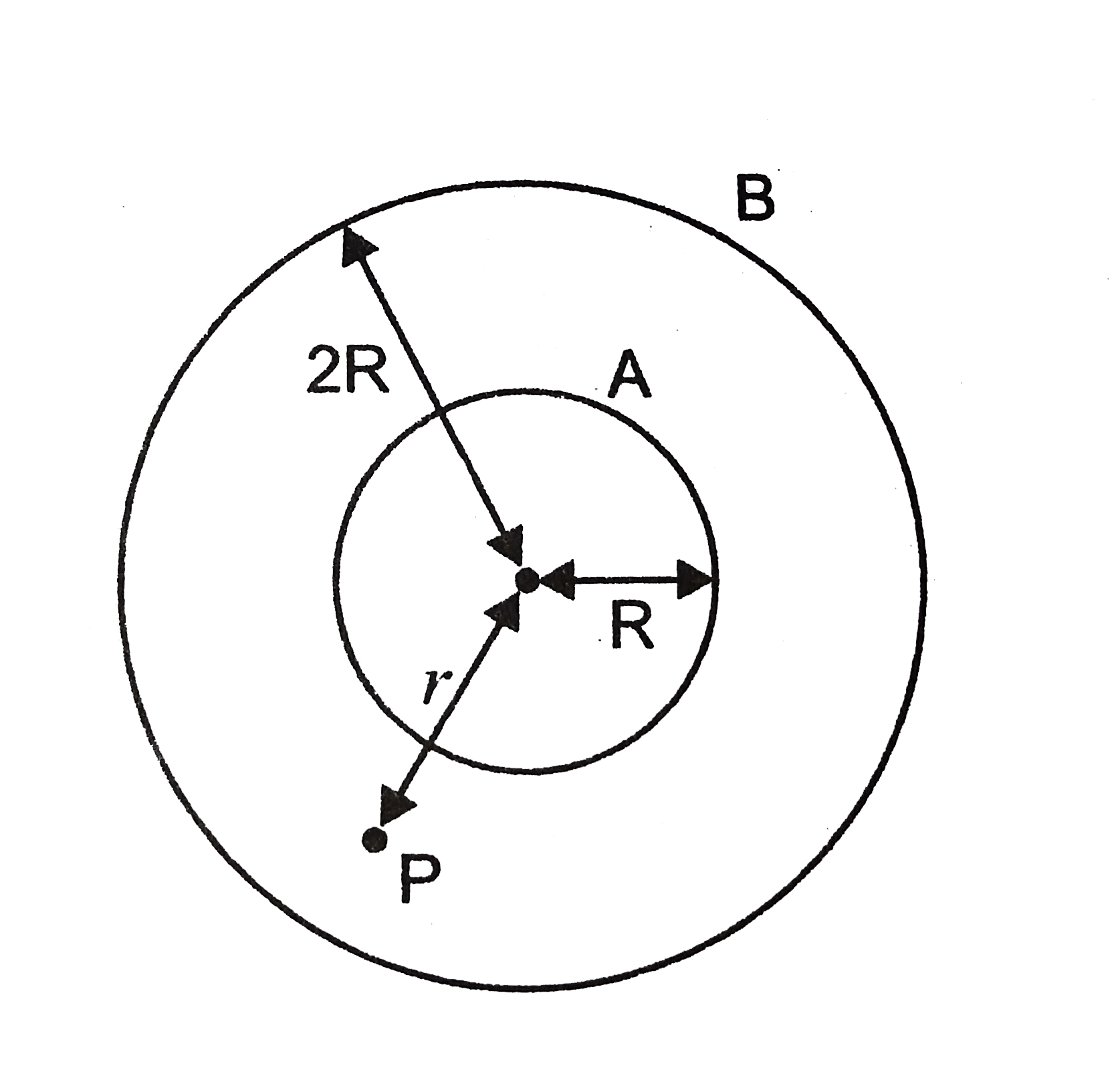 A−4GMRB−9GM2RC−4GM3RDnone of these
A−4GMRB−9GM2RC−4GM3RDnone of theseTwo concentric spheres kept in air have radii R and r. They have similar charge and equal surface charge density σ. The electrical potential at their common centre is (where, ε0= permittivity of free space)
Aσ(R+r)ε0Bσ(R−r)ε0Cσ(R+r)2ε0Dσ(R+r)4ε0Assertion : Two spherical shells have masses m1andm2. Their radii are r1andr2. Let r be the distance of a point from centre. Then gravitational field strength and gravitational potential both are equal to zero for O<r<r1
Reason : In the region r1<r<r2, gravitational field strength due to m2 is zero. But gravitational potential due to m2 is constant (but non-zero).AIf both Assertin and Reason are correct and Reason is the correct explanation of AssertionBIf both Assertion and Reason are correct but Reason is not the correct explanation of AssertionCIf Assertion is true but Reason is falseDIf Assertion is false but Reason is true
A charge Q is distributed over the surfaces of two concentric hollow spheres of radii r and R(R>>r) , such that their surface charge densities are equal . Derive the expression for the potential at the common centre.
View Solution_E01_035_Q01.png)
There is a concentric hole of radius R in a solid sphere of radius 2R. Mass of the remaining portional is M. What is the gravitational potential at centre?
View SolutionTwo concentric spheres of radii R and r have similar charges with equal surface densities (σ) What is the electric potential at their common centre /
View SolutionTwo concentric spheres of radii R and r have similar charges with equal surface densities (σ) What is the electric potential at their common centre /
View SolutionTwo concentric shells of masses M1 and M2 are having radii r1 and r2. Which of the following is the correct expression for the gravitational field on a mass m?

View Solution
